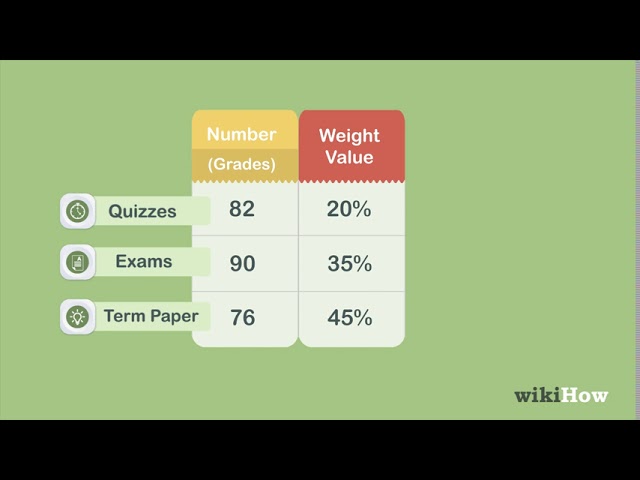
The weighted average coupon (WAC) is a calculation used in finance to determine the average coupon rate of a portfolio of fixed-income securities. It is calculated by multiplying the coupon rate of each security by its weight in the portfolio and then summing the results.
The WAC is an important measure because it provides a single number that represents the average interest rate that the portfolio will earn. This information can be used to compare different portfolios and to make decisions about how to allocate investments. Historically, the WAC was calculated manually, but today it can be calculated quickly and easily using a financial calculator or spreadsheet software.
This article will provide a step-by-step guide on how to calculate the weighted average coupon. We will also discuss the importance of the WAC, its benefits, and its historical development.
How to Calculate Weighted Average Coupon
Weighted average coupon (WAC) is a crucial metric in fixed income analysis. Understanding its calculation involves several essential aspects:
- Coupon Rate
- Maturity Date
- Face Value
- Weighting
- Portfolio Size
- Calculation Method
- Applications
- Limitations
Coupon rate and maturity date determine the cash flows, while face value and weighting reflect the relative importance of each security in the portfolio. The calculation method involves multiplying the coupon rate by the weighting and summing the results. WAC is used to compare portfolios, assess risk, and make investment decisions. However, it assumes equal cash flow intervals and may not consider other factors like call provisions.
Coupon Rate
Coupon rate is an essential component of how to calculate weighted average coupon (WAC). It represents the annual interest payment made on a fixed-income security, typically expressed as a percentage of the face value. Understanding coupon rate is crucial for accurately calculating WAC and evaluating fixed-income investments.
- Nominal Coupon Rate
The stated interest rate on a bond, as specified in the bond indenture. It is used to calculate the periodic interest payments.
- Current Coupon Rate
The annualized interest rate based on the current market price of a bond. It reflects the actual yield to maturity.
- Zero Coupon Rate
A bond that pays no periodic interest payments, but instead is sold at a discount and redeemed at face value at maturity.
- Stepped-Up Coupon Rate
A bond that has a coupon rate that increases over time, providing higher interest payments in later years.
Coupon rate significantly impacts WAC, as higher coupon rates lead to a higher WAC. It is a key factor in determining the overall return and risk profile of a fixed-income portfolio.
Maturity Date
Maturity date plays a crucial role in determining the weighted average coupon (WAC) of a fixed-income portfolio. It represents the date on which the principal amount of a bond or other debt security becomes due and payable to the investor.
- Actual Maturity Date
The specific calendar date on which a bond matures, as stated in the bond indenture.
- Weighted Average Maturity
A calculation that considers the maturity dates and relative weights of all bonds in a portfolio to determine an overall average maturity.
- Extendable Maturity
A feature that allows bond issuers to extend the maturity date of a bond beyond its original term, often subject to certain conditions.
- Callable Maturity
A bond that gives the issuer the option to redeem the bond before its maturity date, often at a specified call price.
Maturity date influences WAC by determining the duration of the cash flows used in the calculation. Longer maturities typically result in higher WAC due to the time value of money and interest rate risk. Callable and extendable bonds introduce additional complexity, as they can alter the effective maturity and impact WAC.
Face Value
Face value, also known as par value or nominal value, is a critical component in calculating the weighted average coupon (WAC) of a fixed-income portfolio. It represents the principal amount of a bond or other debt security, which is typically repaid to the investor at maturity. Face value directly impacts WAC because it determines the base value upon which interest payments are calculated.
To illustrate, consider a bond with a face value of $1,000 and a coupon rate of 5%. The annual coupon payment for this bond is $50 (5% x $1,000). If this bond is part of a portfolio, its weight in the calculation of WAC is determined by its face value relative to the total face value of all bonds in the portfolio. A bond with a higher face value will have a greater weight, resulting in a higher contribution to the overall WAC.
Understanding the relationship between face value and WAC is essential for accurate portfolio analysis and decision-making. By considering the face value of each bond, investors can assess the overall yield and risk profile of their fixed-income investments. WAC provides a comprehensive measure of the average interest rate earned by the portfolio, taking into account the face values and coupon rates of individual bonds. This information is crucial for comparing different portfolios, evaluating investment strategies, and making informed choices about fixed-income investments.
Weighting
Weighting plays a crucial role in the calculation of the weighted average coupon (WAC) of a fixed-income portfolio. It represents the relative importance or proportion of each bond within the portfolio, influencing the overall average coupon rate.
- Face Value Weighting
The weight of a bond based on its face value or principal amount. Bonds with higher face values have a greater impact on the WAC.
- Market Value Weighting
The weight of a bond based on its current market value. This method considers the price fluctuations of bonds in the portfolio.
- Coupon Rate Weighting
The weight of a bond based on its coupon rate. Bonds with higher coupon rates contribute more to the overall WAC.
- Maturity Weighting
The weight of a bond based on its maturity date. Bonds with longer maturities have a greater impact on WAC due to the time value of money.
Weighting allows investors to customize the WAC calculation based on their investment objectives and risk tolerance. By assigning different weights to bonds, they can emphasize specific characteristics, such as high coupon rates or short maturities, to achieve a desired portfolio outcome. Understanding the concept and implications of weighting is essential for accurate WAC calculations and effective fixed-income portfolio management.
Portfolio Size
Portfolio size plays a crucial role in calculating the weighted average coupon (WAC) as it determines the number and composition of bonds within a portfolio. A portfolio’s size can vary significantly, affecting the calculation and interpretation of WAC.
- Number of Bonds
The number of bonds in a portfolio directly influences the calculation of WAC. A portfolio with a larger number of bonds will have a more diversified WAC, as it incorporates a broader range of coupon rates and maturities.
- Face Value
The total face value of bonds in a portfolio affects the weighting of each bond in the WAC calculation. Bonds with higher face values have a greater impact on the overall WAC.
- Asset Allocation
The asset allocation of a portfolio, such as the proportion of bonds to other asset classes, can influence the WAC. A portfolio with a higher allocation to bonds will typically have a higher WAC.
- Investment Strategy
The investment strategy employed by a portfolio manager can impact the portfolio’s size and composition, which in turn affects the WAC. For instance, a strategy focused on high-yield bonds will likely result in a higher WAC.
In summary, portfolio size is a multifaceted aspect that influences the weighted average coupon calculation. It encompasses the number of bonds, face value, asset allocation, and investment strategy, each contributing to the overall WAC. Understanding the relationship between portfolio size and WAC is essential for accurate portfolio analysis and informed investment decision-making.
Calculation Method
The calculation method is a core aspect of weighted average coupon (WAC) determination. It involves precise steps and considerations to accurately reflect the portfolio’s characteristics and risk-return profile.
- Bond Identification
Identifying the specific bonds included in the portfolio is crucial. Each bond’s coupon rate, maturity date, and face value contribute to the overall WAC.
- Weighting Assignment
Assigning weights to each bond based on their face value or market value ensures their proportional impact on the WAC calculation. This reflects the relative significance of each bond within the portfolio.
- Coupon Rate Adjustment
The calculation method should consider any adjustments to coupon rates. This includes accounting for stepped-up or stepped-down coupon payments, which can affect the average coupon rate over the bond’s life.
- Maturity Consideration
The method should incorporate the maturity dates of the bonds. Bonds with longer maturities typically have higher WAC due to the time value of money and interest rate risk.
Understanding the calculation method empowers investors to accurately assess the WAC of their fixed-income portfolios. It provides insights into the portfolio’s overall risk and return characteristics, enabling informed investment decisions.
Applications
The applications of the weighted average coupon (WAC) calculation extend beyond theoretical understanding. It serves as a critical tool for various financial professionals and investors seeking to evaluate and manage fixed-income portfolios.
Firstly, WAC plays a crucial role in assessing the overall risk and return profile of a bond portfolio. By considering both the coupon rates and weights of individual bonds, investors can gauge the portfolio’s sensitivity to interest rate fluctuations and make informed decisions regarding its risk-return trade-offs.
Furthermore, WAC is essential for comparing different fixed-income investment opportunities. By calculating the WAC of various portfolios or bonds, investors can identify those that best align with their investment objectives and risk tolerance. This comparative analysis enables them to optimize their portfolio diversification and maximize potential returns.
In practical terms, WAC finds applications in portfolio management, performance measurement, and risk analysis. Fund managers utilize WAC to monitor and adjust their portfolios’ risk-return profiles, ensuring they meet the desired investment goals. Additionally, WAC serves as a benchmark against which portfolio performance can be evaluated, allowing investors to assess the effectiveness of their investment strategies.
In summary, the calculation of weighted average coupon is a fundamental aspect of fixed-income investing, providing essential insights into portfolio risk and return characteristics. Its practical applications empower investors and financial professionals to make informed decisions, optimize portfolio diversification, and achieve their investment objectives.
Limitations
The calculation of weighted average coupon (WAC) is a valuable tool, but it has certain limitations that should be considered for accurate interpretation and informed decision-making.
- Data Accuracy
The accuracy of WAC depends on the accuracy of the input data, such as coupon rates, maturity dates, and weights. Errors or inconsistencies in the data can lead to incorrect WAC calculations and, consequently, flawed investment decisions.
- Assumptions
The WAC calculation assumes that the cash flows will be received as scheduled and that the coupon rate remains constant over the life of the bond. However, in reality, prepayments, defaults, and changes in interest rates can affect the actual cash flows and, therefore, the realized WAC.
- Market Conditions
The WAC does not fully capture the impact of market conditions, such as changes in interest rates and credit spreads. These factors can significantly affect the value of the underlying bonds and, consequently, the overall return of the portfolio.
- Complexity
Calculating WAC can be complex, especially for portfolios with a large number of bonds or complex structures. This complexity can introduce errors or inaccuracies if not handled properly.
Despite these limitations, WAC remains a useful metric for evaluating fixed-income portfolios. By understanding its limitations and using it in conjunction with other analysis techniques, investors can gain valuable insights into the risk and return characteristics of their investments.
FAQs on Calculating Weighted Average Coupon
This section addresses frequently asked questions (FAQs) related to the calculation of weighted average coupon (WAC). These FAQs aim to clarify common concerns, misconceptions, and technical aspects of WAC calculations.
Question 1: What is the purpose of calculating WAC?
Answer: WAC provides a single metric that represents the average interest rate earned by a fixed-income portfolio. It helps investors assess the overall risk and return profile of their portfolio.
Question 2: How do I calculate WAC for a bond portfolio?
Answer: Multiply the coupon rate of each bond by its weight in the portfolio and sum the results. The weights can be based on face value, market value, or other criteria.
Question 3: What factors affect the WAC of a portfolio?
Answer: Coupon rates, maturity dates, and the weights of individual bonds all influence the WAC. Changes in interest rates and credit spreads can also impact the WAC.
Question 4: How does WAC help in comparing fixed-income investments?
Answer: By calculating the WAC of different portfolios or bonds, investors can identify those that offer the most favorable combination of risk and return.
Question 5: Are there any limitations to using WAC?
Answer: WAC assumes constant cash flows and coupon rates, which may not always be the case. It also does not fully capture the impact of market conditions.
Question 6: How can I use WAC in portfolio management?
Answer: WAC can help portfolio managers assess the risk-return profile of their portfolios and make informed decisions about asset allocation and bond selection.
These FAQs provide a foundation for understanding the calculation and applications of weighted average coupon. In the next section, we will explore advanced concepts related to WAC, including its role in fixed-income risk management and performance evaluation.
Tips for Calculating Weighted Average Coupon
Calculating weighted average coupon (WAC) accurately is crucial for effective fixed-income portfolio management. Here are some practical tips to ensure precise and reliable WAC calculations:
Tip 1: Verify Data Accuracy
Ensure the coupon rates, maturity dates, and weights used in the calculation are accurate and up-to-date. Errors in input data can lead to incorrect WAC results.
Tip 2: Consider Stepped Coupons
If bonds have stepped-up or stepped-down coupon payments, adjust the coupon rate used in the calculation to reflect the average rate over the life of the bond.
Tip 3: Use Appropriate Weighting Method
Choose a weighting method that aligns with your investment objectives. Face value weighting is common, but market value or coupon rate weighting may be more suitable in certain scenarios.
Tip 4: Account for Prepayments and Defaults
WAC assumes timely receipt of cash flows. Consider potential prepayments or defaults and adjust the calculation accordingly.
Tip 5: Monitor Market Conditions
Interest rate changes and credit spreads can impact the value of bonds and, consequently, the WAC. Monitor market conditions and adjust your calculations as needed.
Tip 6: Use Technology for Complex Portfolios
For portfolios with a large number of bonds or complex structures, consider using financial calculators or spreadsheet software to automate WAC calculations.
By following these tips, you can enhance the accuracy and reliability of your WAC calculations, leading to more informed investment decisions.
In the next section, we will explore advanced concepts and applications of WAC, including its role in fixed-income risk management and performance evaluation.
Conclusion
In summary, calculating weighted average coupon (WAC) provides a comprehensive measure of the average interest rate earned by a fixed-income portfolio. Its formula considers both the coupon rates and weights of individual bonds, enabling investors to assess the overall risk and return profile of their investments.
Key points to remember:
- WAC helps investors compare different fixed-income opportunities and make informed decisions about portfolio diversification.
- The accuracy of WAC calculations depends on the reliability of input data and the appropriate consideration of factors such as stepped coupons and market conditions.
- Advanced applications of WAC include its use in fixed-income risk management and performance evaluation, providing valuable insights for portfolio managers.
As the fixed-income landscape continues to evolve, a thorough understanding of WAC remains essential for investors seeking to navigate market complexities and optimize their investment strategies.