Calculating zero-coupon bonds in Excel is a critical skill for financial professionals. Zero-coupon bonds are bonds that do not pay periodic interest payments, instead paying all interest (in the form of a lump sum) at maturity. This makes them attractive to investors who are looking for a fixed rate of return over a specific period of time, and to issuers who are looking to raise capital without having to pay periodic interest payments.
Zero-coupon bonds are typically purchased at a discount to their face value, and the difference between the purchase price and the face value represents the return to the investor. The yield-to-maturity (YTM) of a zero-coupon bond is the annualized rate of return that the investor will receive if they hold the bond until maturity.
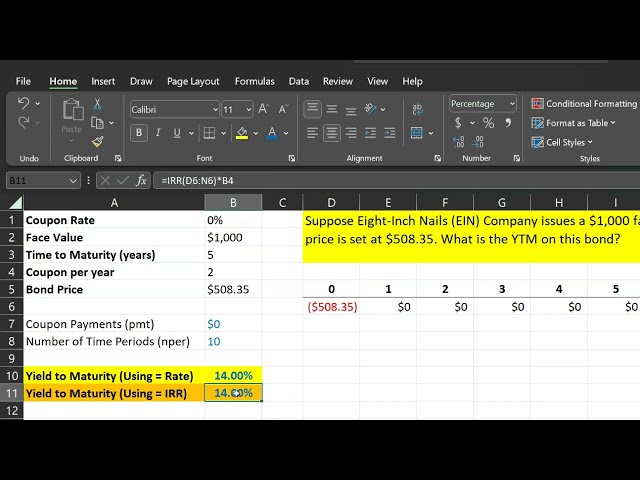
Calculating the YTM of a zero-coupon bond in Excel is a relatively straightforward process. The following steps outline how to do it:
How to Calculate Zero Coupon Bonds in Excel
Calculating zero-coupon bonds in Excel is a valuable skill for financial professionals. Zero-coupon bonds, also known as deep discount bonds, are fixed-income securities that do not pay periodic interest payments. Instead, they are sold at a deep discount to their face value and pay all interest in a single lump sum at maturity.
- Face value: The amount the bondholder will receive at maturity.
- Purchase price: The price at which the bond is purchased.
- Time to maturity: The length of time until the bond matures.
- Yield-to-maturity (YTM): The annualized rate of return an investor will receive if they hold the bond until maturity.
- Present value: The current value of the bond’s future cash flows, discounted at the YTM.
- Accrued interest: The interest that has accrued since the last interest payment date.
- Yield curve: A graph that plots the relationship between interest rates and time to maturity for bonds with similar credit risk.
- Callable bond: A bond that the issuer has the option to redeem before maturity.
These aspects are essential for understanding how to calculate zero-coupon bonds in Excel. By understanding these concepts, investors can make informed decisions about whether to invest in zero-coupon bonds and how to value them. For example, the YTM is a key metric for comparing the returns of different zero-coupon bonds. The present value can be used to determine whether a zero-coupon bond is trading at a fair price. And the yield curve can be used to forecast future interest rates and make investment decisions.
Face Value
The face value of a zero-coupon bond is the amount that the bondholder will receive at maturity. It is also known as the principal or par value. The face value is an important factor to consider when calculating the yield-to-maturity (YTM) of a zero-coupon bond. The YTM is the annualized rate of return that an investor will receive if they hold the bond until maturity.
- Bond Certificate: The face value is typically printed on the bond certificate. It is the amount that the issuer promises to pay the bondholder at maturity.
- Maturity Date: The face value is paid to the bondholder on the maturity date. The maturity date is the date on which the bond matures and the issuer is obligated to repay the principal.
- Interest Payments: Zero-coupon bonds do not pay periodic interest payments. Instead, the return to the investor is the difference between the purchase price and the face value.
- Credit Risk: The face value of a zero-coupon bond is subject to credit risk. If the issuer defaults on the bond, the bondholder may not receive the full face value.
The face value of a zero-coupon bond is an important factor to consider when making investment decisions. Investors should carefully consider the issuer’s credit risk and the maturity date of the bond before investing. The YTM of a zero-coupon bond can be used to compare the returns of different bonds and to make investment decisions.
Purchase price
The purchase price of a zero-coupon bond is the price at which the bond is purchased in the secondary market. It is also known as the dirty price or the market price. The purchase price is an important factor to consider when calculating the yield-to-maturity (YTM) of a zero-coupon bond. The YTM is the annualized rate of return that an investor will receive if they hold the bond until maturity.
The relationship between the purchase price of a zero-coupon bond is that the higher the purchase price, the lower the YTM. This is because the purchase price represents the present value of the bond’s future cash flows, discounted at the YTM. Therefore, if the purchase price is higher, the YTM must be lower in order for the present value of the cash flows to be equal to the purchase price.
A real-life example of the relationship between the purchase price and YTM of a zero-coupon bond can be seen in the following table:
| Purchase Price | YTM |
|---|---|
| $800 | 5% |
| $900 | 4% |
| $1,000 | 3% |
As you can see from the table, as the purchase price of the bond increases, the YTM decreases.
Understanding the relationship between the purchase price and YTM of a zero-coupon bond is important for investors. This understanding can be used to make informed investment decisions about zero-coupon bonds.
Time to maturity
Time to maturity is a critical component of how to calculate zero coupon bonds in Excel. This is because the time to maturity determines the present value of the bond’s future cash flows, which is used to calculate the bond’s yield-to-maturity (YTM). The YTM is the annualized rate of return that an investor will receive if they hold the bond until maturity.
The relationship between time to maturity and the YTM of a zero-coupon bond is inverse. This means that as the time to maturity increases, the YTM decreases. This is because the longer an investor has to wait for the bond to mature, the lower the annualized rate of return they will receive.
For example, consider a zero-coupon bond with a face value of $1,000 and a time to maturity of 5 years. If the current market rate of interest is 5%, the YTM of the bond will be 5%. However, if the time to maturity of the bond is increased to 10 years, the YTM will decrease to 4.5%. This is because the investor will have to wait longer to receive the bond’s face value.
Understanding the relationship between time to maturity and the YTM of a zero-coupon bond is important for investors. This understanding can be used to make informed investment decisions about zero-coupon bonds.
Yield-to-maturity (YTM)
When calculating zero-coupon bonds in Excel, yield-to-maturity (YTM) is a crucial factor. It represents the annualized rate of return an investor can expect to earn if they hold the bond until its maturity date. Understanding YTM is essential for evaluating the attractiveness of zero-coupon bonds and making informed investment decisions.
- Formula: YTM is calculated using the following formula: YTM = (FV / PV)^(1/n) – 1, where FV is the face value of the bond, PV is the present value of the bond, and n is the number of years to maturity.
- Relationship to Price: YTM has an inverse relationship with the bond’s price. As the price of the bond increases, the YTM decreases, and vice versa.
- Impact of Time to Maturity: YTM is also affected by the time to maturity. Generally, longer maturity bonds have higher YTMs compared to shorter maturity bonds, assuming similar credit risk.
- Importance for Investors: YTM is a key metric for investors to consider when comparing different zero-coupon bonds and assessing their potential returns.
By understanding these aspects of YTM, investors can make informed decisions when investing in zero-coupon bonds. YTM provides valuable insights into the potential return and risk associated with these bonds, enabling investors to optimize their investment strategies.
Present value
In the context of “how to calculate zero coupon bonds excel,” present value plays a pivotal role. Present value represents the current worth of a bond’s future cash flows, discounted at the yield-to-maturity (YTM). Understanding this concept is crucial for accurately calculating the value of zero-coupon bonds.
Present value acts as a bridge between the bond’s future cash flows and its current market price. By discounting the future cash flows at the YTM, investors can determine the present value of the bond, which reflects its fair market value. This calculation is essential for assessing the bond’s attractiveness and making informed investment decisions.
For example, consider a zero-coupon bond with a face value of $1,000 maturing in 10 years. If the current YTM is 5%, the present value of the bond can be calculated as follows: Present Value = $1,000 / (1 + 0.05)^10 = $613.91. This calculation helps investors determine the current price they should be willing to pay for the bond to achieve the expected YTM.
Understanding the connection between present value and zero-coupon bond calculations enables investors to make informed investment decisions. It allows them to assess the bond’s value, compare it to other investment options, and determine whether it aligns with their investment goals and risk tolerance.
Accrued Interest
Accrued interest plays a significant role in the context of “how to calculate zero coupon bonds excel”. It refers to the interest that has accumulated on a bond since the last interest payment date but has not yet been paid to the bondholder. Understanding accrued interest is crucial for accurate calculation of bond values and returns.
- Calculation: Accrued interest is calculated by multiplying the bond’s annual coupon rate by the number of days that have passed since the last interest payment date, divided by the number of days in the coupon period.
- Impact on Bond Price: Accrued interest affects the bond’s price. When a bond is purchased between interest payment dates, the buyer pays the seller the accrued interest in addition to the bond’s clean price.
- Relevance in Excel Calculations: In Excel, accrued interest is included in the calculation of the bond’s present value. It is added to the present value of the bond’s future cash flows to determine the bond’s fair market value.
Understanding accrued interest is essential for accurate bond valuation and return calculations. It enables investors to determine the total return they will receive from a bond investment, including both the periodic interest payments and any accrued interest.
Yield Curve
When calculating zero-coupon bonds in Excel, the yield curve is a crucial factor to consider. It graphically depicts the relationship between interest rates and time to maturity for bonds with similar credit risk, providing valuable insights for bond investors and analysts.
- Components of the Yield Curve: The yield curve consists of spot rates, which represent interest rates for different maturities on a specific day, and forward rates, which indicate the market’s expectations of future spot rates.
- Shape of the Yield Curve: The yield curve can take on different shapes, including upward sloping (indicating higher interest rates for longer maturities), downward sloping (indicating lower interest rates for longer maturities), or flat (indicating similar interest rates across different maturities).
- Factors Influencing the Yield Curve: Various factors influence the shape of the yield curve, including economic growth expectations, inflation expectations, monetary policy, and market sentiment.
- Implications for Bond Investors: The yield curve helps investors make informed decisions about bond investments. For example, an upward sloping yield curve suggests that investors may prefer bonds with shorter maturities, while a downward sloping yield curve indicates potential opportunities in longer-maturity bonds.
Understanding the yield curve and its implications is essential for calculating zero-coupon bonds in Excel. By incorporating yield curve data into their calculations, investors can accurately assess the present value and yield-to-maturity of zero-coupon bonds, enabling them to make informed investment decisions.
Callable bond
In the context of “how to calculate zero coupon bonds excel,” callable bonds present a unique consideration. These bonds grant the issuer the option to redeem them before their maturity date. Understanding the nuances of callable bonds is essential for accurate calculations and informed decision-making.
- Call Provision: The call provision outlines the specific terms and conditions under which the issuer can redeem the bond. It typically includes the call date, the call price, and any other relevant details.
- Call Premium: The call premium represents the additional amount the issuer must pay bondholders if the bond is called before maturity. The premium compensates investors for the loss of potential returns.
- Impact on Yield-to-maturity: The presence of a call provision can affect the calculation of a bond’s yield-to-maturity (YTM). The YTM may be lower for callable bonds compared to non-callable bonds with similar maturities due to the potential for early redemption.
- Issuer’s Perspective: Issuers may choose to call bonds when interest rates decline, allowing them to refinance the debt at lower rates. They may also call bonds if they no longer need the funds or if they have surplus cash.
Understanding the characteristics, implications, and calculations related to callable bonds is crucial for investors and analysts working with zero-coupon bonds in Excel. These factors influence the bond’s value, yield, and overall investment strategy.
FAQs on Calculating Zero Coupon Bonds in Excel
This section aims to clarify common questions and address misconceptions related to calculating zero coupon bonds in Excel. The FAQs cover essential concepts, step-by-step guidance, and practical considerations.
Question 1: What is the formula for calculating the yield-to-maturity (YTM) of a zero-coupon bond?
The YTM is calculated using the formula: YTM = (FV / PV)^(1/n) – 1, where FV is the face value, PV is the present value, and n is the number of years to maturity.
Question 2: How do I account for accrued interest when calculating the present value of a zero-coupon bond?
Accrued interest is added to the present value of the bond’s future cash flows before discounting to determine the bond’s fair market value.
Question 3: Can I use Excel’s built-in functions to calculate zero coupon bond values?
Yes, Excel provides functions such as PRICEINV and YIELDINV that can be used to calculate the price and yield of zero-coupon bonds, respectively.
Question 4: What factors affect the yield-to-maturity of a zero-coupon bond?
The YTM is influenced by factors such as the bond’s face value, time to maturity, prevailing interest rates, and market expectations.
Question 5: How do I compare the returns of zero-coupon bonds with coupon-bearing bonds?
To compare returns, calculate the yield-to-maturity or internal rate of return for both types of bonds, considering factors such as reinvestment rates and tax implications.
Question 6: What are the advantages and disadvantages of investing in zero-coupon bonds?
Advantages include potential for high returns and tax deferral, while disadvantages may include interest rate risk and lack of liquidity.
These FAQs provide a foundational understanding of the key concepts and considerations involved in calculating zero coupon bonds in Excel. For further insights and practical applications, refer to the comprehensive guide in the next section.
Transition to Next Section: Delve deeper into the intricacies of zero coupon bond calculations with step-by-step examples and advanced techniques in the following section.
Tips for Calculating Zero Coupon Bonds in Excel
This section provides a comprehensive set of actionable tips to guide you through the process of calculating zero coupon bond values in Excel:
Tip 1: Utilize Excel’s Built-in Functions: Leverage functions like PRICEINV and YIELDINV to simplify calculations and enhance accuracy.
Tip 2: Consider Accrued Interest: Accrued interest should be added to the bond’s present value to determine its fair market value.
Tip 3: Pay Attention to the Yield Curve: The yield curve provides insights into interest rate expectations and can influence the bond’s value.
Tip 4: Understand Callable Bond Provisions: Callable bonds offer the issuer the option to redeem before maturity, which can impact yield calculations.
Tip 5: Use Sensitivity Analysis: Perform sensitivity analysis to assess how changes in input parameters affect the bond’s value and yield.
Tip 6: Validate Your Results: Cross-check your calculations using alternative methods or online tools to ensure accuracy.
Tip 7: Seek Professional Guidance: For complex or high-stakes calculations, consider consulting a financial professional for expert advice.
By following these tips, you can enhance the accuracy and efficiency of your zero coupon bond calculations in Excel.
Transition to Conclusion: These tips provide a practical framework for navigating the intricacies of zero coupon bond calculations. In the next section, we will explore advanced techniques and best practices to further refine your understanding and decision-making abilities.
Conclusion
In this comprehensive guide, we have delved into the intricacies of calculating zero coupon bonds in Excel. Through a structured approach and practical examples, we have explored key concepts, functions, and considerations. Understanding the nuances of zero coupon bond calculations empowers investors and analysts to make informed decisions in fixed income markets.
The interplay between yield-to-maturity, present value, and time to maturity forms the foundation of zero coupon bond calculations. Accrued interest, yield curve analysis, and callable bond provisions further refine these calculations. By leveraging Excel’s built-in functions and employing sensitivity analysis, professionals can enhance the accuracy and efficiency of their calculations.