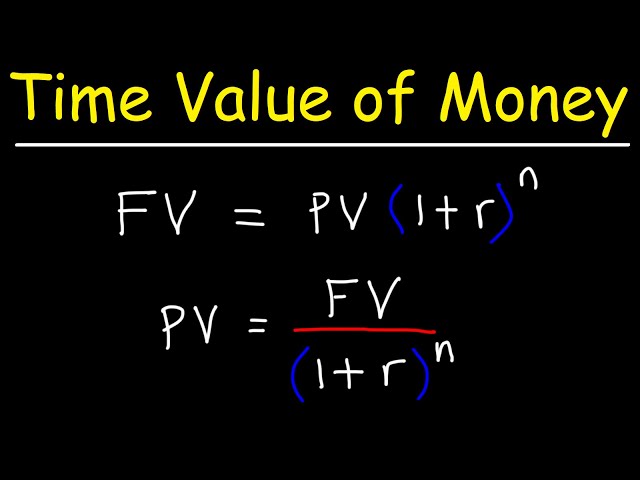
Future value calculations, an essential aspect of financial planning, determine the value of an investment at a specified future date. This calculation incorporates the concept of discount rate, the annual interest rate that reduces the future value to its present value. For instance, if you invest $10,000 with an expected annual return of 5% for five years, you can calculate its future value using a discount rate of 5%.
Understanding how to calculate future value using a discount rate empowers investors to make informed decisions about savings, investments, and long-term financial goals. It provides a basis for comparing investments and assessing their potential returns, aiding in financial planning and wealth management. The concept has its roots in financial mathematics and has been widely adopted in investment analysis and financial decision-making.
In this article, we will delve into the intricacies of calculating future value using discount rate by:
How to Calculate Future Value Using Discount Rate
Understanding the key aspects of calculating future value using a discount rate is crucial for informed financial decision-making.
- Formula and Calculation
- Time Period
- Discount Rate
- Present Value
- Compounding
- Inflation
- Annuity
- Applications
The formula for calculating future value considers the present value, discount rate, time period, and compounding frequency. The discount rate represents the opportunity cost of investing, while the time period determines the duration over which the investment grows. Compounding allows earnings to generate further earnings, and inflation must be factored in to account for the time value of money. Annuities, a series of regular payments, can also be incorporated into future value calculations. Understanding these aspects empowers individuals to make informed decisions about savings, investments, and long-term financial goals.
Formula and Calculation
The formula and calculation for future value using a discount rate are central to understanding how the time value of money impacts investments. Several key facets define the formula and its application.
- Present Value: The initial amount invested, which serves as the starting point for calculating future value.
- Discount Rate: The annual interest rate used to adjust future cash flows to their present value, representing the opportunity cost of investing.
- Time Period: The duration over which the investment is held, which determines the number of compounding periods.
- Compounding: The process of reinvesting earnings to generate further earnings, which accelerates the growth of the investment.
By incorporating these factors into the formula, investors can accurately project the future value of their investments, enabling informed decisions about savings, investments, and long-term financial planning.
Time Period
Time period, a crucial element in calculating future value using a discount rate, encompasses the duration over which an investment’s value grows. Understanding its multifaceted nature is essential for accurate financial planning and informed investment decisions.
- Investment Horizon: The period over which an investment is held, determining the number of compounding periods and the magnitude of future growth.
- Compounding Frequency: The frequency at which interest earned is added back to the principal, directly impacting the future value.
- Maturity Date: The specified date on which an investment matures, marking the end of the time period and the realization of the future value.
- Inflation: The rate at which prices rise over time, eroding the purchasing power of future returns and necessitating adjustments to the time period.
By considering these facets, investors can tailor their calculations to specific investment scenarios, ensuring that the time period aligns with their financial goals and risk tolerance. Accurately accounting for the time value of money through the time period component is fundamental for making informed financial decisions.
Discount Rate
Discount rate, a pivotal aspect of calculating future value, embodies the time value of money and adjusts future cash flows to their present value, reflecting the opportunity cost of investing. Understanding its multifaceted nature unravels the intricacies of future value calculations and informs sound financial decisions.
- Risk-Free Rate: The benchmark rate used to discount future cash flows, representing the return on a risk-free investment, often based on government bonds.
- Inflation: The rate at which prices rise over time, eroding the value of future returns and necessitating adjustments to the discount rate.
- Investment Horizon: The duration over which an investment is held, influencing the choice of discount rate as longer horizons warrant higher rates.
- Risk Premium: The additional return required to compensate for the risk associated with an investment, added to the risk-free rate to determine the appropriate discount rate.
These facets collectively shape the discount rate, which acts as a critical variable in future value calculations. It bridges the gap between future and present values, allowing investors to compare investments, assess potential returns, and make informed financial decisions aligned with their risk tolerance and investment goals.
Present Value
Present value, a foundational concept in finance, holds a critical connection with “how to calculate future value using a discount rate.” It represents the value of a future sum of money today, adjusted to reflect the time value of money and the opportunity cost of investing. Understanding this relationship is essential for informed financial decision-making.
Present value serves as a crucial component of calculating future value using a discount rate. The discount rate, which represents the rate of return an investor could earn on an alternative investment with similar risk, is applied to future cash flows to determine their present value. By summing the present values of all future cash flows, investors can arrive at the future value of an investment.
In real-life applications, present value plays a vital role in various financial scenarios. For instance, it is used to determine the fair value of bonds, where future coupon payments and the principal repayment are discounted back to the present to arrive at the bond’s price. Additionally, present value is employed in project evaluation, where future cash inflows and outflows are discounted to assess a project’s viability.
Understanding the connection between present value and calculating future value using a discount rate empowers individuals to make informed financial decisions. It enables them to compare investment options, assess the time value of money, and plan for future financial goals effectively.
Compounding
Compounding holds a central connection with “how to calculate future value using a discount rate.” It represents the phenomenon where interest earned on an investment is reinvested, generating further earnings. This snowball effect amplifies the growth of an investment over time, and understanding its impact is essential for accurate future value calculations and informed financial decisions.
Compounding is a critical component of calculating future value using a discount rate. The discount rate, which adjusts future cash flows to their present value, incorporates the effect of compounding. By reinvesting earnings and allowing them to compound, the future value of an investment grows at an accelerated pace compared to simple interest calculations.
In real-life applications, compounding plays a significant role in various financial instruments. For instance, in savings accounts and certificates of deposit (CDs), interest earned is compounded periodically, leading to a higher future value compared to accounts that do not offer compounding. Similarly, in the stock market, reinvested dividends contribute to the compounding effect, boosting long-term returns.
Understanding the connection between compounding and calculating future value using a discount rate empowers individuals to make informed financial decisions. It enables them to assess the long-term impact of compounding, compare investment options, and plan for the future with greater accuracy. By incorporating compounding into their calculations, they can make well-informed choices, such as maximizing retirement savings or planning for major financial goals.
Inflation
Inflation, a persistent rise in the general price level of goods and services, holds a direct connection with “how to calculate future value using a discount rate.” Understanding this relationship is crucial for accurate future value calculations and informed financial decision-making.
Inflation erodes the purchasing power of money over time, reducing the value of future cash flows. As a result, the discount rate used to calculate future value must account for inflation to provide a realistic assessment of an investment’s potential return. Ignoring inflation can lead to underestimating future value and, consequently, making poor investment decisions.
In real-life applications, inflation plays a significant role in various financial scenarios. For instance, when calculating the future value of a pension fund, inflation must be considered to ensure that retirees will have sufficient income to maintain their standard of living. Similarly, in project evaluation, future cash flows are adjusted for inflation to determine the project’s true profitability.
Understanding the connection between inflation and calculating future value using a discount rate empowers individuals to make informed financial decisions. It enables them to assess the impact of inflation on their investments, compare investment options, and plan for the future with greater accuracy. By incorporating inflation into their calculations, they can make well-informed choices, such as choosing investments that outpace inflation or adjusting their retirement savings goals accordingly.
Annuity
An annuity refers to a series of regular payments made at equal intervals over a specified period. It holds a critical connection with “how to calculate future value using a discount rate” due to its unique characteristics that align with the principles of future value calculations. By understanding this relationship, individuals can accurately assess the future value of annuity payments and make informed financial decisions.
In calculating future value using a discount rate, annuities play a central role as they represent a stream of future cash flows. The discount rate, which reflects the time value of money and the opportunity cost of investing, is applied to each annuity payment to determine its present value. By summing the present values of all future annuity payments, the future value of the annuity can be calculated.
Real-life examples of annuities include pension payments, regular withdrawals from retirement accounts, and structured settlements. In each case, the future value of the annuity is calculated using a discount rate to determine the present value of the future cash flows. This calculation is essential for ensuring that the annuity payments will provide sufficient income or meet the intended financial goals.
Understanding the connection between annuities and calculating future value using a discount rate is crucial for various practical applications. It enables individuals to compare different annuity options, assess the impact of inflation on future payments, and plan for their financial future. By incorporating annuities into their financial plans and calculations, they can make informed decisions that align with their long-term goals and risk tolerance.
Applications
The concept of “how to calculate future value using discount rate” finds practical applications in diverse financial scenarios, where understanding this relationship is essential for informed decision-making. It serves as a critical component in various financial applications, providing a framework to assess the time value of money and make sound investment choices.
Real-life examples abound where calculating future value using a discount rate plays a pivotal role. In the realm of investments, it enables individuals to compare the future value of different investment options, taking into account the time value of money and the opportunity cost of investing. This comparison empowers them to make informed choices that align with their financial goals and risk tolerance.
Furthermore, the concept finds application in financial planning, particularly in retirement planning and long-term savings. By calculating the future value of regular contributions and investment returns, individuals can estimate the corpus required to meet their future financial needs, ensuring a secure retirement or achieving specific financial milestones. The understanding of future value calculations empowers them to plan and invest accordingly.
In summary, the ability to calculate future value using a discount rate is a cornerstone of financial literacy, enabling individuals to make informed decisions in various financial contexts. It provides a practical tool to assess the time value of money, compare investment options, and plan for their financial future effectively.
Frequently Asked Questions
This section addresses common questions and provides clarification on key aspects of calculating future value using a discount rate.
Question 1: What is the formula for calculating future value using a discount rate?
Answer: The formula is FV = PV x (1 + r)^n, where FV is the future value, PV is the present value, r is the discount rate, and n is the number of periods.
Question 2: How does the discount rate affect future value?
Answer: A higher discount rate results in a lower future value because it represents a higher opportunity cost of investing.
Question 3: What is the difference between simple interest and compound interest in future value calculations?
Answer: Simple interest is calculated on the initial principal only, while compound interest is calculated on the growing balance, leading to a higher future value.
Question 4: How can I account for inflation in future value calculations?
Answer: To account for inflation, use a discount rate that incorporates an inflation premium.
Question 5: What are some real-life applications of future value calculations using a discount rate?
Answer: Applications include investment planning, retirement planning, and project evaluation.
Question 6: How can I learn more about calculating future value using a discount rate?
Answer: Refer to financial textbooks, online resources, or consult with a financial advisor for further guidance.
These FAQs provide a concise overview of the key concepts related to calculating future value using a discount rate. Understanding these concepts is crucial for making informed financial decisions and achieving long-term financial goals.
In the next section, we will delve deeper into the practical applications of future value calculations and explore how they can be used to evaluate investment opportunities and plan for the future effectively.
Tips for Calculating Future Value Using Discount Rate
To effectively calculate future value using a discount rate, consider these practical tips:
Tip 1: Choose the appropriate discount rate. Determine the rate that accurately reflects the time value of money and the risk associated with the investment.
Tip 2: Consider the time period. Accurately estimate the duration over which the investment will grow, as it influences the number of compounding periods.
Tip 3: Account for inflation. Adjust the discount rate to incorporate inflation to ensure realistic future value calculations.
Tip 4: Use a financial calculator or spreadsheet. Utilize tools that simplify the calculation process and minimize errors.
Tip 5: Understand the impact of compounding. Recognize the exponential growth effect of compounding and its influence on future value.
Tip 6: Compare different scenarios. Calculate future values under various assumptions to assess the sensitivity of the results.
Tip 7: Seek professional advice. Consult with a financial advisor for personalized guidance and to ensure accurate calculations.
By following these tips, you can enhance the accuracy and effectiveness of your future value calculations, leading to informed financial decision-making and successful financial planning.
These tips provide a solid foundation for understanding how to calculate future value using a discount rate. In the concluding section, we will explore advanced concepts and delve into practical applications of future value calculations in investment analysis and financial planning.
Conclusion
This comprehensive exploration of “how to calculate future value using discount rate” has shed light on the intricate relationship between the time value of money and investment decision-making. Key insights revolve around three main points:
- Future value calculations empower individuals to project the value of investments over time, considering factors like the discount rate, time period, and inflation.
- Understanding the impact of compounding is crucial, as it accelerates the growth of investments and significantly influences future returns.
- Properly accounting for inflation ensures realistic future value calculations, safeguarding against underestimating the true value of investments.
In essence, calculating future value using a discount rate is a fundamental skill for informed financial decision-making. It enables individuals to compare investment options, plan for their financial future, and make sound choices that align with their long-term goals.