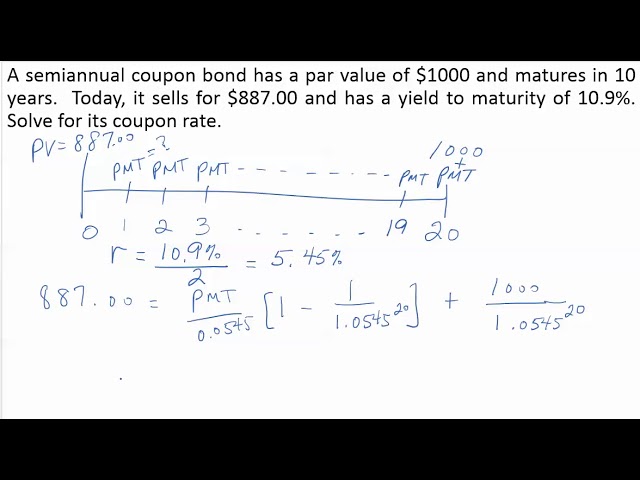
Calculating bond coupons is a vital aspect of understanding the fixed income market. A coupon represents the fixed interest payments made to bondholders over the life of the bond. For example, a bond with a $1,000 face value and a 5% coupon rate would pay $50 in interest payments each year.
Understanding coupon calculations is crucial for investors, as it helps them evaluate the returns and risks associated with bond investments. Historically, the concept of bond coupons emerged in the 17th century with the issuance of government bonds to fund wars and infrastructure projects.
This article delves into the detailed steps involved in calculating bond coupons, exploring the factors that influence coupon rates, and discussing their significance in the bond market.
How to Calculate Coupon in Bond
Calculating bond coupons is crucial for understanding bond investments. Key aspects to consider include:
- Face value
- Coupon rate
- Payment frequency
- Maturity date
- Accrued interest
- Yield to maturity
- Present value
- Future value
- Compound interest
These aspects are interconnected. For example, the coupon rate and payment frequency determine the coupon payment amount. The maturity date and yield to maturity influence the present and future value of the bond. Understanding these aspects helps investors evaluate the cash flows, risks, and returns associated with bond investments.
Face Value
Face value, also known as par value, is a fundamental aspect in calculating bond coupons. It represents the principal amount or the amount that the bondholder will receive upon the bond’s maturity.
- Nominal Value: The face value represents the amount of money that the bond issuer initially borrowed from the bondholder.
- Maturity Amount: The face value is the amount that the bond issuer promises to repay to the bondholder at the end of the bond’s term.
- Coupon Calculation Base: The face value serves as the base for calculating the bond’s coupon payments. The coupon rate is applied to the face value to determine the dollar value of each coupon payment.
- Bond Value Fluctuations: While the face value remains constant throughout the bond’s life, the market value of the bond can fluctuate based on factors such as interest rates and the creditworthiness of the issuer. However, at maturity, the bondholder is entitled to receive the face value, regardless of the market value.
Understanding the concept of face value is crucial for accurately calculating bond coupons and assessing the potential return on investment. It provides a solid foundation for further analysis of bond pricing and risk evaluation.
Coupon rate
The coupon rate is a crucial factor in calculating bond coupons and plays a vital role in determining the overall return on investment for bondholders. It represents the percentage of the face value that is paid to the bondholder as interest payments over the life of the bond.
- Nominal Rate: The coupon rate is typically expressed as an annual percentage of the face value. It is fixed at the time of bond issuance and remains constant throughout the bond’s life.
- Coupon Payments: The coupon rate directly determines the amount of each coupon payment. The payment is calculated by multiplying the coupon rate by the face value and dividing by the number of payment periods per year.
- Bond Pricing: The coupon rate is one of the key factors that influence the market price of a bond. Generally, bonds with higher coupon rates tend to trade at a premium to bonds with lower coupon rates.
- Interest Rate Risk: The coupon rate also affects the bond’s sensitivity to changes in interest rates. Bonds with higher coupon rates are less sensitive to interest rate fluctuations compared to bonds with lower coupon rates.
Understanding the concept of coupon rate is essential for accurately calculating bond coupons and evaluating the potential return and risk associated with bond investments. It provides a basis for comparing different bonds and making informed investment decisions.
Payment frequency
Payment frequency is a critical aspect of calculating bond coupons, influencing the timing and amount of interest payments received by bondholders. It refers to the number of times per year that coupon payments are made.
- Annual: The most common payment frequency, where bondholders receive one coupon payment each year, typically on the anniversary date of the bond’s issuance.
- Semi-annual: Bonds with semi-annual payments make two coupon payments each year, typically on the first day of June and December.
- Quarterly: Quarterly paying bonds distribute coupon payments every three months, typically on the first day of March, June, September, and December.
- Monthly: The least common payment frequency, where bondholders receive monthly interest payments, providing a consistent stream of income.
Payment frequency impacts the calculation of bond coupons as it determines the number of payment periods over which the annual coupon rate is divided. For instance, a bond with a 5% annual coupon rate and semi-annual payments would have a coupon payment of 2.5% (5% / 2) every six months. Understanding payment frequency is crucial for accurately calculating bond coupons and assessing the cash flow patterns of bond investments.
Maturity Date
The maturity date is a critical component of calculating bond coupons. It is the date on which the bond issuer repays the principal amount, or face value, of the bond to the bondholder. The maturity date determines the length of time over which the bondholder will receive coupon payments.
To calculate bond coupons, the annual coupon rate is divided by the number of payment periods per year. The resulting value is the coupon payment amount. The coupon payment amount is then multiplied by the number of years until the maturity date to determine the total amount of coupon payments that the bondholder will receive over the life of the bond.
For example, consider a bond with a face value of $1,000, a coupon rate of 5%, and a maturity date of 10 years. The annual coupon payment would be $50 (0.05 1,000). The total amount of coupon payments that the bondholder would receive over the life of the bond would be $500 (10 years 50 per year).
Understanding the relationship between the maturity date and coupon calculations is crucial for bond investors. It allows them to assess the potential return on their investment and make informed decisions about which bonds to purchase.
Accrued Interest
Accrued interest, a crucial aspect of “how to calculate coupon in bond” represents the interest that has accumulated on a bond but has not yet been paid to the bondholder. Understanding accrued interest is essential for accurate coupon calculations and assessing the overall return on bond investments.
- Calculation: Accrued interest is calculated by multiplying the bond’s annual coupon rate by the number of days since the last coupon payment divided by the number of days in the coupon payment period.
- Example: Consider a bond with a $1,000 face value, a 5% coupon rate, and semi-annual coupon payments. If 30 days have passed since the last coupon payment, the accrued interest would be $7.50 [(0.05 1,000) 30 / 180].
- Impact on Bond Price: Accrued interest is added to the bond’s purchase price when buying a bond between coupon payment dates. This is because the buyer is entitled to receive the accrued interest upon the next coupon payment.
- Importance for Investors: Accrued interest affects the yield to maturity (YTM) calculations, which represent the annualized rate of return an investor can expect to receive from a bond if held until maturity.
Comprehending accrued interest is fundamental for investors to determine the true cost of a bond investment and to make informed decisions based on accurate calculations of bond coupons and yields.
Yield to maturity
Yield to maturity (YTM) is a crucial concept in bond pricing and return calculations. In relation to “how to calculate coupon in bond,” it represents the annualized rate of return an investor can expect to receive if they hold the bond until its maturity date.
- Current Yield: The ratio of the bond’s annual coupon payment to its current market price, providing a snapshot of the current return on investment.
- YTM vs. Coupon Rate: YTM may differ from the bond’s coupon rate due to factors like interest rate changes and market conditions.
- Price and YTM Relationship: Bond prices and YTM have an inverse relationship; as prices rise, YTM falls, and vice versa.
- Implications for Investors: YTM helps investors make informed decisions by comparing potential returns from different bonds and assessing the impact of interest rate fluctuations on their investments.
Comprehending YTM is essential for accurately calculating bond coupons and evaluating the potential return on investment. It provides a more comprehensive view of a bond’s value than simply looking at the coupon rate and aids in making sound financial decisions.
Present value
In the context of “how to calculate coupon in bond,” present value (PV) plays a crucial role in determining the fair value of a bond. It represents the current worth of future cash flows associated with the bond, including both coupon payments and the repayment of the principal amount (face value) at maturity.
To calculate the PV of a bond, one needs to discount the future cash flows back to the present using an appropriate discount rate. This discount rate is typically determined by the market interest rates or the yield to maturity (YTM) of the bond. The PV of each future cash flow is calculated as its nominal value multiplied by the discount factor, which is the present value of $1 received at that future point in time.
Real-life examples of PV in “how to calculate coupon in bond” include determining the fair value of a bond before purchase, calculating the break-even point for a bond investment, and assessing the impact of changes in interest rates on bond prices. Understanding PV is essential for making informed decisions about bond investments and managing bond portfolios effectively.
In summary, PV is a critical component of “how to calculate coupon in bond” as it allows investors to determine the current value of future cash flows and make informed investment decisions. It provides a basis for comparing different bonds, evaluating the impact of interest rate changes, and ultimately optimizing bond investment strategies.
Future value
In the context of “how to calculate coupon in bond,” future value (FV) plays a significant role in determining the potential return on investment. FV represents the value of a bond’s future cash flows, including coupon payments and the repayment of the principal amount at maturity, at a specified future date.
To calculate the FV of a bond, one needs to apply the concept of compound interest. Each future cash flow is multiplied by a factor that accounts for the accumulation of interest over time. The future value of the bond is then calculated as the sum of the future values of all the individual cash flows. The discount rate used in these calculations is typically the bond’s yield to maturity (YTM).
Understanding FV is essential for making informed investment decisions. By calculating the FV of a bond, investors can assess its potential return and compare it to other investment options. Real-life examples of FV in “how to calculate coupon in bond” include determining the maturity value of a bond, calculating the value of a bond fund’s portfolio, and projecting the future value of an investment in bonds.
In summary, FV is a critical component of “how to calculate coupon in bond” as it allows investors to determine the potential value of their investment at a future point in time. It provides a basis for making informed decisions about bond investments and optimizing bond portfolio strategies.
Compound interest
Compound interest plays a crucial role in understanding “how to calculate coupon in bond” as it determines the growth of an investment over time, including both the initial principal and the accumulated interest. This concept is central to bond calculations and has several key facets:
- Exponential growth:
Compound interest allows investments to grow exponentially, as the interest earned in each period is reinvested and earns interest itself, leading to a snowball effect. - Time value of money:
Compound interest underscores the time value of money. The longer an investment remains invested, the greater the potential for substantial growth due to the compounding effect. - Impact on coupon payments:
Compound interest directly affects coupon payments. The interest earned on previously paid coupons is added to the principal, increasing the base amount on which future coupons are calculated. - Real-life applications:
Compound interest has numerous real-life applications, such as savings accounts, certificates of deposit, and bonds. Understanding its significance enables investors to make informed decisions about their financial strategies.
Compound interest is an essential aspect of “how to calculate coupon in bond” as it influences the present and future value of an investment. It highlights the importance of time and the power of reinvesting earnings to maximize returns, providing investors with a deeper understanding of bond calculations and investment planning.
Frequently Asked Questions about “How to Calculate Coupon in Bond”
This section addresses common questions and clarifies aspects related to calculating bond coupons. Each question is answered concisely to provide a comprehensive understanding of the topic.
Question 1: What is the formula for calculating the coupon payment of a bond?
To calculate the coupon payment, multiply the bond’s face value by the annual coupon rate and divide by the number of payment periods per year.
Question 2: How does the maturity date affect coupon calculations?
The maturity date determines the period over which coupon payments are received. It also influences the calculation of accrued interest and the bond’s present and future values.
Question 3: What is the relationship between yield to maturity (YTM) and coupon rate?
YTM and coupon rate may differ due to changes in market conditions and interest rates. Understanding this relationship is crucial for accurately calculating bond coupons and assessing potential returns.
Question 4: How is compound interest applied to bond coupon calculations?
Compound interest affects the growth of coupon payments over time. The interest earned on previously paid coupons is added to the principal, increasing the base amount for future coupon calculations.
Question 5: What is the impact of payment frequency on coupon calculations?
Payment frequency determines how often coupon payments are made. It affects the timing and amount of interest payments received by bondholders.
Question 6: How can I use coupon calculations to evaluate bond investments?
Coupon calculations provide insights into a bond’s potential return, risk, and cash flow patterns. They aid in comparing different bonds and making informed investment decisions.
In summary, these FAQs covered essential aspects of calculating bond coupons, including the impact of various factors on coupon payments. Understanding these concepts helps investors navigate the bond market and make well-informed investment choices.
Moving forward, the next section delves into advanced topics related to bond coupon calculations, including the effect of interest rate fluctuations and strategies for maximizing returns.
Tips for Calculating Bond Coupons
This section provides actionable tips to enhance your understanding of bond coupon calculations and maximize your investment returns:
Tip 1: Understand the Basics: Grasp the fundamental concepts of face value, coupon rate, maturity date, and payment frequency to lay a solid foundation for accurate calculations.
Tip 2: Apply the Formula: Utilize the formula (Coupon Payment = Face Value Coupon Rate Payment Frequency) to determine the dollar value of each coupon payment.
Tip 3: Consider Accrued Interest: Factor in accrued interest when purchasing bonds between coupon payment dates to account for the accumulated but unpaid interest.
Tip 4: Calculate Yield to Maturity (YTM): Determine the annualized rate of return you can expect to receive if you hold the bond until its maturity date, considering the current market price and future cash flows.
Tip 5: Evaluate Present and Future Values: Calculate the present value to determine the current worth of future cash flows and the future value to assess the potential growth of your investment over time.
Tip 6: Compare Different Bonds: Use coupon calculations to compare the potential returns and risks associated with various bonds, aiding in informed investment decisions.
Tip 7: Monitor Interest Rate Changes: Stay informed about interest rate fluctuations and their impact on bond prices and coupon payments to adjust investment strategies accordingly.
Tip 8: Seek Professional Advice: If needed, consult with a financial advisor or bond specialist for personalized guidance and in-depth analysis of complex bond investments.
By implementing these tips, you can enhance the accuracy of your bond coupon calculations, make informed investment decisions, and optimize your returns in the bond market.
The following section explores advanced strategies for maximizing bond returns, building upon the foundational knowledge gained from these tips.
Conclusion
This comprehensive guide has delved into the intricacies of “how to calculate coupon in bond,” providing a solid foundation for understanding bond investments. Key ideas explored include the concepts of face value, coupon rate, maturity date, and payment frequency, which are essential for accurate coupon calculations.
Two main points to reemphasize are the impact of yield to maturity (YTM) and the importance of considering accrued interest. YTM represents the annualized return on investment, while accrued interest accounts for the accumulated interest earned but not yet paid when purchasing a bond between coupon payment dates. Understanding these factors allows investors to make informed decisions and optimize their bond portfolio strategies.
Remember, accurate coupon calculations are crucial for evaluating potential returns, comparing different bonds, and navigating the bond market effectively. By mastering these techniques, investors can enhance their financial literacy and make smarter investment choices.