Discount terminal value (DTV) is an economic measure that determines the present value of a future cash inflow or outflow at a specified discount rate. It is a critical factor in determining the value of long-term assets and the cost of capital. For instance, a company might use DTV to assess the value of its investment in a new machine.
DTV is relevant because it helps businesses make informed decisions about investments and financial planning. It provides a more accurate representation of the future value of cash flows, taking into account the time value of money. Historically, the concept of DTV has evolved as financial professionals have developed more sophisticated methods for valuing assets and forecasting future cash flows.
This article will explore the formula for calculating DTV, discuss its applications in finance, and provide practical examples of how it is used in business decision-making.
How to Calculate Discount Terminal Value
Discount terminal value (DTV) is a crucial financial concept used to determine the present value of future cash flows. Its calculation involves several key aspects:
- Discount rate
- Terminal growth rate
- Terminal cash flow
- Projection period
- Perpetuity
- Horizon value
- Present value
- Time value of money
- Investment valuation
These aspects are interconnected and play vital roles in accurately calculating DTV. The discount rate represents the cost of capital and the risk associated with the investment, while the terminal growth rate reflects the expected long-term growth of the cash flows. Terminal cash flow is the cash flow at the end of the projection period, which is typically assumed to grow at a constant rate in perpetuity. The horizon value is the present value of the cash flows beyond the projection period, which is calculated using the perpetuity formula. Understanding these aspects is essential for effectively applying the DTV concept in financial analysis and decision-making.
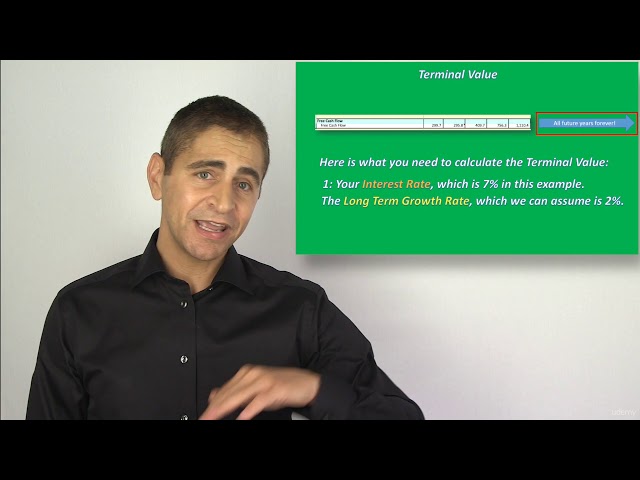
Discount Rate
Discount rate plays a critical role in the calculation of discount terminal value (DTV). It represents the cost of capital, which is the minimum rate of return that investors expect to receive for investing in a particular asset. The discount rate is used to determine the present value of future cash flows, which are then used to calculate the DTV.
The discount rate has a significant impact on the DTV. A higher discount rate will result in a lower DTV, and vice versa. This is because a higher discount rate means that the future cash flows are less valuable in present terms. As a result, the DTV will be lower.
In practice, the discount rate is often determined using the weighted average cost of capital (WACC). The WACC is a calculation that takes into account the cost of both debt and equity financing. It is used to determine the overall cost of capital for a company.
Understanding the relationship between discount rate and DTV is important for financial analysts and investors. It allows them to make informed decisions about the value of assets and the cost of capital.
Terminal Growth Rate
In the context of calculating discount terminal value (DTV), terminal growth rate plays a significant role in determining the long-term value of future cash flows. It is the rate at which cash flows are expected to grow beyond the explicit forecast period, representing the sustainable growth of the business.
- Constant Growth: Terminal growth rate often assumes a constant growth rate for cash flows in perpetuity, reflecting a steady and predictable growth trajectory.
- Industry Benchmarks: Businesses may refer to industry benchmarks or peer comparisons to establish a reasonable terminal growth rate based on historical trends and industry dynamics.
- Economic Factors: Macroeconomic factors such as inflation, interest rates, and GDP growth influence the terminal growth rate, affecting the overall economic environment and business performance.
- Company-Specific Factors: The terminal growth rate also considers company-specific factors like competitive advantages, market share, and management capabilities, which can impact the long-term growth potential.
Understanding the terminal growth rate’s components and implications is crucial for accurate DTV calculation. By incorporating realistic assumptions about long-term cash flow growth, businesses can better estimate the present value of future cash flows and make informed decisions regarding investment valuations and financial planning.
Terminal cash flow
Within the framework of calculating discount terminal value (DTV), terminal cash flow holds significant importance as it represents the cash flow generated at the end of the explicit forecast period. This cash flow serves as the foundation for projecting future cash flows and determining the present value of those cash flows.
- Stable Cash Flow: Terminal cash flow is often assumed to be stable or growing at a constant rate, reflecting the expectation of ongoing business operations and revenue generation.
- Perpetual Growth: The terminal cash flow is assumed to continue in perpetuity, implying that the business is expected to operate indefinitely.
- Normalization: In practice, terminal cash flow may be normalized to account for unusual or non-recurring items that could distort the cash flow in the final year of the explicit forecast period.
- Industry Analysis: Businesses often consider industry benchmarks and peer comparisons to establish a reasonable terminal cash flow assumption based on historical trends and industry dynamics.
Understanding the concept and implications of terminal cash flow is crucial for accurate DTV calculation. By incorporating realistic assumptions about the terminal cash flow, businesses can better estimate the present value of future cash flows and make informed decisions regarding investment valuations and financial planning.
Projection period
In the context of calculating discount terminal value (DTV), the projection period holds significant importance, as it represents the duration over which explicit cash flow forecasts are made before transitioning to a terminal growth rate assumption. This period is a critical component of DTV calculation, as it directly influences the estimated present value of future cash flows.
The projection period is typically determined based on several factors, including the industry, business model, and availability of reliable data. A longer projection period allows for more detailed and accurate cash flow forecasting, but it also introduces greater uncertainty. Conversely, a shorter projection period may limit the accuracy of cash flow estimates, but it reduces the impact of uncertainty.
Real-life examples of projection periods in DTV calculations can be found in various industries. For instance, in the technology sector, a projection period of 5-7 years may be appropriate due to the rapidly evolving nature of the industry. In contrast, a utility company may use a projection period of 10-15 years due to the more stable and predictable nature of its cash flows.
Understanding the relationship between projection period and DTV calculation is crucial for financial analysts and investors. It allows them to make informed decisions about the appropriate projection period based on the specific circumstances and the level of uncertainty involved. By carefully considering the projection period, businesses can improve the accuracy of their DTV calculations and make more informed investment and financial planning decisions.
Perpetuity
In the framework of how to calculate discount terminal value (DTV), the concept of perpetuity plays a pivotal role. Perpetuity, in this context, refers to the assumption that a stream of cash flows will continue indefinitely into the future at a constant rate. This assumption is crucial for calculating the terminal value of a project or investment because it allows us to estimate the present value of all future cash flows beyond the explicit projection period.
Perpetuity is a critical component of DTV calculation as it provides a means to account for the ongoing value of a project or investment beyond the finite projection period. By assuming a constant growth rate in perpetuity, we can estimate the terminal value as the present value of an infinite stream of cash flows. This terminal value then forms a significant portion of the overall DTV calculation.
In practice, the assumption of perpetuity is commonly used in industries with stable and predictable cash flow patterns, such as utilities, real estate, and certain types of infrastructure projects. For instance, in the case of a long-term lease agreement, the rental payments can be assumed to continue in perpetuity, providing a basis for calculating the DTV of the investment.
Understanding the connection between perpetuity and DTV calculation is essential for financial analysts and investors. It allows them to accurately estimate the long-term value of projects and investments, particularly those with long-term cash flow horizons. By incorporating the concept of perpetuity into DTV calculations, businesses can make more informed decisions regarding capital budgeting, investment appraisal, and financial planning.
Horizon value
In the context of how to calculate discount terminal value (DTV), horizon value plays a critical role in determining the overall value of a project or investment. Horizon value refers to the present value of all cash flows that are expected to occur beyond the explicit projection period, assuming a constant growth rate in perpetuity. It is the discounted value of the terminal cash flow, which is the cash flow generated at the end of the projection period.
Horizon value is a critical component of DTV calculation because it captures the long-term value of the project or investment beyond the finite projection period. By incorporating the horizon value, businesses can more accurately estimate the overall worth of their investments and make informed decisions regarding capital budgeting and financial planning.
In practice, horizon value finds applications in various industries and scenarios. For example, in the valuation of real estate properties, the horizon value represents the present value of the rental income expected to be generated over the property’s remaining economic life. Similarly, in infrastructure projects, the horizon value may represent the present value of future toll revenue or other forms of long-term income.
Understanding the connection between horizon value and DTV calculation is essential for financial analysts and investors. It allows them to accurately assess the long-term potential of projects and investments, especially those with long-term cash flow horizons. By considering the horizon value, businesses can make more informed decisions regarding investment strategies, risk management, and overall financial planning.
Present value
Present value (PV) plays a critical role in the calculation of discount terminal value (DTV). PV is the current worth of a future sum of money or stream of cash flows, discounted at a specified rate of return. In the context of DTV, PV is used to determine the present value of the terminal cash flow, which is the cash flow generated at the end of the explicit projection period.
PV is a critical component of DTV calculation because it allows us to compare the value of future cash flows to their present-day value. This is important because cash flows that occur in the future are worth less than cash flows that occur today due to the time value of money. By discounting future cash flows to their present value, we can determine their true economic value and incorporate them into the calculation of DTV.
In practice, PV is used in a wide range of financial applications, including investment valuation, capital budgeting, and financial planning. For example, in the valuation of a stock, PV is used to determine the present value of the future dividends that the stock is expected to pay. Similarly, in capital budgeting, PV is used to evaluate the profitability of a potential investment by comparing the present value of the future cash flows to the initial investment cost.
Understanding the connection between PV and DTV calculation is essential for financial analysts and investors. It allows them to accurately assess the value of long-term investments and make informed decisions regarding capital allocation and financial planning.
Time value of money
The time value of money (TVM) is a fundamental concept in finance that recognizes the different values of money at different points in time. In the context of calculating discount terminal value (DTV), TVM plays a critical role in determining the present value of future cash flows.
- Present value vs. future value: TVM emphasizes that a sum of money today is worth more than the same sum in the future due to its potential earning power. This concept forms the basis of DTV calculation, as future cash flows are discounted to their present value to determine their true economic value.
- Discount rate: The discount rate used in DTV calculation represents the rate of return that could be earned on an alternative investment with similar risk. By discounting future cash flows at the appropriate discount rate, we can account for the time value of money and compare them on an equal footing.
- Compound interest: TVM incorporates the effect of compound interest, which allows money to grow exponentially over time. In DTV calculation, compound interest is considered when determining the future value of cash flows, ensuring that the time value of money is accurately reflected.
- Inflation: Inflation erodes the purchasing power of money over time. In DTV calculation, inflation is accounted for by using real discount rates that adjust for the expected rate of inflation. This ensures that the true economic value of future cash flows is captured.
Understanding the time value of money is crucial for accurately calculating DTV. By incorporating TVM into the calculation, businesses and investors can make informed decisions about long-term investments and financial planning.
Investment valuation
Investment valuation is the process of determining the value of an investment. It is a critical component of financial analysis and decision-making, as it helps investors assess the potential risks and returns of an investment and make informed decisions about whether to buy, sell, or hold an asset. Discount terminal value (DTV) plays a crucial role in investment valuation, as it provides a method for valuing long-term investments and determining their present value.
DTV is the present value of all future cash flows that an investment is expected to generate beyond the explicit forecast period. It is calculated using a discount rate that reflects the opportunity cost of capital and the risk associated with the investment. By discounting future cash flows to their present value, investors can compare investments with different time horizons and risk profiles on an equal footing.
Real-life examples of DTV applications in investment valuation include:
- Company valuation: DTV is used to value companies by estimating the present value of their future cash flows. This is important for mergers and acquisitions, as well as for determining the intrinsic value of a company’s stock.
- Project evaluation: DTV is used to evaluate long-term investment projects, such as capital expenditures or research and development projects. By considering the time value of money, DTV helps investors make informed decisions about whether to proceed with an investment.
- Real estate valuation: DTV is used to value real estate properties by estimating the present value of future rental income and capital appreciation. This is important for investors looking to buy, sell, or refinance a property.
Understanding the connection between investment valuation and DTV is essential for financial analysts and investors. It allows them to accurately assess the value of long-term investments and make informed decisions about capital allocation and financial planning. By incorporating DTV into investment valuation, investors can increase their chances of making profitable investment decisions and achieving their financial goals.
Frequently Asked Questions (FAQs) on How to Calculate Discount Terminal Value
This section addresses common queries and misconceptions regarding the calculation of discount terminal value (DTV). These FAQs aim to clarify essential aspects of DTV and provide practical guidance for its application.
Question 1: What is the significance of the discount rate in DTV calculation?
Answer: The discount rate represents the cost of capital or the minimum acceptable rate of return for an investment. It is a crucial factor that influences the present value of future cash flows and thus the overall DTV.
Question 2: How is terminal growth rate determined?
Answer: Terminal growth rate reflects the expected long-term growth rate of cash flows beyond the explicit forecast period. It can be estimated based on industry benchmarks, company-specific factors, and macroeconomic conditions.
Question 3: What is the purpose of assuming perpetuity in DTV calculation?
Answer: Perpetuity assumes that cash flows will continue indefinitely at a constant growth rate. This simplifies the calculation of the terminal value, which represents the present value of all future cash flows beyond the forecast period.
Question 4: How does inflation impact DTV calculation?
Answer: Inflation reduces the purchasing power of money over time. To account for this, real discount rates adjusted for inflation should be used in DTV calculation to ensure accurate valuation.
Question 5: Can DTV be used to value different types of investments?
Answer: Yes, DTV is a versatile tool that can be applied to value various investments, including stocks, bonds, real estate, and long-term projects.
Question 6: What are the limitations of using DTV for investment valuation?
Answer: While DTV provides a valuable estimate of long-term value, it relies on assumptions about future cash flows and growth rates. Therefore, it is essential to consider these assumptions and their potential impact on the accuracy of the DTV calculation.
These FAQs offer insights into the key considerations and applications of DTV calculation. By addressing common questions, they enhance the understanding and practical use of this important financial valuation technique.
The next section will delve deeper into the practical applications of DTV, showcasing real-world examples and case studies to demonstrate its significance in investment decision-making.
Tips for Calculating Discount Terminal Value
This section provides practical tips to enhance the accuracy and effectiveness of discount terminal value (DTV) calculations. By following these guidelines, financial analysts and investors can improve their ability to assess the long-term value of investments and make informed decisions.
Tip 1: Determine an appropriate discount rate. The discount rate should reflect the cost of capital and the risk associated with the investment. Consider using the weighted average cost of capital (WACC) to account for both debt and equity financing.
Tip 2: Estimate a realistic terminal growth rate. The terminal growth rate should represent the sustainable long-term growth rate of cash flows. Consider industry benchmarks, company-specific factors, and macroeconomic conditions when making this estimate.
Tip 3: Use a consistent projection period. The projection period should be long enough to capture the majority of the investment’s cash flows. However, it should not be so long that the accuracy of the cash flow forecasts becomes questionable.
Tip 4: Consider inflation. Inflation erodes the purchasing power of money over time. Use real discount rates that adjust for inflation to ensure that the DTV accurately reflects the future value of cash flows.
Tip 5: Test the sensitivity of the DTV to changes in assumptions. Perform sensitivity analysis to assess how the DTV changes when key assumptions, such as the discount rate or terminal growth rate, are varied. This analysis can provide valuable insights into the robustness of the DTV calculation.
Tip 6: Use DTV in conjunction with other valuation methods. DTV is a valuable tool, but it should not be used in isolation. Consider using other valuation methods, such as discounted cash flow analysis or comparable company analysis, to triangulate the investment’s value.
Tip 7: Seek professional advice. If you are unsure about how to calculate DTV or interpret the results, consider seeking advice from a qualified financial advisor or investment professional.
By following these tips, you can improve the accuracy and reliability of your DTV calculations. This will enable you to make more informed investment decisions and enhance your overall financial planning.
The next section will explore real-world examples of DTV calculation, demonstrating its practical applications in investment analysis and decision-making.
Conclusion
In summary, this article has explored the intricacies of calculating discount terminal value (DTV), a crucial concept in investment valuation and financial planning. We have emphasized the importance of using an appropriate discount rate, estimating a realistic terminal growth rate, and considering inflation when performing DTV calculations.
Key takeaways from this article include:
- DTV is a valuable tool for assessing the long-term value of investments, but it is essential to use it in conjunction with other valuation methods and sensitivity analysis to ensure accuracy.
- The discount rate, terminal growth rate, and projection period are critical inputs that significantly impact the DTV calculation, and they should be carefully considered and justified.
- DTV provides insights into the future value of cash flows, enabling informed investment decisions and effective financial planning.
As the financial landscape continues to evolve, the ability to accurately calculate DTV will remain a cornerstone of sound investment practices. By understanding the concepts and applications discussed in this article, financial analysts, investors, and business leaders can enhance their decision-making and achieve their long-term financial goals.