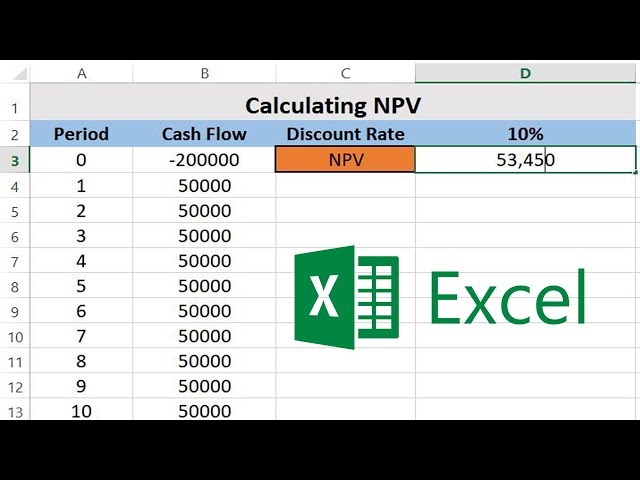
Discount rate for net present value (NPV) is a critical concept in finance to evaluate the attractiveness of investment projects. It is a rate that reflects the value of money over time, considering factors such as inflation, risk, and opportunity cost.
Understanding how to calculate the discount rate for NPV in Excel empowers financial analysts to evaluate projects accurately. It helps them make informed decisions about investment opportunities, allocate capital efficiently, and maximize returns.
The development of spreadsheet software like Excel revolutionized the calculation of the discount rate for NPV. It automated complex computations, making the process faster and less prone to error.
How to Calculate Discount Rate for NPV in Excel
Accurate calculation of the discount rate for Net Present Value (NPV) in Excel is essential for evaluating investment projects and making well-informed financial decisions.
- Project cash flows
- Risk assessment
- Time value of money
- Cost of capital
- Weighted average cost of capital (WACC)
- Inflation
- Opportunity cost
- Excel formulas
- NPV calculation
Understanding these aspects allows you to calculate the discount rate accurately, considering factors such as the time value of money, the risk associated with the project, and the cost of capital. This ensures that NPV calculations are reliable and provide meaningful insights into the potential profitability of investment projects.
Project cash flows
Project cash flows are the lifeblood of any investment project evaluation, including Net Present Value (NPV) analysis. They represent the expected inflows and outflows of cash over the project’s lifetime and are a critical component of calculating the discount rate for NPV in Excel.
The discount rate, in turn, is the rate at which future cash flows are discounted to calculate their present value. Accurate estimation of the discount rate is crucial for reliable NPV calculations and decision-making. Factors such as the time value of money, inflation, and risk must be considered when determining the appropriate discount rate.
The connection between project cash flows and the discount rate is evident in the NPV formula: NPV = -Initial Investment + Sum of (Cash Flow / (1 + Discount Rate)^Period) From this formula, we can see that the discount rate directly impacts the present value of future cash flows, which in turn affects the overall NPV calculation. Thus, understanding project cash flows and their relationship with the discount rate is essential for accurate project evaluation and decision-making.
Risk assessment
Risk assessment plays a critical role in determining the appropriate discount rate for Net Present Value (NPV) calculations in Excel. It involves evaluating the level of uncertainty and potential risks associated with an investment project and incorporating that assessment into the discount rate.
The discount rate serves as a benchmark against which future cash flows are discounted to calculate their present value. A higher discount rate implies greater risk and reduces the present value of future cash flows, while a lower discount rate indicates lower risk and a higher present value. Therefore, accurate risk assessment is essential to ensure that the discount rate used in NPV calculations reflects the true risk profile of the project.
In practice, risk assessment for NPV calculations considers various factors such as market volatility, industry dynamics, regulatory changes, and geopolitical events. Financial analysts use a combination of quantitative and qualitative analysis to assess risk and determine an appropriate risk premium to be added to the risk-free rate to arrive at the discount rate.
Understanding the connection between risk assessment and discount rate calculation is crucial for making informed investment decisions. By incorporating a realistic assessment of risk into the discount rate, financial analysts can ensure that NPV calculations accurately reflect the potential risks and rewards of an investment project.
Time value of money
The time value of money (TVM) is a fundamental concept in financial analysis and plays a crucial role in calculating the discount rate for Net Present Value (NPV) in Excel. It reflects the idea that money available today is worth more than the same amount in the future due to its potential earning power.
- Present value: The present value of a future sum of money is its current worth, discounted at a specified interest rate to reflect its time value.
- Future value: The future value of a present sum of money is the amount it will grow to at a specified interest rate over a given period.
- Compounding: Compounding refers to the growth of an investment over time, where interest is added to the principal, and interest is earned on the accumulated amount.
- Discounting: Discounting is the opposite of compounding and involves calculating the present value of future cash flows by dividing them by (1 + discount rate) raised to the power of the number of periods.
Understanding the time value of money and its components is essential for accurately calculating the discount rate in NPV analysis. By considering the time value of money, financial analysts can make informed investment decisions that account for the potential earning power and risk associated with future cash flows.
Cost of capital
The cost of capital is a critical concept in calculating the discount rate for Net Present Value (NPV) in Excel. It represents the minimum rate of return that a company must earn on its investments to satisfy its stakeholders, including shareholders and creditors. Accurately determining the cost of capital is essential for making sound investment decisions and ensuring that projects meet or exceed the required rate of return.
- Debt cost: The cost of borrowing funds through loans or bonds, typically represented by the interest rate paid.
- Equity cost: The cost of raising capital through the issuance of common or preferred stock, often estimated using the Capital Asset Pricing Model (CAPM).
- Weighted average cost of capital (WACC): A blended cost of capital that considers both debt and equity costs, weighted by their respective proportions in the company’s capital structure.
- Risk premium: An additional return required by investors to compensate for the risk associated with an investment, typically estimated using beta coefficients or historical data.
These components of the cost of capital play a crucial role in determining the appropriate discount rate for NPV calculations. By considering the cost of debt, equity, and risk, financial analysts can ensure that the discount rate accurately reflects the company’s financing structure and the level of risk associated with the project being evaluated.
Weighted average cost of capital (WACC)
Weighted average cost of capital (WACC) is a critical component in calculating the discount rate for Net Present Value (NPV) in Excel. It represents the average cost of capital that a company incurs to finance its operations and investments. WACC considers both the cost of debt and equity, weighted by their respective proportions in the company’s capital structure.
The discount rate, in turn, is a rate used to determine the present value of future cash flows. Accurately calculating the discount rate is essential for making sound investment decisions and ensuring that projects meet or exceed the required rate of return. By considering WACC as a component of the discount rate, financial analysts can ensure that the discount rate reflects the company’s financing structure and the cost of capital.
In practice, WACC is calculated using the following formula: WACC = (E/V x Re) + (D/V x Rd x (1 – Tc))where: – E is the market value of the company’s equity – V is the total market value of the company (E + D) – Re is the cost of equity – D is the market value of the company’s debt – Rd is the cost of debt – Tc is the corporate tax rate
Understanding the connection between WACC and the discount rate is crucial for financial analysts and investors. By considering WACC in NPV calculations, they can make informed investment decisions that account for the company’s cost of capital and financing structure.
Inflation
Inflation is a critical factor that impacts the calculation of the discount rate for Net Present Value (NPV) in Excel. It represents the rate at which the general price level of goods and services increases over time, reducing the purchasing power of money.
In NPV calculations, the discount rate is used to determine the present value of future cash flows. Inflation affects the calculation of the discount rate because it reduces the value of future cash flows in real terms. As inflation increases, the purchasing power of money decreases, which means that each unit of currency will be able to purchase fewer goods and services in the future. Therefore, a higher inflation rate will result in a higher discount rate.
Understanding the connection between inflation and the discount rate is crucial for accurate NPV calculations. By considering inflation, financial analysts can adjust the discount rate to reflect the expected decrease in the purchasing power of money over the project’s lifetime. This ensures that the NPV calculation accurately reflects the real value of future cash flows and provides a more realistic assessment of a project’s profitability.
Opportunity cost
Opportunity cost is a fundamental concept in financial analysis that plays a crucial role in calculating the discount rate for Net Present Value (NPV) in Excel. It represents the value of the next best alternative that is forgone when choosing one course of action over another.
- Foregone profits: The potential profits that could have been earned by investing in a different project or asset, instead of the one being evaluated.
- Time value of money: The loss of potential earnings due to the delay in receiving cash flows from the chosen project, compared to the alternative.
- Implicit costs: Expenses that are not explicitly recorded in the financial statements, such as the value of the entrepreneur’s time or resources used in the project.
- Risk premium: The additional return required to compensate for the risk associated with investing in the project, which could have been avoided by choosing a less risky alternative.
Understanding opportunity cost is crucial for accurate NPV calculations. By considering the value of the best alternative that was not taken, financial analysts can make more informed decisions about investment projects and ensure that they are choosing the option that maximizes their overall financial gain.
Excel formulas
Excel formulas play a crucial role in accurately calculating the discount rate for Net Present Value (NPV) in Excel. These formulas automate complex calculations, reduce errors, and simplify financial analysis, making it accessible to a broader range of users.
- Discount rate calculation: Excel provides built-in functions such as RATE and XIRR to calculate the discount rate based on the project’s cash flows.
- Cash flow estimation: Formulas like SUM and AVERAGE can be used to estimate cash flows from historical data or projections.
- Time value of money: Functions like PV and FV help determine the present or future value of cash flows, incorporating the time value of money.
- Scenario analysis: Excel’s powerful scenario manager allows users to explore different combinations of assumptions and their impact on the discount rate and NPV.
By harnessing the capabilities of Excel formulas, financial analysts can efficiently evaluate investment opportunities, make informed decisions, and mitigate risks associated with project cash flows and discount rate calculations.
NPV calculation
Net present value (NPV) calculation is a fundamental aspect of the broader process of determining the discount rate for NPV in Excel. NPV analysis allows businesses to evaluate the profitability of potential investments by considering the time value of money and discounting future cash flows back to the present.
- Cash Flow Projection: Estimating future cash inflows and outflows associated with the investment, considering factors like sales revenue, operating expenses, and capital expenditures.
- Discount Rate Selection: Determining the appropriate discount rate to apply to future cash flows. This rate reflects the cost of capital and the risk associated with the investment.
- Time Value of Money: Incorporating the concept that money available today is worth more than the same amount in the future, due to its potential earning power.
- NPV Calculation: Subtracting the initial investment from the sum of the discounted future cash flows. A positive NPV indicates that the investment is expected to generate a positive return.
These components of NPV calculation are interconnected and play a crucial role in assessing the viability of investment projects. By carefully considering each aspect, businesses can make informed decisions about allocating capital and maximizing their financial performance.
FAQs on Calculating Discount Rate for NPV in Excel
These frequently asked questions provide clear and concise answers to common queries related to calculating the discount rate for Net Present Value (NPV) in Excel. They address key concepts, formula usage, and best practices to help you confidently perform this calculation.
Question 1: What is the discount rate in NPV calculation?
The discount rate represents the cost of capital for the project and is used to determine the present value of future cash flows. It reflects the time value of money and the risk associated with the investment.
Question 2: How do I determine the appropriate discount rate?
Several methods can be used to determine the discount rate, including the Weighted Average Cost of Capital (WACC), Capital Asset Pricing Model (CAPM), and Risk-Adjusted Discount Rate (RADR). The choice of method depends on the project’s specific characteristics and industry.
Question 3: What is the formula for calculating NPV in Excel?
The NPV formula in Excel is: =NPV(discount_rate, cash_flow_range) where discount_rate is the cell reference for the discount rate and cash_flow_range is the range of cells containing the project’s cash flows.
Question 4: How do I handle uneven cash flows in NPV calculations?
To account for uneven cash flows, you can use the XNPV function in Excel, which allows you to specify the timing of each cash flow. Alternatively, you can create a separate row for each cash flow and use the NPV function.
Question 5: What are some best practices for calculating the discount rate?
Best practices include using a range of discount rates to assess the project’s sensitivity, considering inflation and risk when setting the rate, and documenting the assumptions and methodology used in the calculation.
Question 6: What are the limitations of NPV analysis?
While NPV is a valuable tool, it has limitations. It assumes constant cash flows and discount rates, does not consider all project risks, and may not be suitable for complex or long-term projects.
These FAQs provide essential guidance for calculating the discount rate for NPV in Excel. Understanding these concepts and applying them accurately is crucial for making informed investment decisions and evaluating project profitability.
In the next section, we will explore advanced techniques for NPV analysis, including scenario analysis and sensitivity testing, to further enhance the accuracy and reliability of your project evaluations.
Tips for Calculating Discount Rate for NPV in Excel
Accurately calculating the discount rate is crucial for reliable Net Present Value (NPV) analysis. Here are some practical tips to enhance your calculations:
Tip 1: Consider Project Risk: Assess the level of risk associated with the project and incorporate a risk premium into the discount rate to account for potential uncertainties.
Tip 2: Estimate Opportunity Cost: Determine the value of alternative investments that you are forgoing by choosing this project. Include this opportunity cost in your discount rate calculation.
Tip 3: Use Weighted Average Cost of Capital (WACC): For projects funded using a combination of debt and equity, calculate the WACC to represent the overall cost of capital.
Tip 4: Consider Inflation: Adjust the discount rate for expected inflation to ensure that future cash flows are discounted at a rate that reflects the decreasing purchasing power of money.
Tip 5: Test Sensitivity to Discount Rate: Perform scenario analysis by varying the discount rate within a reasonable range to assess the project’s sensitivity to changes in the cost of capital.
Tip 6: Document Assumptions: Clearly document the assumptions and methodology used in your discount rate calculation for transparency and auditability.
Tip 7: Use Excel Functions: Leverage Excel functions like RATE and XIRR to automate discount rate calculations and minimize errors.
Tip 8: Seek Professional Advice: For complex projects or when dealing with significant uncertainties, consider consulting with a financial professional to determine an appropriate discount rate.
By following these tips, you can enhance the accuracy and reliability of your NPV calculations, leading to more informed investment decisions and improved project evaluation.
In the concluding section, we will discuss advanced techniques for NPV analysis, including scenario analysis and sensitivity testing, to further refine your project evaluations and mitigate potential risks.
Conclusion
Calculating the discount rate for Net Present Value (NPV) in Excel is a critical skill for financial analysis. This article provided a comprehensive overview of the concepts, formulas, and best practices involved in this process. By understanding the time value of money, cost of capital, and risk assessment, financial professionals can determine the appropriate discount rate to accurately evaluate investment opportunities.
Key points to remember include:
- The discount rate represents the cost of capital and is used to discount future cash flows to their present value.
- Various methods exist to determine the discount rate, such as the Weighted Average Cost of Capital (WACC) and Risk-Adjusted Discount Rate (RADR).
- Excel provides powerful functions like RATE and XIRR to automate discount rate calculations and enhance accuracy.
Accurately calculating the discount rate is essential for making sound investment decisions. By following the principles outlined in this article, financial analysts can confidently evaluate projects, allocate capital efficiently, and maximize returns. The ability to calculate the discount rate in Excel is a valuable skill that empowers professionals to navigate the complexities of financial analysis and contribute to organizational success.