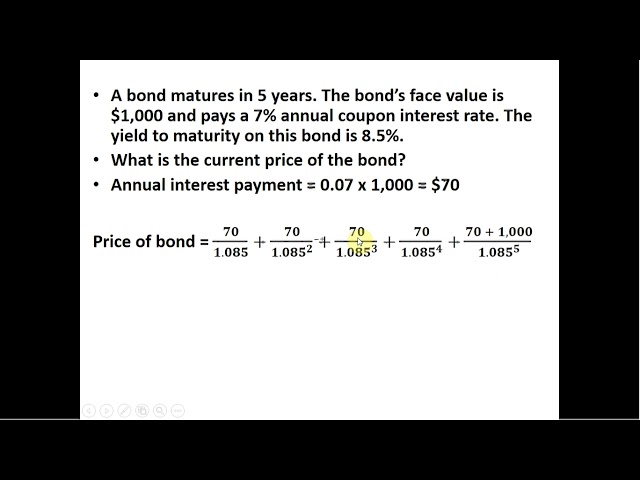
A discount bond formula with example is a mathematical equation used to calculate the present value of a bond that is sold at a price below its face value. For instance, a bond with a $1,000 face value that is sold for $900 is considered a discount bond.
The discount bond formula is significant in finance as it enables investors to determine the potential return on their investment. By understanding the formula and its implications, investors can make informed decisions about purchasing discount bonds.
The formula was first developed in the 19th century by mathematicians and economists who sought to establish a standardized method for valuing bonds. Since then, it has become a cornerstone of bond pricing and is widely used by investment professionals.
Discount Bond Formula with Example
The discount bond formula is a mathematical equation used to calculate the present value of a bond that is sold at a price below its face value. The formula is an essential tool for investors and financial professionals, as it allows them to determine the potential return on their investment. Key aspects of the discount bond formula and its application include:
- Present value
- Face value
- Coupon rate
- Maturity date
- Yield to maturity
- Bond pricing
- Investment analysis
- Portfolio management
- Financial planning
- Risk assessment
Understanding these key aspects is crucial for investors seeking to make informed decisions about purchasing discount bonds. The formula and its implications play a vital role in bond valuation and risk management, enabling investors to maximize their returns and achieve their financial goals.
Present Value
In the context of discount bond formula with example, present value plays a critical role in determining the current worth of a future cash flow. It involves discounting future cash flows back to the present day at a specified rate, known as the yield to maturity. Understanding present value helps investors assess the true value of a bond, especially when it is sold at a discount to its face value.
- Bond Pricing: Present value is used to calculate the fair price of a discount bond by determining the sum of the present values of all future coupon payments and the present value of the face value at maturity.
- Investment Analysis: By comparing the present value of a discount bond to its current market price, investors can gauge its potential return and make informed investment decisions.
- Portfolio Management: Present value analysis aids in constructing bond portfolios that align with specific investment goals and risk tolerance levels.
- Financial Planning: For long-term financial planning, present value helps individuals determine the future value of their investments, considering the effects of inflation and interest rates.
In summary, present value is a crucial aspect of discount bond formula with example. It enables investors to evaluate the present worth of future cash flows, make informed investment choices, and plan for their financial future.
Face Value
In the context of the discount bond formula with example, face value holds significant importance as it represents the nominal value or principal amount of the bond at maturity. It serves as the benchmark against which the bond’s price and yield are determined.
The discount bond formula with example calculates the present value of a bond that is sold below its face value. The difference between the face value and the purchase price represents the discount. By incorporating the face value into the formula, investors can assess the potential return on their investment and make informed decisions about purchasing discount bonds.
For instance, consider a bond with a $1,000 face value that is purchased for $900. The discount of $100 represents the difference between the face value and the purchase price. Using the discount bond formula with example, investors can calculate the yield to maturity and determine the potential return on their investment over the bond’s lifetime.
Understanding the relationship between face value and the discount bond formula with example is crucial for investors seeking to navigate the bond market effectively. By considering the face value, investors can evaluate the potential return and risk associated with discount bonds and make informed investment choices.
Coupon rate
Within the realm of the discount bond formula with example, the coupon rate holds a pivotal position. It represents the annual interest payment made to bondholders, expressed as a percentage of the bond’s face value. Understanding the coupon rate is crucial for investors seeking to evaluate the potential return and risk associated with discount bonds.
- Fixed vs. Floating Rates: Coupon rates can be either fixed or floating. Fixed rates remain constant throughout the bond’s lifetime, while floating rates fluctuate based on prevailing market conditions.
- Relationship to Bond Pricing: Discount bonds with higher coupon rates tend to trade at a premium to their face value, while those with lower coupon rates trade at a discount.
- Impact on Yield to Maturity: The coupon rate significantly influences the yield to maturity of a bond. Bonds with higher coupon rates typically have lower yields to maturity than those with lower coupon rates.
- Implications for Investors: Investors should consider the coupon rate in conjunction with other factors, such as the bond’s face value, maturity date, and current market interest rates, when making investment decisions.
In conclusion, the coupon rate is an integral component of the discount bond formula with example, influencing bond pricing, yield to maturity, and investment decisions. By understanding the different facets and implications of the coupon rate, investors can make informed choices and navigate the bond market effectively.
Maturity date
Within the context of the discount bond formula with example, the maturity date holds significant importance as it represents the specific day on which the bond reaches its final maturity and the principal amount, also known as the face value, becomes due and payable to the bondholder. The maturity date serves as a critical component of the discount bond formula with example, influencing the calculation of the bond’s present value and yield to maturity.
The discount bond formula with example takes into account the time value of money, recognizing that the present value of a future cash flow is less than its face value due to the effects of compounding interest. The maturity date plays a crucial role in determining the length of time over which this discounting process occurs. Bonds with longer maturities have a greater present value discount compared to those with shorter maturities, resulting in a lower purchase price for the bond.
In real-world applications, the maturity date of a discount bond with example significantly impacts investment decisions. Investors must consider the trade-off between potential return and risk when evaluating bonds with different maturity dates. Bonds with longer maturities generally offer higher yields to maturity, but they also expose investors to greater interest rate risk. Conversely, bonds with shorter maturities have lower yields to maturity but carry less interest rate risk.
Understanding the relationship between the maturity date and the discount bond formula with example empowers investors to make informed decisions about bond investments. By considering the time value of money and the impact of maturity dates on bond pricing and yields, investors can effectively manage their portfolios and achieve their financial goals.
Yield to Maturity
Yield to maturity (YTM) is a crucial concept in the realm of the discount bond formula with example. It represents the annualized rate of return an investor can expect to earn if they hold a bond until its maturity date. Understanding YTM is paramount for investors seeking to make informed decisions about bond investments.
- Market Price and YTM: The market price of a discount bond is influenced by its YTM. Bonds with higher YTMs tend to trade at a discount to their face value, while those with lower YTMs trade at a premium.
- Maturity Date Impact: YTM and maturity date are inversely related. Bonds with longer maturities typically have higher YTMs, reflecting the increased risk and time value of money.
- Interest Rate Risk: YTM is sensitive to changes in interest rates. When interest rates rise, YTMs of existing bonds tend to fall, and vice versa.
- Real-World Example: Consider a bond with a $1,000 face value, 5% coupon rate, and 10-year maturity. If the bond is currently trading at $900, its YTM is approximately 6.38%.
In summary, YTM is a critical component of the discount bond formula with example, providing valuable insights into bond pricing, investment returns, and risk management. By understanding the multifaceted aspects of YTM, investors can make informed decisions and navigate the bond market effectively.
Bond pricing
Bond pricing and the discount bond formula with example are inextricably linked, as the formula serves as a fundamental tool for determining the fair price of a bond. The discount bond formula factors in key bond characteristics, such as face value, coupon rate, maturity date, and yield to maturity, to calculate the present value of its future cash flows. This present value directly influences the bond’s market price.
A critical component of the discount bond formula with example, bond pricing helps investors understand the relationship between a bond’s price and its expected return. By calculating the present value of a bond’s future cash flows, investors can assess whether the bond is trading at a discount, at par, or at a premium. This information is vital for making informed investment decisions, as it allows investors to determine the potential return on their investment relative to the market price.
For instance, consider a bond with a $1,000 face value, a 5% coupon rate, and a 10-year maturity. If the current market interest rate is 6%, the discount bond formula with example would calculate a present value of approximately $900. This indicates that the bond is trading at a discount to its face value, offering investors a potential opportunity for a higher return if interest rates remain stable or decline.
Understanding the connection between bond pricing and the discount bond formula with example empowers investors to navigate the bond market effectively. By leveraging this formula, investors can make informed decisions about bond purchases, considering factors such as yield to maturity, credit risk, and market conditions.
Investment analysis
Investment analysis plays a critical role within the framework of the discount bond formula with example. This intricate relationship stems from the fundamental purpose of investment analysis, which lies in evaluating the potential risks and returns associated with various investment options, including bonds. The discount bond formula with example serves as a quantitative tool that aids investors in determining the fair value of a discount bond, incorporating key factors such as present value, yield to maturity, and maturity date.
The discount bond formula with example relies heavily on investment analysis to provide meaningful insights into the bond market. By analyzing factors such as interest rate trends, economic conditions, and the creditworthiness of bond issuers, investors can make informed decisions about the purchase and sale of discount bonds. Moreover, investment analysis enables investors to assess the potential returns and risks associated with different bonds, allowing them to construct diversified portfolios that align with their financial goals and risk tolerance.
In practice, investment analysis is essential for applying the discount bond formula with example effectively. Real-life examples abound, where investors utilize investment analysis to uncover undervalued bonds and make strategic investment decisions. For instance, an investor may analyze the historical performance and financial health of a company issuing discount bonds. By evaluating the company’s revenue growth, debt-to-equity ratio, and industry outlook, the investor can assess the likelihood of the company fulfilling its debt obligations and make an informed decision about investing in the bond.
Portfolio management
Portfolio management and the discount bond formula with example are inextricably linked, with portfolio management serving as a critical component of effective bond investment strategies. The discount bond formula, by calculating the present value of a bond’s future cash flows, provides a fundamental basis for evaluating the potential risks and returns associated with discount bonds. This understanding empowers portfolio managers to make informed decisions when constructing and managing bond portfolios.
In practice, portfolio managers utilize the discount bond formula with example to determine the fair value of discount bonds, enabling them to identify undervalued opportunities and optimize portfolio returns. By incorporating factors such as yield to maturity, coupon payments, and maturity date, the formula helps portfolio managers assess the impact of interest rate fluctuations and market conditions on bond prices. This analysis allows them to construct diversified portfolios that align with specific investment objectives and risk tolerance levels.
For example, a portfolio manager seeking to reduce interest rate risk may choose to allocate a portion of the portfolio to discount bonds with shorter maturities. Conversely, a portfolio manager with a higher risk tolerance may invest in discount bonds with longer maturities, potentially offering higher returns but also exposing the portfolio to greater interest rate risk. The discount bond formula with example provides the necessary framework for making these strategic asset allocation decisions.
In summary, the discount bond formula with example serves as a foundational tool for portfolio management, enabling investors to analyze and value discount bonds, construct diversified portfolios, and make informed investment decisions. By leveraging this formula, portfolio managers can navigate the bond market effectively, optimize returns, and mitigate risks, ultimately achieving their clients’ financial goals.
Financial planning
Financial planning holds a pivotal role in the context of the discount bond formula with example. It serves as a comprehensive framework for individuals and organizations to develop and execute strategies for achieving their financial goals. The discount bond formula, on the other hand, is a mathematical equation used to calculate the present value of a bond that is sold below its face value. Understanding the intricate relationship between these two concepts is paramount for effective financial planning and investment decision-making.
Financial planning provides a structured approach to evaluate an individual’s or organization’s financial situation, considering factors such as income, expenses, assets, liabilities, and risk tolerance. This assessment forms the foundation for making informed decisions about saving, investing, and managing debt. The discount bond formula with example plays a crucial role in this process, as it enables individuals to determine the fair value of discount bonds and assess their potential return on investment.
Real-life examples abound where financial planning incorporates the discount bond formula with example. For instance, individuals planning for retirement may utilize the formula to calculate the present value of their future retirement savings, considering factors such as their desired retirement income and life expectancy. Similarly, organizations may use the formula to evaluate the present value of future cash flows from capital projects or investments, aiding in decision-making and risk management.
The practical applications of understanding the connection between financial planning and the discount bond formula with example are far-reaching. It empowers individuals and organizations to make informed investment decisions, plan for future financial needs, and mitigate risks. By integrating the formula into financial planning models, individuals can optimize their savings strategies, accumulate wealth, and achieve their long-term financial objectives.
Risk assessment
Risk assessment and the discount bond formula with example are inextricably linked, with risk assessment forming a critical component of the formula’s application and interpretation. The discount bond formula with example calculates the present value of a bond that is sold below its face value, considering factors such as the bond’s coupon rate, maturity date, and yield to maturity. Risk assessment plays a crucial role in determining the appropriate yield to maturity, which is a fundamental input to the formula.
Understanding the relationship between risk assessment and the discount bond formula with example is essential for investors and financial professionals seeking to make informed investment decisions. The yield to maturity, which is used to discount future cash flows back to the present value, is influenced by various risk factors, including credit risk, interest rate risk, and liquidity risk. A thorough risk assessment helps investors gauge the potential risks associated with a bond and determine an appropriate yield to maturity that reflects the level of risk they are willing to take.
In practice, investors often utilize credit ratings assigned by credit rating agencies to assess the creditworthiness of bond issuers. These ratings provide an indication of the likelihood that the issuer will default on its debt obligations, which in turn affects the yield to maturity demanded by investors. Additionally, investors may consider factors such as the issuer’s financial performance, industry outlook, and economic conditions to conduct a comprehensive risk assessment.
The practical significance of understanding the connection between risk assessment and the discount bond formula with example extends to various financial applications. For instance, portfolio managers use the formula to calculate the present value of bonds and construct diversified portfolios that balance risk and return. Corporate treasurers may employ the formula to evaluate the cost of debt financing and make informed decisions about capital structure. Moreover, the formula is widely used by financial analysts to assess the fair value of bonds and provide investment recommendations.
Frequently Asked Questions (FAQs) on Discount Bond Formula with Example
This section addresses common questions and clarifications related to the discount bond formula with example. These FAQs aim to provide a deeper understanding of the formula’s application and implications in various financial scenarios.
Question 1: What is the difference between a discount bond and a premium bond?
A discount bond is sold below its face value, while a premium bond is sold above its face value. The discount or premium reflects the difference between the bond’s market price and its face value.
Question 2: How does the yield to maturity affect the discount bond formula?
The yield to maturity (YTM) is a crucial input in the discount bond formula. A higher YTM results in a lower present value for the bond, making it more attractive to investors.
Question 3: What is the significance of the maturity date in the discount bond formula?
The maturity date determines the duration of the bond and influences its present value. Bonds with longer maturities have a lower present value due to the time value of money.
Question 4: How can the discount bond formula be used in investment analysis?
The discount bond formula helps investors assess the potential return and risk associated with discount bonds. By calculating the present value, investors can determine the bond’s fair price and make informed investment decisions.
Question 5: What are the practical applications of the discount bond formula in the financial industry?
The discount bond formula is widely used by portfolio managers, corporate treasurers, and financial analysts to value bonds, construct portfolios, and make investment recommendations.
Question 6: How can investors incorporate the discount bond formula into their financial planning?
Individuals can use the discount bond formula to calculate the present value of their future retirement savings, considering factors such as their desired retirement income and life expectancy.
In summary, these FAQs provide a comprehensive overview of the discount bond formula with example, its applications, and its significance in investment decision-making. Understanding these concepts empowers investors and financial professionals to navigate the bond market effectively.
Building upon this understanding, the next section will delve deeper into advanced applications of the discount bond formula, exploring its use in complex financial modeling and risk management strategies.
Tips to Enhance Your Understanding of the Discount Bond Formula with Example
This section provides practical tips to help you master the discount bond formula with example. By implementing these tips, you can refine your bond valuation skills and make more informed investment decisions.
Tip 1: Understand the Fundamentals: Begin by grasping the core concepts of bond pricing, present value, and yield to maturity. A solid foundation will enhance your comprehension of the formula and its applications.
Tip 2: Practice Regularly: Engage in regular practice using the discount bond formula with example. Apply it to various scenarios and bond characteristics to develop proficiency and build confidence.
Tip 3: Consider Real-World Factors: When applying the formula, incorporate real-world factors that influence bond prices, such as interest rate changes, credit risk, and market conditions. This will provide a more realistic understanding of bond valuation.
Tip 4: Use Financial Calculators: Leverage financial calculators or online tools to simplify your calculations and save time. However, ensure you understand the underlying principles to avoid errors.
Tip 5: Analyze Bond Ratings: Incorporate bond ratings into your analysis to assess the creditworthiness of bond issuers. This will help you determine appropriate yield to maturity rates and make informed decisions.
Tip 6: Monitor Market Trends: Keep abreast of market trends and economic news that may impact bond prices. This will provide context for your analysis and help you anticipate potential changes.
Tip 7: Consult with Experts: If you encounter complexities or have specific questions, consider consulting with financial advisors or experienced investors. Their insights can supplement your understanding.
Tip 8: Stay Updated: The financial landscape is constantly evolving. Stay informed about new developments and best practices related to bond valuation to maintain your expertise.
Implementing these tips will significantly enhance your understanding and application of the discount bond formula with example. By mastering this formula, you can effectively evaluate discount bonds, make informed investment decisions, and navigate the bond market with confidence.
Building upon these tips, the concluding section will highlight the broader implications of the discount bond formula with example, exploring its significance in portfolio management, risk assessment, and financial planning.
Conclusion
In summary, the exploration of “discount bond formula with example” provides valuable insights for navigating the bond market effectively. Understanding the formula and its components, including present value, yield to maturity, and maturity date, is essential for informed decision-making.
Key ideas highlighted in this article include:
- The discount bond formula with example serves as a fundamental tool for calculating the present value of bonds sold below their face value.
- The formula incorporates real-world factors such as interest rate changes, credit risk, and market conditions, making it a practical tool for bond valuation.
- The discount bond formula with example finds extensive applications in portfolio management, risk assessment, and financial planning, empowering investors to make strategic investment decisions.
Understanding and applying the discount bond formula with example empowers investors and financial professionals to assess the potential return and risk associated with discount bonds, ultimately contributing to successful investment outcomes. As the financial landscape continues to evolve, staying abreast of advancements in bond valuation techniques is crucial for investors seeking to maximize their returns and achieve their financial goals.