Calculating zero coupon bond prices is a fundamental skill in finance. A zero-coupon bond is a type of fixed-income security that does not pay periodic interest payments (coupons) but instead is sold at a deep discount to its face value. Investors make a profit when the bond matures and is redeemed at full value.
Valuing zero-coupon bonds requires careful consideration of factors such as the bond’s maturity date, the prevailing interest rates, and the bond’s face value. By accurately calculating the present value of the bond’s future cash flows, investors can make informed decisions about whether to buy, sell, or hold zero-coupon bonds.
Understanding how to calculate zero coupon bond prices is essential for financial analysts, portfolio managers, and anyone interested in fixed-income investing. In this article, we will thoroughly examine the steps and formulas involved in determining the price of a zero-coupon bond using Microsoft Excel, a widely accessible and versatile software tool.
How to Calculate Zero Coupon Bond Price in Excel
Calculating zero coupon bond prices in Excel involves considering several key aspects:
- Face value
- Maturity date
- Yield to maturity
- Present value
- Excel functions (PV, FV, RATE)
- Time value of money
- Bond pricing models
- Financial calculators
- Market conditions
These aspects are interconnected and influence the accuracy of the calculated bond price. Understanding their relationships is essential for effective bond valuation. For instance, the present value of a bond’s future cash flows is directly affected by the yield to maturity and the time remaining until maturity. Excel functions like PV and FV simplify the calculation process, but it’s crucial to apply them correctly based on the bond’s characteristics and market conditions.
Face value
In the context of zero-coupon bond pricing in Excel, the face value holds significant importance. It represents the amount an investor will receive upon the bond’s maturity, making it a fundamental component of the calculation.
- Bond principal
The face value is the principal amount borrowed by the bond issuer and repaid to the investor at maturity. - Maturity price
Upon maturity, the bond is redeemed at its face value, which represents the bond’s maturity price. - Present value calculation
The face value is a crucial input in calculating the present value of the bond’s future cash flows, which determines its current market price. - Excel functions
Excel functions like PV and FV utilize the face value to compute the bond’s present value and future value, respectively.
Understanding the face value and its implications is essential for accurate bond pricing in Excel. It serves as the benchmark against which the bond’s market value is assessed and helps investors make informed decisions about bond purchases and sales.
Maturity date
In the realm of bond pricing, the maturity date holds immense significance. It marks the predetermined date on which a bond reaches its maturity, culminating in the repayment of the principal amount to the investor. Understanding the nuances of the maturity date is crucial for accurate bond valuation using Excel.
- Bond duration
The maturity date directly influences a bond’s duration, which measures the sensitivity of its price to changes in interest rates. - Yield to maturity
The yield to maturity (YTM) is calculated based on the bond’s price, maturity date, and coupon payments. It represents the annualized rate of return an investor can expect to earn if they hold the bond until maturity. - Present value
The present value of a bond’s future cash flows is heavily dependent on the maturity date. Bonds with longer maturities typically have lower present values due to the time value of money. - Excel functions
Excel functions like DATE and EDATE are commonly used to calculate maturity dates based on specified parameters like issue date and term.
Comprehending the maturity date and its multifaceted implications empowers investors and analysts to make informed decisions. It enables them to assess the potential risks and rewards associated with bond investments and optimize their portfolios accordingly.
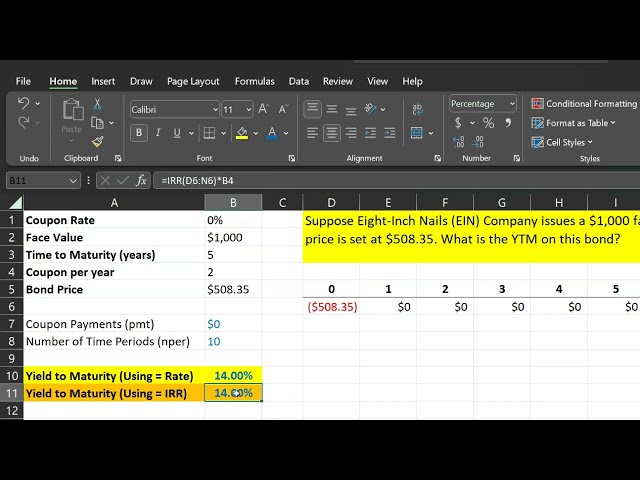
Yield to maturity
Yield to maturity (YTM) plays a pivotal role in determining the price of zero-coupon bonds in Excel. It represents the annualized rate of return an investor can expect to earn if they hold the bond until maturity. The YTM is a critical component of the pricing calculation, as it directly influences the present value of the bond’s future cash flows.
In Excel, the YTM is used in conjunction with the PV function to calculate the bond’s price. The PV function takes into account the bond’s face value, maturity date, and YTM to determine its present value. A higher YTM will result in a lower present value, and vice versa. This inverse relationship is due to the time value of money, which states that the present value of a future cash flow decreases as the required rate of return (YTM) increases.
Real-life examples further illustrate the connection between YTM and zero-coupon bond pricing in Excel. Consider a zero-coupon bond with a face value of $1,000 and a maturity date of 5 years. If the prevailing YTM is 5%, the bond’s price, as calculated using the PV function in Excel, would be approximately $783.50. However, if the YTM were to increase to 7%, the bond’s price would decrease to approximately $746.20. This demonstrates how changes in YTM can significantly impact the price of a zero-coupon bond.
Understanding the relationship between YTM and zero-coupon bond pricing in Excel is essential for investors and analysts. It enables them to accurately value bonds, assess their potential returns, and make informed investment decisions. By utilizing Excel’s powerful functions and formulas, investors can efficiently calculate bond prices and navigate the complexities of fixed-income investing.
Present value
In the realm of fixed-income investing, understanding the concept of present value is crucial for accurately pricing zero-coupon bonds using Excel. Present value represents the current worth of a future sum of money, taking into account the time value of money and the prevailing interest rates.
Calculating the present value of a zero-coupon bond’s future cash flows is a critical component of determining its price in Excel. The present value is directly influenced by the bond’s face value, maturity date, and the yield to maturity (YTM). A higher YTM will result in a lower present value due to the inverse relationship between interest rates and present value. Excel’s PV function is commonly used to calculate the present value, incorporating these parameters into its formula.
Real-life examples further illustrate the significance of present value in zero-coupon bond pricing. Consider a zero-coupon bond with a face value of $1,000 and a maturity of 5 years. If the prevailing YTM is 5%, the bond’s present value, as calculated using the PV function in Excel, would be approximately $783.50. This present value represents the current worth of the future $1,000 payment, discounted at a rate of 5% per year over the 5-year period.
Understanding the relationship between present value and zero-coupon bond pricing in Excel empowers investors to make informed investment decisions. By accurately calculating the present value, investors can assess the fair value of the bond, compare it to market prices, and determine whether it is an attractive investment opportunity. Furthermore, this understanding enables investors to manage interest rate risk and optimize their fixed-income portfolios.
Excel functions (PV, FV, RATE)
In practical terms, Excel functions, particularly PV, FV, and RATE, form the backbone of zero coupon bond pricing calculations in Excel. These functions provide a convenient and accurate means of determining the present value, future value, and yield to maturity of a zero-coupon bond, respectively. Their seamless integration into Excel’s formulaic environment empowers analysts and investors to swiftly and efficiently value bonds, enabling informed investment decisions.
The PV function, in particular, plays a pivotal role in calculating the present value of a zero-coupon bond’s future cash flows. By incorporating the bond’s face value, maturity date, and yield to maturity as inputs, the PV function discounts the future payment back to the present, providing a precise assessment of the bond’s current worth. This present value serves as a fundamental input for determining the bond’s market price.
Real-life examples further underscore the critical importance of Excel functions in zero-coupon bond pricing. Consider an investor seeking to purchase a zero-coupon bond with a face value of $1,000 and a maturity of 5 years. Using the prevailing yield to maturity of 5%, the investor can employ the PV function in Excel to calculate the bond’s present value, which equates to approximately $783.50. This present value represents the current worth of the future $1,000 payment, discounted at the rate of 5% per year over the 5-year period.
Understanding the connection between Excel functions (PV, FV, RATE) and the calculation of zero-coupon bond prices in Excel is a key aspect of fixed-income analysis. By leveraging these functions, investors can accurately value bonds, assess potential returns, and make prudent investment decisions. Furthermore, this understanding enables investors to manage interest rate risk and optimize their fixed-income portfolios.
Time value of money
When calculating zero coupon bond prices in Excel, understanding the concept of time value of money (TVM) is crucial. TVM recognizes that the value of money today is different from its value in the future due to its earning potential. This concept forms the foundation for accurate bond pricing and investment decision-making.
- Present vs. future value
TVM acknowledges that a dollar received today is worth more than a dollar received in the future due to the potential for growth through investment. - Discounting
When valuing future cash flows, TVM employs discounting to determine their present value, considering the time value of money and the prevailing interest rates. - Compound interest
TVM incorporates the effect of compound interest, where interest is earned not only on the principal but also on the accumulated interest, leading to exponential growth over time. - Annuity
TVM is applied in calculating the present value or future value of a series of equal cash flows occurring at regular intervals, such as bond coupon payments or regular savings contributions.
In the context of zero-coupon bond pricing in Excel, TVM plays a critical role in determining the present value of the bond’s future cash flow, which is the sole payment received at maturity. By considering the time value of money, investors can accurately assess the bond’s current worth and make informed investment decisions based on its potential return and risk profile.
Bond pricing models
In the realm of fixed-income investing, bond pricing models serve as essential tools for determining the fair value of bonds, including zero-coupon bonds. These models incorporate a range of factors to assess the bond’s risk and return profile, enabling investors to make informed investment decisions.
- Discounted cash flow (DCF) models
DCF models discount the bond’s future cash flows back to the present using a specified discount rate, typically the yield to maturity. This approach provides a straightforward and widely used method for bond valuation. - Structural models
Structural models employ a more complex approach, considering the underlying economic factors that influence bond prices, such as interest rates, inflation, and credit risk. These models require specialized knowledge and data but can provide a more accurate valuation under certain market conditions. - Credit risk models
Credit risk models assess the likelihood of a bond issuer defaulting on its obligations. By incorporating factors such as the issuer’s financial health, industry trends, and economic conditions, these models help investors quantify the risk associated with investing in a particular bond. - Prepayment models
Prepayment models are specifically designed for mortgage-backed securities, which carry the risk of being prepaid before maturity. These models incorporate factors such as interest rate movements, housing market conditions, and prepayment penalties to estimate the probability and timing of prepayments, which can significantly impact bond pricing.
Understanding these bond pricing models is crucial for accurately calculating zero coupon bond prices in Excel. By selecting the appropriate model based on the bond’s characteristics and market conditions, investors can enhance the precision of their valuations and make informed investment decisions.
Financial calculators
Financial calculators play a critical role in the practical application of “how to calculate zero coupon bond price in excel”. They provide a convenient and portable means of performing complex financial calculations, including bond pricing, which can be particularly useful when working with zero-coupon bonds due to their unique characteristics.
The connection between financial calculators and “how to calculate zero coupon bond price in excel” is evident in several ways. Firstly, financial calculators are designed to handle the intricate calculations involved in bond pricing, incorporating formulas and functions that account for factors such as present value, yield to maturity, and time value of money. These calculators can quickly and accurately determine the price of a zero-coupon bond, allowing investors to make informed decisions about buying, selling, or holding such bonds.
Moreover, financial calculators provide real-time results, enabling investors to assess the impact of changing market conditions on bond prices. This is particularly valuable in the case of zero-coupon bonds, which are highly sensitive to interest rate fluctuations. By using a financial calculator, investors can adjust the yield to maturity and observe the corresponding changes in bond price, allowing them to make timely and appropriate adjustments to their investment strategies.
In summary, financial calculators are essential tools for calculating zero coupon bond prices in excel and are widely used by investors, analysts, and financial professionals. Their ability to perform complex calculations quickly and accurately, coupled with their portability and ease of use, makes them indispensable for anyone seeking to make informed decisions in the fixed-income market.
Market conditions
In the context of fixed-income investing, market conditions play a pivotal role in shaping the prices of zero-coupon bonds. These conditions encompass a wide range of factors that influence the overall investment landscape, directly affecting the calculations used to determine zero-coupon bond prices in Excel.
One critical connection between market conditions and zero-coupon bond pricing lies in the relationship between interest rates and bond prices. Zero-coupon bonds are particularly sensitive to interest rate fluctuations due to the absence of periodic coupon payments. When interest rates rise, the present value of future cash flows decreases, leading to a decline in zero-coupon bond prices. Conversely, falling interest rates result in higher bond prices as the present value of future cash flows increases.
Real-life examples further illustrate this connection. During periods of economic growth and rising interest rates, investors may sell their zero-coupon bonds to lock in profits or seek higher returns elsewhere. This increased supply of bonds on the market can drive down prices. Conversely, in times of economic slowdown and falling interest rates, investors may seek the safety and stability of zero-coupon bonds, leading to increased demand and higher prices.
Understanding the impact of market conditions on zero-coupon bond pricing is crucial for informed decision-making. Investors need to consider the prevailing interest rate environment, economic outlook, and market sentiment when calculating bond prices in Excel. This understanding empowers them to make appropriate adjustments to their investment strategies, such as buying or selling bonds at opportune moments to maximize returns or mitigate risks.
Frequently Asked Questions
This FAQ section addresses common questions and clarifies aspects of “how to calculate zero coupon bond price in excel” to enhance understanding and practical application.
Question 1: What is the primary factor influencing zero-coupon bond prices?
Answer: Interest rates play a crucial role, as zero-coupon bonds are highly sensitive to changes in interest rates due to the absence of periodic coupon payments.
Question 2: How do I incorporate the yield to maturity into my Excel calculations?
Answer: Utilize the YIELD function in Excel, which calculates the yield to maturity based on the bond’s price, maturity date, and number of payments per year.
Question 3: Can I use Excel to determine the present value of a zero-coupon bond’s future cash flow?
Answer: Yes, employ the PV function in Excel, which calculates the present value by considering the face value, maturity date, and yield to maturity.
Question 4: How do I account for time value of money in my calculations?
Answer: Excel functions like PV and FV incorporate time value of money by discounting future cash flows back to the present, considering the prevailing interest rates.
Question 5: What if I want to assess the impact of different interest rate scenarios on bond prices?
Answer: Use Excel’s scenario manager to create multiple scenarios with varying interest rates and observe the corresponding changes in bond prices.
Question 6: Are there any built-in Excel templates or add-ins specifically designed for zero-coupon bond pricing?
Answer: Yes, search for templates or add-ins within Excel that cater to fixed-income analysis, including zero-coupon bond pricing calculations.
These FAQs provide valuable insights into the key considerations and practical steps involved in calculating zero coupon bond prices in Excel. Understanding these concepts empowers investors and analysts to make informed investment decisions in the fixed-income market.
In the next section, we will delve deeper into advanced techniques and strategies for zero-coupon bond pricing, exploring more complex scenarios and incorporating additional factors that influence bond valuations.
Tips for Calculating Zero Coupon Bond Prices in Excel
To enhance your understanding and practical application of zero coupon bond pricing in Excel, here are several actionable tips:
Tip 1: Familiarize Yourself with Excel Functions: Master the functions essential for bond pricing, such as PV, FV, RATE, and YIELD, to accurately calculate present value, future value, and yield to maturity.
Tip 2: Consider Time Value of Money: Recognize the impact of time value of money on future cash flows, as it affects the present value calculations that determine bond prices.
Tip 3: Utilize Financial Calculators: Leverage financial calculators to supplement your Excel calculations, providing quick and portable access to bond pricing functions.
Tip 4: Monitor Market Conditions: Stay informed about prevailing interest rates and economic conditions, as they significantly influence zero-coupon bond prices.
Tip 5: Explore Bond Pricing Models: Familiarize yourself with different bond pricing models, such as DCF and structural models, to enhance the accuracy of your calculations under varying market scenarios.
Tip 6: Practice with Real-World Examples: Apply your knowledge and skills to real-life bond pricing scenarios to develop a practical understanding of the concepts.
Tip 7: Seek Professional Guidance: If needed, consult with financial professionals or experts to gain additional insights and guidance on complex bond pricing situations.
Tip 8: Stay Updated on Market Developments: Keep abreast of the latest advancements in Excel functions and bond pricing techniques to ensure your knowledge remains current.
By implementing these tips, you can refine your Excel skills for zero coupon bond pricing, leading to more informed investment decisions and a deeper understanding of fixed-income markets.
In the next section, we will explore advanced strategies for zero-coupon bond pricing, delving into the intricacies of complex scenarios and incorporating additional factors that influence bond valuations.
Conclusion
This comprehensive guide has delved into the intricacies of “how to calculate zero coupon bond price in excel,” providing a thorough understanding of the key concepts, formulas, and techniques involved. We have explored the impact of factors such as face value, maturity date, yield to maturity, present value, and time value of money, highlighting their interconnections and significance in determining bond prices.
Two main points stand out: Firstly, understanding the relationship between present value and yield to maturity is crucial, as changes in one directly affect the other. Secondly, incorporating market conditions into calculations is essential, as interest rate fluctuations and economic outlook can significantly influence zero-coupon bond prices. By mastering these concepts and applying them effectively, investors can accurately value zero-coupon bonds and make informed investment decisions.
In today’s dynamic financial landscape, the ability to calculate zero coupon bond prices in Excel is an invaluable skill for investors seeking to navigate the fixed-income market with confidence. Equipping oneself with this knowledge empowers individuals to assess bond values, compare investment options, and optimize their portfolios for desired risk and return profiles.