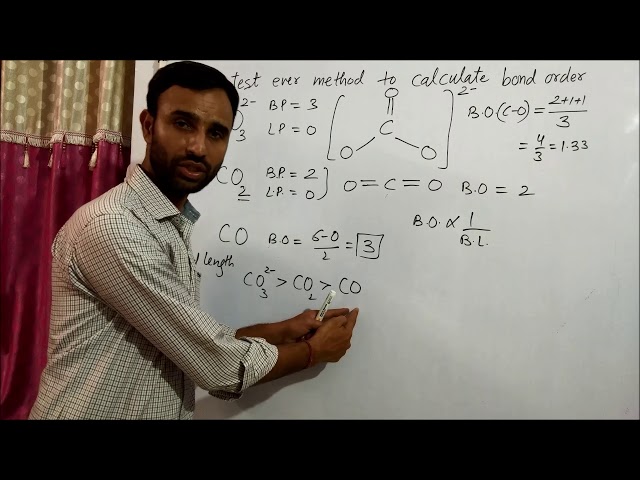
Understanding bond order is crucial in chemistry, as it determines the strength and stability of chemical bonds. Calculating bond orders in polyatomic molecules, which contain more than two atoms, requires a systematic approach.
Bond order provides insights into molecular properties, reactivity, and electronic structure. It finds applications in various fields, including materials science, drug design, and atmospheric chemistry. The concept of bond order originated with Linus Pauling’s groundbreaking work on valence bond theory in the 1930s.
This article delves into the methods for calculating bond orders in polyatomic molecules, covering both qualitative and quantitative approaches. We will explore resonance theory, molecular orbital theory, and experimental techniques to determine bond orders and gain a deeper understanding of molecular bonding.
How to Calculate Bond Order of Polyatomic Molecules
Understanding how to calculate bond order in polyatomic molecules is crucial for comprehending their chemical bonding and properties. Several key aspects are involved in this calculation:
- Molecular orbital theory
- Valence bond theory
- Resonance
- Electron configuration
- Hybridization
- Delocalization
- Formal charge
- Oxidation state
- Quantum mechanics
These aspects provide a comprehensive framework for determining bond order, considering factors such as the overlap of atomic orbitals, electron distribution, and the nature of the chemical bond. By understanding these key aspects, chemists can gain valuable insights into the electronic structure and reactivity of polyatomic molecules.
Molecular Orbital Theory
Molecular orbital theory provides a theoretical framework for understanding the electronic structure of molecules, offering valuable insights into bond formation, properties, and reactivity. This theory is particularly useful for calculating bond orders in polyatomic molecules, where multiple atoms and complex bonding patterns exist.
- Electron Configuration: Molecular orbital theory considers the electron configuration of atoms involved in bonding, enabling the determination of the number and type of molecular orbitals formed.
- Orbital Overlap: It emphasizes the overlap of atomic orbitals, which leads to the formation of molecular orbitals. The extent of overlap influences the strength and type of chemical bond.
- Bonding and Antibonding Orbitals: The theory distinguishes between bonding orbitals, which stabilize the molecule, and antibonding orbitals, which destabilize it. The number of bonding and antibonding orbitals determines the bond order.
- Delocalization: Molecular orbital theory accounts for the delocalization of electrons across multiple atoms, allowing for the formation of resonance structures and contributing to the overall stability of the molecule.
In summary, molecular orbital theory provides a comprehensive understanding of the electronic structure of polyatomic molecules. By considering electron configurations, orbital overlap, bonding and antibonding orbitals, and delocalization, this theory offers a powerful tool for calculating bond orders and gaining insights into molecular properties and bonding.
Valence Bond Theory
Valence bond theory (VBT) is a fundamental concept used to calculate bond order in polyatomic molecules. It focuses on the interactions between atomic orbitals to form covalent bonds, providing a localized perspective on molecular bonding.
- Hybridization: VBT explains how atomic orbitals combine to form hybrid orbitals with specific geometries. This hybridization influences the bond angles and strengths in polyatomic molecules.
- Overlap: VBT emphasizes the overlap of atomic orbitals as the driving force behind bond formation. The extent of overlap determines the bond order and strength.
- Resonance: VBT recognizes that some molecules can exist as resonance hybrids, where multiple Lewis structures contribute to the overall bonding. Resonance affects bond order calculations.
- Formal Charge: VBT assigns formal charges to atoms in a molecule to determine their potential contribution to bond formation. Formal charges aid in predicting bond order.
By considering these aspects of VBT, chemists can gain insights into the electronic structure and bonding of polyatomic molecules. VBT complements molecular orbital theory, providing an alternative perspective on bond order calculations and molecular properties.
Resonance
Resonance is a crucial aspect in understanding how to calculate bond order of polyatomic molecules. It provides a means to represent the delocalization of electrons within a molecule, offering a deeper insight into its electronic structure and bonding.
- Contributing Structures: Resonance involves the use of multiple Lewis structures, known as contributing structures, to describe a molecule. These structures differ in the placement of double bonds and lone pairs, but they all have the same overall connectivity of atoms.
- Delocalized Electrons: Resonance implies that certain electrons in a molecule are not confined to a specific bond or atom but are rather spread out over a larger region of the molecule. This delocalization of electrons contributes to the stability of the molecule.
- Equivalent Resonance Structures: The contributing structures used in resonance are not equally important. Some structures may contribute more significantly to the overall resonance hybrid than others. The more equivalent the contributing structures, the more stable the resonance hybrid.
- Impact on Bond Order: Resonance affects the bond order of a molecule. In resonance hybrids, the bond order of a particular bond is an average of the bond orders in the contributing structures.
By considering resonance, chemists can gain a more accurate representation of the electronic structure of polyatomic molecules. Resonance provides valuable insights into molecular properties, stability, and reactivity, and it plays a significant role in determining bond orders.
Electron Configuration
Electron configuration plays a pivotal role in calculating bond order of polyatomic molecules. It provides insights into the arrangement and behavior of electrons within a molecule, which directly influences the formation and strength of chemical bonds.
- Atomic Orbitals: Electron configuration describes the distribution of electrons in atomic orbitals, which serve as the building blocks for molecular orbitals in polyatomic molecules.
- Valence Electrons: Valence electrons, the outermost electrons in an atom, are primarily involved in chemical bonding. Electron configuration helps identify the number and type of valence electrons available for bond formation.
- Molecular Orbital Formation: The overlap of atomic orbitals, guided by electron configuration, leads to the formation of molecular orbitals. The number and symmetry of these molecular orbitals determine the bond order and properties of the molecule.
- Bonding and Antibonding Orbitals: Electron configuration influences the formation of bonding and antibonding molecular orbitals. Bonding orbitals promote bond formation, while antibonding orbitals weaken or prevent it.
Understanding electron configuration allows chemists to predict the electronic structure and bonding characteristics of polyatomic molecules. It provides a foundation for calculating bond orders, explaining molecular properties, and predicting chemical reactivity.
Hybridization
Hybridization, within the realm of calculating bond order of polyatomic molecules, offers a deeper understanding of the electronic structure and molecular geometry. It involves the intermixing of atomic orbitals to form new hybrid orbitals with specific shapes and orientations.
- sp3 Hybridization: Involves the mixing of one s orbital with three p orbitals, resulting in four equivalent hybrid orbitals with tetrahedral geometry. Example: Methane (CH4).
- sp2 Hybridization: Combines one s orbital with two p orbitals, forming three hybrid orbitals with trigonal planar geometry. Example: Ethylene (C2H4).
- sp Hybridization: Mixes one s orbital with one p orbital, resulting in two hybrid orbitals with linear geometry. Example: Acetylene (C2H2).
- dsp3 Hybridization: Combines one d orbital, one s orbital, and three p orbitals, forming five hybrid orbitals with trigonal bipyramidal geometry. Example: Phosphorus pentachloride (PCl5).
By considering hybridization, chemists can explain the geometries, bond angles, and relative energies of molecular orbitals in polyatomic molecules. Hybrid orbitals provide a framework for understanding the formation and properties of chemical bonds, making hybridization a crucial aspect in calculating bond order and comprehending the electronic structure of these molecules.
Delocalization
Within the realm of calculating bond order of polyatomic molecules, the concept of delocalization holds significant importance. Delocalization refers to the spreading out of electrons over multiple atoms, rather than their confinement to a specific bond or atom. This phenomenon arises due to the resonance between different Lewis structures, leading to a more stable resonance hybrid.
In calculating bond order, delocalization plays a critical role. It affects the distribution of electrons within the molecule, influencing the strength and character of the bonds. Delocalized electrons contribute to the overall stability of the molecule by lowering its energy. A classic example of delocalization is benzene, where the electrons in the pi system are delocalized over the entire ring, resulting in resonance and increased stability.
Understanding delocalization is crucial for accurately calculating bond order in polyatomic molecules. It provides insights into the electronic structure, molecular properties, and reactivity of these molecules. Delocalization can alter bond lengths, bond strengths, and molecular geometry, impacting the overall behavior and functionality of the molecule. In fields such as organic chemistry and materials science, understanding delocalization is essential for designing and synthesizing molecules with specific properties.
Formal charge
In the context of calculating bond order of polyatomic molecules, formal charge plays a significant role. Formal charge is a conceptual tool used to assess the charge distribution within a molecule, providing insights into its electronic structure and bonding characteristics. Understanding formal charge is crucial for accurately determining bond order, which is a measure of the strength and nature of the chemical bonds within a molecule.
The formal charge of an atom in a molecule is calculated by considering the number of valence electrons it has in the free state, the number of lone pair electrons it possesses, and the number of electrons involved in bonds with neighboring atoms. The difference between these values gives the formal charge. Formal charge helps identify atoms with a positive or negative charge imbalance, which can influence bond polarity and overall molecular properties.
Real-life examples of formal charge in calculating bond order include:
- In the water molecule (H2O), oxygen has a formal charge of -1, indicating a slight negative charge on the atom.
- In the carbon dioxide molecule (CO2), carbon has a formal charge of +2, representing a positive charge on the atom.
Understanding formal charge allows chemists to predict and rationalize the electronic structure and bonding in polyatomic molecules, enabling them to design and synthesize molecules with desired properties for various applications.
In summary, formal charge is an essential concept in calculating bond order of polyatomic molecules. It provides valuable insights into the charge distribution within the molecule, aiding in the comprehension of its electronic structure and bonding characteristics. This understanding has practical applications in diverse fields, including molecular modeling, drug design, and materials science.
Oxidation state
In the context of calculating bond order of polyatomic molecules, understanding oxidation state is crucial. It provides insights into the distribution of charges within the molecule, allowing for a deeper comprehension of its electronic structure and chemical bonding. Oxidation state, often referred to as oxidation number, is a measure of the hypothetical charge an atom would have if all bonds to neighboring atoms were completely ionic.
- Definition and Calculation: Oxidation state is assigned to each atom in a molecule based on the assumption that all bonds are ionic. It considers the electronegativity of the atoms involved and the number of electrons gained or lost during bond formation.
- Real-life Examples: In water (H2O), oxygen has an oxidation state of -2, while each hydrogen has an oxidation state of +1. In sodium chloride (NaCl), sodium has an oxidation state of +1, and chlorine has an oxidation state of -1.
- Bond Order Determination: Oxidation states aid in determining bond order by providing information about the charge distribution within the molecule. A higher difference in oxidation states between bonded atoms typically indicates a higher bond order.
- Redox Reactions: Oxidation state changes are central to redox reactions, where electrons are transferred between atoms or ions. Understanding oxidation states helps identify the species undergoing oxidation or reduction and determine the direction of electron flow.
In summary, oxidation state plays a vital role in calculating bond order of polyatomic molecules. It offers insights into charge distribution, aids in bond order determination, and facilitates the analysis of redox reactions. By considering oxidation states, chemists gain a more comprehensive understanding of the electronic structure and chemical bonding within complex molecules, enabling accurate predictions of their properties and reactivities.
Quantum Mechanics
Quantum mechanics, the fundamental theory governing the behavior of matter at the atomic and subatomic level, plays a pivotal role in our understanding of how to calculate bond order of polyatomic molecules. The principles of quantum mechanics provide the theoretical foundation for describing and predicting the electronic structure and bonding characteristics of these complex molecules.
At the heart of quantum mechanics lies the concept of wave-particle duality, where particles such as electrons exhibit both wave-like and particle-like properties. This duality leads to the formation of atomic orbitals, which are mathematical functions describing the spatial distribution and energy of electrons within an atom. The overlap of these atomic orbitals during chemical bonding results in the formation of molecular orbitals, which govern the electronic structure and properties of polyatomic molecules.
In practice, quantum mechanics provides sophisticated computational tools, such as density functional theory (DFT) and Hartree-Fock theory, which enable chemists to calculate the electronic structure of polyatomic molecules with remarkable accuracy. These methods solve the Schrdinger equation, a fundamental equation in quantum mechanics, to obtain the wave function of the molecule. Once the wave function is known, various properties, including bond order, can be calculated.
The understanding of bond order derived from quantum mechanics has broad practical applications in chemistry and materials science. It allows researchers to design and synthesize molecules with specific properties, predict reactivity and stability, and understand the behavior of molecules in different environments. Quantum mechanics also underpins the development of new materials, such as semiconductors and superconductors, with tailored electronic and optical properties.
FAQs on Calculating Bond Order of Polyatomic Molecules
This section addresses frequently asked questions to clarify concepts and provide additional insights on “how to calculate bond order of polyatomic molecules.” It covers common concerns and misconceptions, offering a deeper understanding of the topic.
Question 1: What is the significance of bond order in understanding molecular properties?
Answer: Bond order provides valuable information about the strength, stability, and reactivity of chemical bonds. It helps predict molecular properties such as bond length, bond energy, and magnetic behavior.
Question 2: How does hybridization affect bond order calculations?
Answer: Hybridization influences the overlap of atomic orbitals, which in turn affects the bond order. Hybrid orbitals with better overlap result in higher bond orders and stronger bonds.
Question 3: Can bond order be used to predict the reactivity of a molecule?
Answer: Yes, bond order can serve as an indicator of molecular reactivity. Higher bond orders generally correspond to less reactive bonds, while lower bond orders indicate more reactive bonds.
Question 4: How does resonance affect bond order calculations?
Answer: Resonance involves the delocalization of electrons, leading to multiple contributing structures. Bond order in resonance structures is an average of the bond orders in the individual structures.
Question 5: What are some experimental techniques used to determine bond order?
Answer: Experimental techniques include X-ray crystallography, neutron diffraction, and vibrational spectroscopy. These methods provide insights into molecular structure and bond lengths, which can be used to infer bond order.
Question 6: How can computational chemistry assist in bond order calculations?
Answer: Computational chemistry methods, such as density functional theory (DFT), offer powerful tools for calculating bond orders. They employ sophisticated algorithms to solve quantum mechanical equations and provide accurate predictions of molecular electronic structures.
In summary, understanding bond order is crucial for deciphering the electronic structure and properties of polyatomic molecules. These FAQs address common questions and misconceptions, providing a deeper grasp of the topic. In the next section, we will delve into the practical methods for calculating bond orders, exploring both qualitative and quantitative approaches.
Tips for Calculating Bond Order of Polyatomic Molecules
To enhance your understanding and accuracy in calculating bond order, consider incorporating these practical tips into your approach:
Tip 1: Determine the molecular orbital diagram and identify the bonding and antibonding orbitals involved.
Tip 2: Utilize valence bond theory to analyze the hybridization of atomic orbitals and predict bond angles.
Tip 3: Consider resonance structures and their contributions to the overall bond order of the molecule.
Tip 4: Examine the electron configuration of the atoms to determine the number and type of electrons available for bonding.
Tip 5: Employ formal charge calculations to assess the charge distribution within the molecule and its potential impact on bond order.
Tip 6: Utilize oxidation states to understand the hypothetical charge distribution and its relationship to bond order.
Tip 7: Apply quantum mechanical principles, such as molecular orbital theory and density functional theory, for precise bond order calculations.
Tip 8: Leverage experimental techniques, like X-ray crystallography and vibrational spectroscopy, to obtain experimental data that can inform bond order calculations.
By incorporating these tips, you will gain a more comprehensive understanding of the factors influencing bond order and enhance the accuracy of your calculations.
In the concluding section, we will explore advanced applications of bond order calculations in diverse fields, showcasing its significance in understanding molecular properties and chemical reactivity.
Conclusion
Throughout this article, we have delved into the multifaceted topic of “how to calculate bond order of polyatomic molecules.” We have explored qualitative and quantitative approaches, considering key factors such as molecular orbital theory, hybridization, resonance, electron configuration, formal charge, oxidation state, and quantum mechanics. By understanding these concepts and applying them effectively, researchers can gain valuable insights into the electronic structure and bonding characteristics of polyatomic molecules.
The ability to calculate bond order provides a powerful tool for predicting molecular properties, reactivity, and stability. It forms the foundation for understanding chemical bonding and enables the rational design of novel materials with tailored properties. The interconnections between these concepts highlight the complexity and interconnectedness of molecular systems.
As we continue to push the boundaries of chemistry and materials science, the accurate calculation of bond order will remain a cornerstone of our endeavors. It will empower researchers to explore new frontiers in fields such as drug discovery, catalysis, and nanotechnology, shaping the future of scientific discovery and technological innovation.