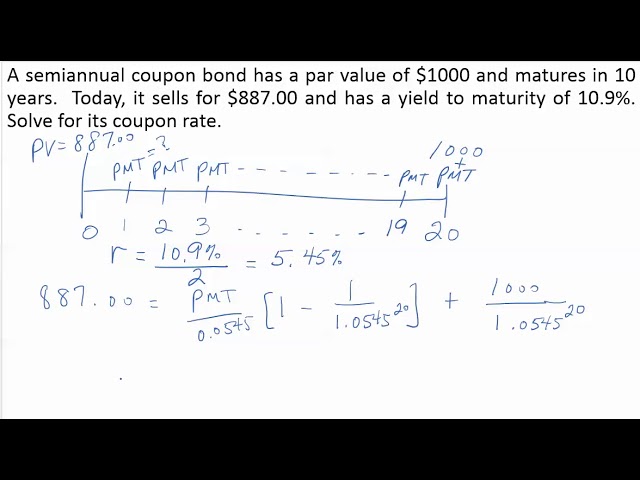
Calculating coupon interest rate, a crucial aspect of fixed-income securities, is the process of determining the interest payments made to bondholders. For instance, a bond with a $1,000 face value and a 5% coupon rate would pay $50 in interest annually.
Understanding coupon interest rate is essential for investors to assess the income potential of bonds, compare different bond offerings, and make informed investment decisions. Historically, the development of zero-coupon bonds, which pay no interest until maturity, has led to increased flexibility in bond structuring.
This article will delve into the formula, factors, and implications of coupon interest rate calculation, providing a comprehensive guide for investors and financial professionals.
How to Calculate Coupon Interest Rate
Understanding the aspects of coupon interest rate calculation is crucial for accessing bond investments. These aspects encompass:
- Bond Face Value
- Coupon Rate
- Payment Frequency
- Bond Maturity
- Accrued Interest
- Interest Payment Date
- Par Value
- Present Value
- Yield to Maturity
Each aspect plays a distinct role in determining the interest payments received by bondholders. For example, bond face value and coupon rate directly influence the dollar amount of interest payments, while payment frequency affects the timing of these payments. Understanding these aspects empowers investors to make informed decisions about bond investments.
Bond Face Value
Bond face value, also known as par value, is the principal amount of a bond, which represents the amount that the issuer of the bond owes to the holder. It is a critical component of how to calculate coupon interest rate because it serves as the base upon which interest payments are calculated. The coupon rate, expressed as a percentage, is applied to the bond face value to determine the dollar amount of interest paid to the bondholder.
For example, consider a bond with a face value of $1,000 and a coupon rate of 5%. The annual interest payment on this bond would be $50, calculated as $1,000 x 5%. This demonstrates the direct relationship between bond face value and coupon interest rate, where a higher face value leads to a higher interest payment, assuming the coupon rate remains constant.
Understanding the connection between bond face value and coupon interest rate is essential for investors to assess the income potential of bonds and compare different bond offerings. By considering both the face value and the coupon rate, investors can make informed decisions about which bonds align with their investment goals and risk tolerance.
Coupon Rate
Coupon rate is a crucial component of how to calculate coupon interest rate, as it directly influences the amount of interest paid to bondholders. Expressed as a percentage, the coupon rate represents the annual interest payment per $100 of face value. This relationship is fundamental in determining the income potential and attractiveness of bonds.
For instance, a bond with a face value of $1,000 and a coupon rate of 5% would pay an annual interest of $50 ($1,000 x 5%). Conversely, a bond with a higher coupon rate, such as 7%, would pay an annual interest of $70, assuming the same face value. This illustrates the direct correlation between coupon rate and coupon interest rate.
Understanding the relationship between coupon rate and coupon interest rate is essential for investors to make informed decisions about bond investments. By considering the coupon rate in conjunction with other factors such as bond face value and maturity, investors can assess the potential return and risk associated with different bonds.
Payment Frequency
Payment frequency, a key aspect of how to calculate coupon interest rate, refers to the schedule of interest payments made to bondholders. Its significance lies in determining the timing and frequency of interest payments, which have implications for investors’ cash flow and overall return.
- Annual Payments
The most common payment frequency, annual payments involve one interest payment per year. Simplicity and ease of management make it a popular choice, with the annual coupon rate directly determining the annual interest payment.
- Semi-Annual Payments
With semi-annual payments, interest is paid twice a year, typically every six months. This more frequent payment schedule provides investors with a more consistent cash flow and can enhance the attractiveness of bonds.
- Quarterly Payments
Quarterly payments offer a higher frequency of interest payments, with interest paid every three months. The increased frequency provides investors with a steadier cash flow, but it may come with slightly lower coupon rates compared to bonds with less frequent payments.
- Monthly Payments
Monthly payments, the most frequent payment schedule, provide investors with a predictable and steady stream of income. While offering the highest level of cash flow consistency, bonds with monthly payments may have lower coupon rates and face higher administrative costs.
Understanding payment frequency is crucial for investors to align their cash flow needs with the timing of interest payments. By considering the frequency of payments in conjunction with other factors such as coupon rate and bond maturity, investors can make informed decisions about which bonds suit their financial goals and risk tolerance.
Bond Maturity
Bond maturity, a crucial aspect of how to calculate coupon interest rate, refers to the predetermined date on which a bond’s principal amount, also known as the face value, becomes due and payable to the bondholder. Understanding bond maturity is vital as it impacts the calculation of interest payments and the overall return on investment.
- Maturity Date
The maturity date is the specific date on which the bond matures and becomes due for repayment. It plays a key role in determining the length of time an investor will hold the bond and receive interest payments.
- Bond Term
Bond term refers to the number of years until a bond matures. It is calculated from the issuance date to the maturity date and influences the level of interest rates and the overall risk associated with the bond.
- Callable Bonds
Callable bonds give the issuer the option to redeem the bonds before the maturity date. This feature affects the calculation of coupon interest rate as it introduces an additional layer of complexity and potential impact on the bond’s value.
- Puttable Bonds
Puttable bonds provide bondholders with the right to sell the bonds back to the issuer before the maturity date. This feature also affects the calculation of coupon interest rate as it introduces a potential change in the bond’s duration and yield.
In summary, bond maturity encompasses various facets that influence how to calculate coupon interest rate. By understanding the maturity date, bond term, and features such as callability and puttability, investors can make informed decisions when evaluating and investing in bonds.
Accrued Interest
Accrued Interest, an integral aspect of how to calculate coupon interest rate, represents the portion of interest that has accumulated on a bond since the last interest payment date but has not yet been paid to the bondholder. Understanding Accrued Interest is crucial for accurate calculation of coupon interest rate and assessment of bond investments.
- Calculation
Accrued Interest is calculated by multiplying the bond’s annual coupon rate by the number of days since the last interest payment date, divided by the number of days in the coupon payment period. - Example
For a bond with a $1,000 face value, a 5% annual coupon rate, and an interest payment date of January 1st, the Accrued Interest on February 15th would be $12.07, assuming a 360-day year. - Impact on Bond Price
Accrued Interest affects the bond’s price. When a bond is purchased between interest payment dates, the buyer must pay the Accrued Interest in addition to the bond’s face value. Conversely, when a bond is sold before the next interest payment date, the seller receives the Accrued Interest as part of the proceeds. - Relevance to Coupon Interest Rate Calculation
Accrued Interest is added to the regular coupon payment to determine the total interest payment received by the bondholder. This accurate calculation is crucial for assessing the bond’s yield and making informed investment decisions.
In summary, Accrued Interest is a key component of how to calculate coupon interest rate, influencing the bond’s price and the total interest payment received by the bondholder. A thorough understanding of its calculation and implications empowers investors to make informed decisions when investing in bonds.
Interest Payment Date
Interest Payment Date, a crucial component of how to calculate coupon interest rate, holds significant implications for bondholders and investors. It represents the predetermined date on which bondholders receive interest payments, typically semi-annually or annually, as specified in the bond’s terms.
The Interest Payment Date directly affects the calculation of coupon interest rate. To accurately determine the interest payment, one must consider the time elapsed since the last payment date. This calculation involves multiplying the bond’s annual coupon rate by the number of days since the last payment date and dividing it by the number of days in the coupon payment period. This process ensures that the accrued interest, which represents the earned but unpaid interest up to the calculation date, is correctly accounted for.
Understanding the relationship between Interest Payment Date and coupon interest rate calculation is essential for investors to determine the timing and amount of interest payments they will receive. This knowledge empowers them to make informed decisions when purchasing and managing bonds, ensuring that they align with their financial goals and cash flow needs.
Par Value
In the context of fixed-income securities, Par Value, also known as Face Value, is a fundamental concept intertwined with the calculation of coupon interest rate. It represents the principal amount of a bond, serving as the basis upon which interest payments are determined.
Par Value plays a pivotal role in calculating coupon interest rate. The coupon rate, expressed as a percentage, is applied to the Par Value to determine the dollar amount of interest paid to the bondholder. For instance, a bond with a Par Value of $1,000 and a coupon rate of 5% would yield an annual interest payment of $50. This relationship underscores the direct impact of Par Value on the coupon interest rate calculation.
Moreover, Par Value holds significance in practical applications. It serves as a reference point for calculating bond prices and yields. When a bond is issued at par, its market price is equal to its Par Value. However, bond prices can fluctuate in the secondary market, resulting in premiums or discounts relative to Par Value. Understanding the interplay between Par Value and coupon interest rate is crucial for investors to assess bond values and make informed investment decisions.
Present Value
In the realm of fixed-income securities, Present Value holds immense significance in the calculation of coupon interest rate. It represents the current worth of future cash flows associated with a bond, discounted at a specified rate, typically the yield to maturity or market interest rate. Understanding Present Value is crucial for investors to accurately determine the fair value of bonds and make informed investment decisions.
- Discounted Cash Flows
Present Value is calculated by discounting each future cash flow, including coupon payments and the final principal repayment, back to the present using the appropriate discount rate. This process reflects the time value of money and allows for the comparison of cash flows occurring at different points in time.
- Yield to Maturity
The discount rate used in Present Value calculations is often the bond’s yield to maturity (YTM). YTM represents the annualized rate of return an investor can expect to earn if they hold the bond until maturity. Understanding the relationship between Present Value and YTM is essential for investors to assess the attractiveness of different bond investments.
- Bond Pricing
Present Value plays a fundamental role in determining bond prices. The market price of a bond is typically close to its Present Value, although it can fluctuate due to factors such as supply and demand. By understanding Present Value, investors can evaluate whether a bond is fairly priced or over/under-valued.
- Investment Decisions
The calculation of Present Value empowers investors to make informed investment decisions. By comparing the Present Value of a bond to its market price, investors can determine whether the bond offers a reasonable return relative to the prevailing interest rates. This analysis helps investors construct bond portfolios that align with their risk tolerance and financial goals.
In summary, Present Value is an integral component of how to calculate coupon interest rate. It allows investors to assess the fair value of bonds, compare investment opportunities, and make informed decisions by considering the time value of money and the relationship between bond prices and yields.
Yield to Maturity
Yield to Maturity (YTM) is a critical component of “how to calculate coupon interest rate”, as it represents the annualized rate of return an investor can expect to earn if they hold the bond until maturity. YTM is closely related to the coupon interest rate, as it reflects the relationship between the bond’s price, coupon payments, and time to maturity.
The relationship between YTM and coupon interest rate is reciprocal. A higher coupon interest rate generally leads to a higher YTM, as investors are willing to pay more for a bond with a higher stated rate of interest. Conversely, a lower coupon interest rate typically results in a lower YTM, as investors demand a higher yield to compensate for the lower coupon payments.
For example, consider two bonds with the same face value and maturity date. One bond has a coupon rate of 5%, while the other has a coupon rate of 10%. Assuming the market interest rate is 8%, the bond with the 5% coupon rate will have a YTM of approximately 7.5%, while the bond with the 10% coupon rate will have a YTM of approximately 9.5%. This demonstrates how the coupon interest rate directly influences the YTM of a bond.
Understanding the relationship between YTM and coupon interest rate is crucial for investors in fixed-income securities. By considering both factors, investors can make informed decisions about which bonds to purchase and how to structure their bond portfolios.
Frequently Asked Questions on Coupon Interest Rate Calculation
These FAQs address common queries and provide clarifications on various aspects of coupon interest rate calculation.
Question 1: What is the formula for calculating coupon interest rate?
Coupon interest rate = (Coupon payment / Bond face value) x (Number of payments per year)
Question 2: How does bond maturity affect coupon interest rate?
Longer bond maturities typically lead to higher coupon interest rates, as investors demand higher returns for locking in their funds for extended periods.
Question 3: What is the impact of payment frequency on coupon interest rate?
More frequent payment schedules (e.g., monthly or quarterly) generally result in lower coupon interest rates compared to less frequent payments (e.g., semi-annual or annual), as investors receive interest payments more often.
Question 4: How is accrued interest calculated?
Accrued interest = (Coupon rate / Number of days in year) x (Number of days since last interest payment)
Question 5: What is the relationship between coupon interest rate and bond price?
Coupon interest rate and bond price are inversely related. When interest rates rise, bond prices tend to fall, leading to higher coupon interest rates on newly issued bonds.
Question 6: How does yield to maturity differ from coupon interest rate?
Yield to maturity represents the annualized return an investor can expect if they hold the bond until maturity, while coupon interest rate only considers the stated interest payments. YTM takes into account the bond’s price, coupon payments, and time to maturity.
These FAQs provide valuable insights into coupon interest rate calculation, empowering investors to make informed decisions and navigate the fixed-income market effectively. Understanding these concepts forms the foundation for further exploration of bond valuation and investment strategies.
Moving forward, we will delve deeper into the complexities of bond pricing and yield calculations.
Tips for Calculating Coupon Interest Rate
This section provides practical tips to assist you in accurately calculating coupon interest rate and making informed investment decisions.
Tip 1: Determine the Bond’s Face Value and Coupon Rate
The face value represents the principal amount of the bond, while the coupon rate is the annual interest rate paid on the face value. Clearly identify these values in the bond’s prospectus or other relevant documentation.
Tip 2: Establish the Payment Frequency
Bonds typically make interest payments semi-annually or annually. Determine the payment frequency to calculate the number of payments per year.
Tip 3: Calculate the Coupon Payment
Multiply the bond’s face value by the coupon rate to determine the dollar amount of each interest payment.
Tip 4: Consider Accrued Interest
If you purchase a bond between interest payment dates, you may need to pay accrued interest in addition to the purchase price. Calculate accrued interest based on the number of days since the last interest payment date.
Tip 5: Understand Yield to Maturity
Yield to maturity (YTM) represents the annualized return you can expect to earn if you hold the bond until maturity. YTM is closely related to coupon interest rate, and understanding both concepts is crucial for bond evaluation.
Tip 6: Use a Bond Calculator
Online bond calculators can simplify the coupon interest rate calculation process. However, it’s important to verify the accuracy of the results.
Tip 7: Consult a Financial Advisor
For complex bond investments or if you need personalized advice, consider consulting a qualified financial advisor who can guide you through the calculations and help you make informed decisions.
Summary: By following these tips, you can effectively calculate coupon interest rate, assess bond investments, and make informed decisions aligned with your financial goals.
Transition: Understanding coupon interest rate calculation is a foundational step in bond investing. In the next section, we will explore advanced concepts related to bond pricing and yield calculations, building on the knowledge gained in this section.
Conclusion
This article has explored the intricacies of “how to calculate coupon interest rate,” providing a comprehensive guide to this essential aspect of fixed-income securities. We have examined the key components involved in coupon interest rate calculation, including bond face value, coupon rate, payment frequency, bond maturity, and accrued interest. Understanding these components is crucial for investors to accurately determine the interest payments they will receive and to make informed investment decisions.
Throughout this exploration, we have highlighted several main points:
1. Coupon rate directly influences coupon interest rate calculation, with a higher coupon rate leading to a higher interest payment.
2. Payment frequency affects the timing and number of interest payments, which can impact investment strategies.
3. Bond maturity and accrued interest play significant roles in determining the total amount of interest earned over the life of the bond.
These interconnected concepts provide a framework for understanding coupon interest rate calculation and its implications for bond investments. By grasping these principles, investors can confidently navigate the fixed-income market and make informed decisions that align with their financial objectives.