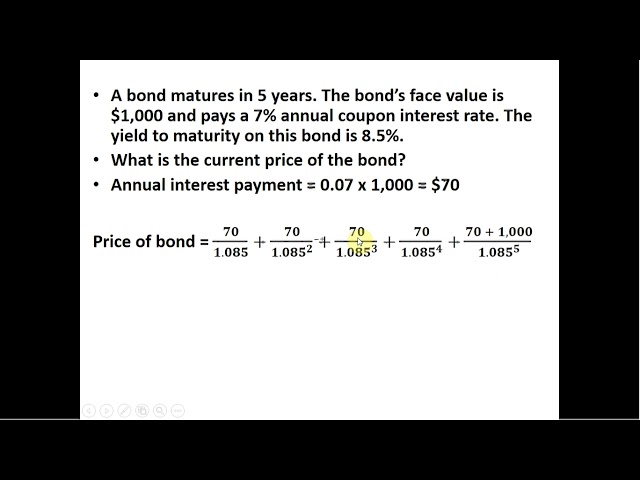
Determining the coupon rate in economics involves calculating the fixed interest rate paid to bondholders, typically expressed as a percentage of the bond’s face value. For instance, a bond with a face value of $1,000 and a 5% coupon rate would pay $50 in interest annually.
Understanding coupon rate is crucial in finance as it influences bond prices, investment decisions, and overall market dynamics. Historically, the coupon rate has played a significant role in bond issuance and has evolved with financial markets’ development.
This article will provide a comprehensive guide to calculating coupon rates, exploring their significance, and examining historical developments that have shaped this essential economic measure.
How to Calculate Coupon Rate in Economics
Calculating the coupon rate is essential for understanding bond pricing, investment decisions, and market dynamics. The key aspects involved in this calculation include:
- Face value
- Interest rate
- Payment frequency
- Bond maturity
- Time to maturity
- Present value
- Future value
- Yield to maturity
- Bond rating
- Market conditions
These aspects are interconnected and influence the overall calculation of the coupon rate. For example, the face value, interest rate, and payment frequency determine the total interest payments made over the life of the bond. The bond maturity and time to maturity affect the present and future value of the bond, which in turn impact the yield to maturity. Market conditions and bond ratings can also influence the coupon rate offered by bond issuers.
Face Value
In the context of coupon rate calculation, the face value, also known as the par value, represents the principal amount of a bond. It is the amount that the bondholder will receive upon the bond’s maturity date, assuming no default occurs. The face value is a critical component in determining the coupon rate, as it serves as the base upon which interest payments are calculated.
The coupon rate is expressed as a percentage of the face value. For instance, a bond with a face value of $1,000 and a coupon rate of 5% will pay annual interest of $50 (1,000 x 0.05). The face value, therefore, directly influences the amount of interest paid to bondholders.
Understanding the relationship between face value and coupon rate is essential for investors and financial analysts. It enables them to assess the attractiveness of a bond investment, compare different bonds, and make informed decisions regarding bond purchases and sales. By calculating the coupon rate and considering it in conjunction with other factors such as bond maturity and credit risk, investors can optimize their investment strategies and achieve their financial goals.
Interest rate
In calculating coupon rates in economics, interest rate plays a pivotal role, directly influencing the amount of interest paid to bondholders. Interest rate can be dissected into various facets, each shaping the overall coupon rate determination.
- Nominal Interest Rate:
The stated, face-value interest rate printed on the bond certificate. It serves as the basis for coupon payments and influences the bond’s market value. - Real Interest Rate:
The nominal interest rate adjusted for inflation. It reflects the actual return on investment, considering the erosion of purchasing power due to price increases. - Effective Interest Rate:
The annual interest rate that accounts for the compounding effect of interest. It provides a more accurate representation of the true cost of borrowing or the return on savings. - Yield to Maturity:
The internal rate of return (IRR) of an investment in a bond, taking into account all future interest payments and the repayment of principal at maturity. It serves as a key benchmark for bond investors.
These facets of interest rate collectively impact the calculation of coupon rates, affecting bond pricing, investment decisions, and overall market dynamics. Understanding the interrelationship between interest rate and coupon rate empowers investors and financial analysts to make informed choices and optimize their financial strategies.
Payment frequency
In the realm of economics and finance, “payment frequency” stands as a critical component in the calculation of coupon rates. It refers to the intervals at which interest payments are distributed to bondholders throughout the life of a bond.
The significance of payment frequency lies in its direct impact on the effective yield and overall attractiveness of a bond investment. Bonds with more frequent interest payments, such as semi-annual or quarterly, provide a steady stream of income for investors. This regular cash flow can be advantageous for those seeking consistent returns or who rely on bond income as part of their financial plan.
Real-life examples abound, demonstrating the practical implications of payment frequency in coupon rate calculation. Take, for instance, two bonds with identical face values and coupon rates but different payment frequencies. The bond with semi-annual payments will yield a higher effective yield compared to the bond with annual payments, assuming all other factors remain constant. This is because the more frequent payments allow for a compounding effect, resulting in a greater total return over time.
Understanding the connection between payment frequency and coupon rate calculation is essential for investors and financial analysts alike. It empowers them to make informed decisions when selecting bonds that align with their investment goals and risk tolerance. By carefully considering the impact of payment frequency, investors can optimize their bond portfolios and maximize their returns.
Bond Maturity
In the calculation of coupon rates, bond maturity holds significant sway. It denotes the predetermined date on which the principal amount of a bond becomes due and payable to the bondholder.
- Face Value:
The principal amount of the bond that is repaid at maturity. - Term to Maturity:
The duration between the issuance date and the maturity date, influencing the bond’s overall yield. - Callable Feature:
A provision that allows the bond issuer to redeem the bond before maturity, affecting the calculation of present value and yield to maturity. - Puttable Feature:
An option granted to bondholders to sell the bond back to the issuer before maturity, potentially impacting the bond’s value and coupon rate.
Understanding the multifaceted nature of bond maturity is crucial for accurately calculating coupon rates. It empowers investors and financial analysts to make informed decisions, assess risk, and optimize their bond portfolios. By considering the interplay between bond maturity and other factors like interest rates and payment frequency, they can navigate the complexities of bond markets and achieve their financial goals.
Time to Maturity
In economics, time to maturity holds a pivotal role in the calculation of coupon rates, as it determines the period over which interest payments will be made and the principal amount repaid. Understanding this connection is essential for investors and financial analysts to make informed decisions regarding bond investments.
Time to maturity directly influences the present value of a bond, which is the current worth of all future cash flows, including interest payments and the repayment of principal at maturity. Longer time to maturity results in a lower present value, as the future cash flows are spread over a more extended period, reducing their current worth. This, in turn, affects the coupon rate, as a higher coupon rate is required to compensate investors for the lower present value of the bond.
Real-life examples illustrate this relationship in the bond market. Consider two bonds with identical face values and coupon rates, but different time to maturity. The bond with a longer time to maturity will have a lower present value and, consequently, a higher coupon rate to attract investors. This is because investors demand a higher return for committing their funds for a more extended period.
In conclusion, time to maturity is a critical component in calculating coupon rates, as it impacts the present value of a bond and the level of return required by investors. By understanding this connection, investors can assess the risk-return profile of bonds and make informed investment decisions that align with their financial goals.
Present value
Present value (PV) is a critical component in calculating coupon rates in economics. It represents the current value of a future sum of money, taking into account the time value of money and the prevailing interest rates. The relationship between present value and coupon rate is bidirectional, as the coupon rate directly influences the present value of a bond, and vice versa.
A higher coupon rate leads to a higher present value for a bond, as the future interest payments are worth more in the present. This is because the coupon payments are discounted back to the present using the coupon rate as the discount rate. Conversely, a lower coupon rate results in a lower present value for the bond, as the future interest payments are worth less in the present.
Real-life examples abound in the bond market. Consider two bonds with identical face values and maturities, but different coupon rates. The bond with a higher coupon rate will have a higher present value, as the higher coupon payments result in a greater present value when discounted back to the present using the prevailing interest rate.
Understanding the connection between present value and coupon rate is essential for investors and financial analysts to accurately calculate bond yields and make informed investment decisions. By considering the impact of coupon rate on present value, investors can assess the risk-return profile of different bonds and select those that align with their investment goals and risk tolerance.
Future value
In the context of calculating coupon rates in economics, future value (FV) plays a significant role in determining the present worth of future cash flows. It represents the value of a sum of money at a specified future date, considering the effects of compounding interest.
- Nominal Future Value:
The future value calculated using the nominal, or stated, interest rate, assuming no change in the interest rate over time. - Real Future Value:
The future value adjusted for inflation, reflecting the actual purchasing power of money in the future. - Effective Future Value:
The future value calculated using the effective, or annualized, interest rate, which accounts for the compounding effect of interest. - Present Value:
The current value of the future value, discounted back to the present using the appropriate discount rate.
Understanding the concept of future value is essential for accurately calculating coupon rates and assessing the value of bonds. By considering the time value of money and the effects of compounding interest, investors can make informed decisions about bond investments and optimize their returns.
Yield to Maturity
Yield to maturity (YTM) is a critical component in calculating coupon rates in economics. It represents the internal rate of return (IRR) of an investment in a bond, considering all future interest payments and the repayment of principal at maturity. In essence, YTM reflects the annualized return an investor can expect to earn by holding the bond until maturity.
The relationship between YTM and coupon rate is reciprocal. A higher coupon rate typically leads to a higher YTM, as the investor receives more interest payments over the life of the bond. Conversely, a lower coupon rate generally results in a lower YTM. However, this relationship is not always straightforward, as other factors such as bond maturity, market conditions, and credit risk can also influence YTM.
Real-life examples illustrate the impact of YTM on coupon rates. Consider two bonds with identical face values and maturities, but different coupon rates. The bond with the higher coupon rate will typically have a higher YTM, assuming all other factors remain constant. This is because the higher coupon payments result in a greater total return over the life of the bond.
Understanding the connection between YTM and coupon rate is essential for investors and financial analysts to accurately calculate bond yields and make informed investment decisions. By considering the interplay between these two factors, investors can assess the risk-return profile of bonds and select those that align with their investment goals and risk tolerance.
Bond rating
Bond rating holds significant importance in the context of calculating coupon rates in economics. It is an assessment of the creditworthiness of a bond issuer, reflecting the likelihood of timely interest payments and repayment of principal.
- Creditworthiness:
The overall evaluation of a bond issuer’s ability to meet its financial obligations, based on factors such as financial health, industry position, and management track record.
- Default Risk:
The probability that a bond issuer will fail to make timely interest payments or repay the principal amount at maturity, influencing the perceived risk of the bond and its coupon rate.
- Investment Grade:
A bond rating indicating a low risk of default, typically assigned to bonds issued by governments, large corporations, and financial institutions.
- Junk Grade:
A bond rating indicating a higher risk of default, usually assigned to bonds issued by companies with lower creditworthiness or higher financial leverage.
Understanding bond ratings is crucial for investors and financial analysts to assess the risk-return profile of bonds and make informed investment decisions. Higher-rated bonds generally have lower coupon rates due to their lower perceived risk, while lower-rated bonds typically offer higher coupon rates to compensate investors for the increased risk of default. By considering bond ratings in conjunction with other factors such as coupon rate, maturity, and yield to maturity, investors can allocate their capital effectively and optimize their bond portfolios.
Market conditions
Market conditions play a pivotal role in the dynamics of coupon rate calculation in economics. They encompass a range of factors that influence the overall supply and demand for bonds, impacting the cost of borrowing for issuers and the returns sought by investors.
- Economic growth:
A period of robust economic growth generally leads to increased demand for bonds as investors seek safe havens for their capital. This can put upward pressure on coupon rates as issuers compete for funds.
- Inflation:
Rising inflation erodes the real value of fixed income payments, making bonds less attractive to investors. To compensate for this, issuers may offer higher coupon rates to entice investors.
- Interest rate environment:
Changes in the prevailing interest rate environment can significantly impact coupon rates. When interest rates rise, coupon rates tend to follow suit, as issuers adjust their borrowing costs.
- Risk appetite:
Investors’ risk appetite influences the demand for bonds. During periods of high risk aversion, investors may flock to bonds, driving down coupon rates. Conversely, when investors are more risk-tolerant, they may seek higher returns, leading to higher coupon rates.
These market conditions are interconnected and constantly evolving, shaping the landscape for bond issuance and investment. Understanding their impact is crucial for accurately calculating coupon rates and making informed decisions in the bond market.
FAQs on Calculating Coupon Rates in Economics
This section addresses frequently asked questions and clarifies essential aspects of calculating coupon rates in economics.
Question 1: What is the formula for calculating the coupon rate?
Answer: Coupon Rate = (Coupon Payment / Face Value) x 100%
Question 2: How does the face value impact the coupon rate?
Answer: The face value serves as the base upon which interest payments are calculated. A higher face value results in higher coupon payments, assuming the coupon rate remains constant.
Question 3: What is the relationship between interest rate and coupon rate?
Answer: The coupon rate is typically influenced by prevailing interest rates. Higher interest rates generally lead to higher coupon rates, as issuers need to offer competitive returns to attract investors.
Question 4: How does payment frequency affect the coupon rate?
Answer: More frequent coupon payments (e.g., semi-annual or quarterly) result in a higher effective yield. This is because investors earn interest more frequently, leading to a higher return over time.
Question 5: What role does bond maturity play in coupon rate calculation?
Answer: Bond maturity influences the present value of the bond, which in turn affects the coupon rate. Longer maturities typically command higher coupon rates to compensate investors for the extended investment period.
Question 6: How do market conditions impact coupon rates?
Answer: Market conditions, such as economic growth, inflation, and risk appetite, can influence the supply and demand for bonds, ultimately affecting coupon rates. For instance, during periods of high demand, coupon rates may decrease.
These FAQs provide a concise overview of the key factors and considerations involved in calculating coupon rates in economics. Understanding these concepts is essential for investors and financial analysts to make informed decisions and navigate the bond market effectively.
In the next section, we will delve into advanced topics related to coupon rate calculation and explore strategies for optimizing bond investments.
Tips to Optimize Coupon Rate Calculations
This section presents actionable tips to enhance the accuracy and effectiveness of coupon rate calculations in economics. By incorporating these strategies, investors and financial analysts can make well-informed decisions in the bond market.
Tip 1: Determine the Issuer’s Creditworthiness: Assess the bond issuer’s financial health and credit history to gauge the likelihood of timely interest payments and principal repayment. Higher creditworthiness generally corresponds to lower coupon rates.
Tip 2: Consider the Bond’s Maturity: Longer maturities typically command higher coupon rates to compensate investors for the extended investment period and associated risks. Carefully evaluate the maturity date in relation to your investment horizon.
Tip 3: Monitor Market Conditions: Stay abreast of economic growth, inflation, interest rate changes, and investor risk appetite, as these factors can influence the supply and demand for bonds, ultimately affecting coupon rates.
Tip 4: Calculate the Yield to Maturity: Determine the internal rate of return (IRR) of the bond to compare it with other investment opportunities. Yield to maturity provides a comprehensive assessment of the bond’s return potential.
Tip 5: Utilize Bond Pricing Tools: Leverage online calculators or financial software to accurately calculate coupon rates and yields. These tools can expedite the process and enhance precision.
Tip 6: Consult Financial Professionals: Seek guidance from experienced financial advisors or investment managers to gain expert insights and personalized advice on coupon rate calculations and bond investments.
Tip 7: Monitor Coupon Payments: Regularly track coupon payments to ensure timely receipt and identify any potential issues. Prompt action can minimize financial losses.
Incorporating these tips can significantly improve the accuracy and outcomes of coupon rate calculations. By following these guidelines, investors and financial analysts can make informed decisions, optimize their bond portfolios, and achieve their financial goals.
The next section of this article will explore advanced strategies for exploiting coupon rates in investment decision-making, further enhancing your understanding of this critical aspect of bond analysis.
Conclusion
This comprehensive guide has explored the intricacies of calculating coupon rates in economics, providing investors and financial analysts with a robust framework for accurate calculations. Understanding the key factors that influence coupon rates, such as face value, interest rate, payment frequency, bond maturity, time to maturity, and market conditions, is essential for making informed investment decisions.
Two main points to reiterate are: (1) Coupon rates are directly tied to the bond’s yield to maturity, which represents the internal rate of return (IRR) of the investment, and (2) Market conditions play a crucial role in shaping the supply and demand dynamics of bonds, ultimately affecting coupon rates. By staying abreast of economic growth, inflation, and risk appetite, investors can anticipate shifts in the bond market and adjust their strategies accordingly.