Ascertaining a bond’s coupon rate is a fundamental task in finance, providing crucial insights for investors and analysts. The coupon rate represents the fixed interest payment made by the bond issuer to holders over the bond’s life.
Understanding how to calculate the coupon rate from financial statements is essential for evaluating bond investments, assessing their risk and return profiles, and making informed decisions. Historically, coupon rates have played a significant role in shaping bond markets and influencing investment strategies.
In this article, we will delve into the methods for calculating coupon rates from financial statements, highlighting their importance and practical applications. We will cover the relevant formulas, provide real-world examples, and discuss the key factors that influence coupon rate determination.
How to Calculate Coupon Rate from Financial Statements
Understanding how to calculate the coupon rate from financial statements is essential for evaluating bond investments, assessing their risk and return profiles, and making informed decisions. Key aspects to consider include:
- Face value
- Coupon payment
- Bond term
- Interest payment frequency
- Bond price
- Yield to maturity
- Callable and putable bonds
- Floating rate bonds
- Zero-coupon bonds
These aspects provide a comprehensive understanding of the factors that influence coupon rate determination. By considering the relationship between face value, coupon payment, bond term, and bond price, investors can accurately calculate the coupon rate and make informed investment decisions.
Face Value
Face value, also known as par value, is the nominal value of a bond, representing the amount that the issuer promises to repay to the bondholder at maturity. The face value serves as a critical component in calculating the coupon rate, which determines the fixed interest payments made to bondholders throughout the bond’s life.
The coupon rate is calculated as a percentage of the face value. For example, a bond with a face value of $1,000 and a coupon rate of 5% will pay annual interest payments of $50 (5% of $1,000). This relationship is essential for bond issuers to determine the total interest expense they will incur during the bond’s term and for investors to assess the potential return on their investment.
Understanding the connection between face value and coupon rate is crucial for various practical applications. Investors use this knowledge to compare bonds with different face values and coupon rates, enabling them to make informed decisions about which bonds align with their investment objectives. Additionally, analysts utilize this understanding to assess the creditworthiness of bond issuers, as a higher face value relative to the issuer’s financial strength may indicate a higher risk of default.
Coupon Payment
Coupon payment is a critical component in calculating the coupon rate of a bond. The coupon rate represents the fixed interest payment made by the bond issuer to holders over the bond’s life, and it is expressed as a percentage of the bond’s face value. The coupon payment is the dollar amount of interest paid to bondholders for each coupon period, which may occur semi-annually or annually. To calculate the coupon payment, we multiply the coupon rate by the face value of the bond.
For example, consider a bond with a face value of $1,000 and a coupon rate of 5%. The annual coupon payment for this bond would be $50 (5% x $1,000). Bondholders receive this fixed interest payment throughout the life of the bond, providing them with a steady stream of income. Understanding the relationship between coupon payment and coupon rate is essential for investors to assess the potential return on their investment and compare different bonds effectively.
The practical applications of this understanding extend beyond investment analysis. Companies use coupon payments to attract investors and raise capital for various purposes, such as funding operations, expansion projects, or debt refinancing. Additionally, understanding coupon payments is crucial for bond issuers to manage their debt obligations and ensure they can meet their interest payment commitments.
Bond Term
Bond term, also known as bond maturity, is a crucial element in calculating the coupon rate of a bond. It represents the period of time from the bond’s issuance until its maturity date, when the principal amount is repaid to bondholders. The bond term directly influences the calculation of the coupon rate, as it determines the number of coupon payments that will be made over the bond’s life.
To calculate the coupon rate, we divide the annual coupon payment by the bond’s face value and multiply by 100. Since the bond term dictates the frequency of coupon payments, it significantly impacts the coupon rate calculation. For instance, a bond with a longer term will have a higher number of coupon payments compared to a bond with a shorter term, assuming both bonds have the same face value and annual coupon payment. Consequently, the bond with the longer term will have a lower coupon rate.
Understanding the relationship between bond term and coupon rate is essential for investors and analysts. It enables them to assess the potential return on their investment and compare different bonds effectively. Longer-term bonds generally offer higher coupon rates to compensate investors for the increased risk associated with longer maturities. Conversely, shorter-term bonds tend to have lower coupon rates due to their lower risk profile.
In conclusion, bond term is a critical component in calculating the coupon rate of a bond. It determines the frequency of coupon payments over the bond’s life, which directly affects the calculation. Understanding this relationship is vital for investors and analysts to make informed decisions about bond investments and assess the associated risks and returns.
Interest Payment Frequency
Interest payment frequency is a crucial aspect in calculating the coupon rate of a bond. It refers to the number of times per year that interest payments are made to bondholders. The coupon rate is the annual interest rate paid on a bond, expressed as a percentage of the bond’s face value. Interest payment frequency directly affects the calculation of the coupon rate, as it determines the number of coupon payments made over the bond’s life.
To calculate the coupon rate, we divide the annual coupon payment by the bond’s face value and multiply by 100. Since the interest payment frequency determines the number of coupon payments per year, it significantly impacts the coupon rate calculation. For instance, a bond with a semi-annual interest payment frequency will have a lower coupon rate compared to a bond with an annual interest payment frequency, assuming both bonds have the same face value and annual coupon payment. This is because the semi-annual bond makes two coupon payments per year, while the annual bond makes only one.
Understanding the relationship between interest payment frequency and coupon rate is essential for investors and analysts. It enables them to assess the potential return on their investment and compare different bonds effectively. Bonds with more frequent interest payments may be more attractive to investors who seek regular income, as they receive interest payments more often. Conversely, bonds with less frequent interest payments may be more suitable for investors who prefer to reinvest the interest payments and benefit from compounding returns.
In conclusion, interest payment frequency is a critical component in calculating the coupon rate of a bond. It determines the number of coupon payments made over the bond’s life, which directly affects the calculation. Understanding this relationship is vital for investors and analysts to make informed decisions about bond investments and assess the associated risks and returns.
Bond Price
Bond price is a critical component in calculating the coupon rate of a bond. It represents the current market value of the bond, which is influenced by various factors such as interest rates, creditworthiness of the issuer, and supply and demand dynamics. The relationship between bond price and coupon rate is inverse, meaning that as the bond price increases, the coupon rate decreases, and vice versa.
To understand this relationship, consider the following example. Suppose a bond has a face value of $1,000 and a coupon rate of 5%. If the bond is trading at a premium, say $1,100, it means that investors are willing to pay more than the face value to acquire the bond. In this case, the effective yield or return on the bond is lower than the coupon rate, as the investor is paying a higher price for the same coupon payments. Conversely, if the bond is trading at a discount, say $900, investors can purchase the bond for less than its face value, resulting in a higher effective yield than the coupon rate.
Understanding the relationship between bond price and coupon rate is essential for investors and analysts. It enables them to assess the potential return on their investment and compare different bonds effectively. Bonds trading at a premium may be attractive to investors seeking income, while bonds trading at a discount may be more suitable for investors seeking capital appreciation. Additionally, this understanding is crucial for bond issuers as it influences the cost of borrowing and the overall attractiveness of their bond offerings.
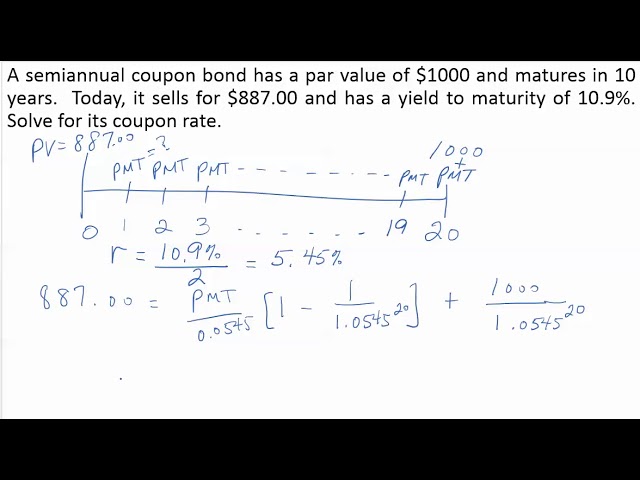
Yield to maturity
Yield to maturity (YTM) is a critical concept closely intertwined with the calculation of coupon rates from financial statements. YTM represents the annualized rate of return an investor can expect to receive if they hold a bond until its maturity date. It encompasses both the coupon payments and any potential capital gains or losses upon maturity.
The relationship between YTM and coupon rate is reciprocal. A bond’s coupon rate directly influences its YTM. Higher coupon rates generally lead to lower YTMs, and vice versa. This is because investors are willing to pay a premium for bonds with higher coupon rates, resulting in a lower YTM. Conversely, bonds with lower coupon rates may trade at a discount, leading to a higher YTM.
Understanding the interplay between YTM and coupon rate is crucial for investors and analysts. It enables them to evaluate the overall attractiveness of a bond investment. Bonds with higher YTMs may be more suitable for investors seeking higher returns, while bonds with lower YTMs may be more appropriate for those seeking stability and income. Additionally, this understanding is essential for bond issuers as it affects the cost of borrowing and the overall success of their bond offerings.
Callable and putable bonds
Callable and putable bonds are types of bonds that give the issuer or the holder the option to redeem the bond before its maturity date. Callable bonds allow the issuer to redeem the bond at a specified price, while putable bonds allow the holder to sell the bond back to the issuer at a specified price. The existence of callable and putable bonds can affect the calculation of coupon rates from financial statements.
Callable bonds typically have lower coupon rates compared to non-callable bonds because the issuer has the option to redeem the bond if interest rates fall. This is because investors demand a higher return for taking on the risk that the bond may be called away from them early. Conversely, putable bonds may have higher coupon rates than non-putable bonds because the holder has the option to sell the bond back to the issuer if interest rates rise. This is because investors are willing to accept a lower return in exchange for the flexibility to exit the investment if interest rates become more favorable.
Understanding the impact of callable and putable bonds on coupon rates is essential for investors when evaluating bond investments. Investors need to consider the potential impact of these features on the overall return and risk profile of the bond. Additionally, analysts need to be aware of these features when calculating coupon rates from financial statements to ensure accurate and reliable financial reporting.
Floating rate bonds
Floating rate bonds (FRBs) are a type of bond whose coupon rate is not fixed but instead fluctuates based on a reference interest rate, such as the London Interbank Offered Rate (LIBOR). This unique feature distinguishes FRBs from traditional fixed-rate bonds and introduces a different approach to calculating coupon rates from financial statements.
In the context of calculating coupon rates from financial statements, FRBs pose a unique challenge. Unlike fixed-rate bonds, where the coupon rate is explicitly stated and remains constant throughout the bond’s life, FRBs require a different approach. Analysts must refer to the terms of the FRB to determine the reference rate and the spread or margin added to that rate to calculate the current coupon rate. This dynamic nature of FRBs necessitates careful attention to the underlying reference rate and the specific terms of the bond.
Real-life examples of FRBs include the LIBOR-linked FRB issued by the World Bank in 2019. This bond had an initial coupon rate set at LIBOR plus 1.10%. As LIBOR fluctuated, so did the coupon rate on the FRB, providing investors with exposure to changes in short-term interest rates. Another example is the Secured Overnight Financing Rate (SOFR)-linked FRB issued by Fannie Mae in 2022. This bond’s coupon rate is pegged to SOFR plus a spread, reflecting the evolving market conditions and the transition away from LIBOR.
Understanding the practical applications of FRBs and their impact on coupon rate calculations is essential for investors and analysts. FRBs offer investors a way to manage interest rate risk and potentially benefit from rising interest rates. For analysts, accurately calculating FRB coupon rates is crucial for accurate financial reporting and assessing the performance and risk profile of these bonds.
Zero-coupon bonds
When delving into the intricacies of calculating coupon rates from financial statements, it is imperative to consider the unique characteristics of zero-coupon bonds. These bonds, devoid of periodic coupon payments, present distinct challenges and opportunities in terms of coupon rate determination.
- No Periodic Coupon Payments
Unlike traditional bonds that pay regular interest payments, zero-coupon bonds do not offer any interim coupon payments. Instead, investors receive a single lump sum payment at maturity, representing the accumulated interest and principal.
- Deep Discount Issuance
Zero-coupon bonds are typically issued at a deep discount to their face value. This discount reflects the absence of periodic coupon payments and represents the implicit interest earned over the bond’s life.
- Yield to Maturity Calculation
Calculating the coupon rate of a zero-coupon bond involves determining the yield to maturity (YTM). YTM represents the internal rate of return an investor will earn if they hold the bond until maturity. It is calculated using the present value formula, considering the bond’s issue price, face value, and time to maturity.
- Real-Life Examples
Zero-coupon bonds are commonly issued by governments and corporations. An example is the U.S. Treasury Zero-Coupon Security, which offers investors a fixed return upon maturity without any interim interest payments.
Understanding the dynamics of zero-coupon bonds is crucial for accurately calculating coupon rates from financial statements. By considering the absence of periodic coupon payments, deep discount issuance, and the unique method of yield to maturity calculation, analysts and investors can effectively assess the return potential and risk profile of these bonds.
Frequently Asked Questions (FAQs)
This section addresses commonly asked questions and clarifies essential aspects related to calculating coupon rates from financial statements.
Question 1: What is the formula for calculating the coupon rate?
Answer: The coupon rate is calculated by dividing the annual coupon payment by the bond’s face value and multiplying by 100. (Coupon Rate = (Annual Coupon Payment / Face Value) * 100)
Question 2: How does bond term impact the coupon rate?
Answer: Longer bond terms generally lead to lower coupon rates, as investors demand higher returns for the increased risk associated with longer maturities.
Question 3: What is the relationship between bond price and coupon rate?
Answer: Bond price and coupon rate are inversely related. As the bond price increases, the coupon rate decreases, and vice versa.
Question 4: How are coupon rates calculated for callable and putable bonds?
Answer: Callable and putable bonds have unique features that can affect coupon rate calculations. Callable bonds typically have lower coupon rates due to the issuer’s option to redeem them early, while putable bonds may have higher coupon rates due to the holder’s option to sell them back to the issuer.
Question 5: How do I calculate the coupon rate of a zero-coupon bond?
Answer: Zero-coupon bonds do not have periodic coupon payments. Instead, their coupon rate is calculated using the yield to maturity (YTM), which represents the internal rate of return an investor will earn if they hold the bond until maturity.
Question 6: What are some real-world examples of coupon rate calculations?
Answer: Real-world examples include calculating the coupon rate of a corporate bond issued by a company to raise capital for expansion or a government bond issued to finance infrastructure projects.
These FAQs provide insights into the various factors and considerations involved in calculating coupon rates from financial statements. Understanding these concepts is crucial for investors, analysts, and anyone seeking to make informed decisions regarding bond investments.
In the next section, we will delve deeper into the practical applications of coupon rate calculations, exploring how they impact investment strategies and financial planning.
Tips to Enhance Coupon Rate Calculations from Financial Statements
This section provides practical tips to enhance the accuracy and efficiency of calculating coupon rates from financial statements:
Tip 1: Accurately Determine Face Value: Identify the bond’s face value, which represents the principal amount that will be repaid at maturity.
Tip 2: Calculate Annual Coupon Payment: Determine the fixed amount of interest paid to bondholders each year, typically expressed as a percentage of the face value.
Tip 3: Consider Bond Term: Take into account the bond’s maturity date, which influences the number of coupon payments to be made over its lifetime.
Tip 4: Determine Interest Payment Frequency: Identify how often interest payments are made, whether annually, semi-annually, or quarterly.
Tip 5: Incorporate Bond Price: Consider the current market price of the bond, which affects the effective yield and thus the coupon rate calculation.
Tip 6: Calculate Yield to Maturity (YTM): Determine the annualized rate of return an investor can expect to receive if they hold the bond until maturity, which is related to the coupon rate.
By following these tips, investors and analysts can enhance the accuracy and efficiency of their coupon rate calculations, leading to better decision-making and improved financial planning.
In the conclusion, we will explore the practical applications of these tips, highlighting how they contribute to informed investment strategies and effective financial management.
Conclusion
This comprehensive exploration of “How to Calculate Coupon Rate from Financial Statements” has illuminated the intricacies of determining the coupon rate, a crucial aspect of bond investments. Key insights gained include the significance of correctly identifying the bond’s face value, annual coupon payment, and bond term, as well as considering the interest payment frequency, bond price, and yield to maturity. These factors, when combined, enable investors and analysts to accurately calculate the coupon rate.
Understanding how to calculate coupon rates empowers individuals to make well-informed investment decisions. It provides a foundation for evaluating the attractiveness of bond offerings, comparing them to alternative investments, and managing bond portfolios effectively. By incorporating the practical tips outlined in this article, investors can enhance the accuracy and efficiency of their calculations, ultimately leading to better financial planning and investment outcomes.