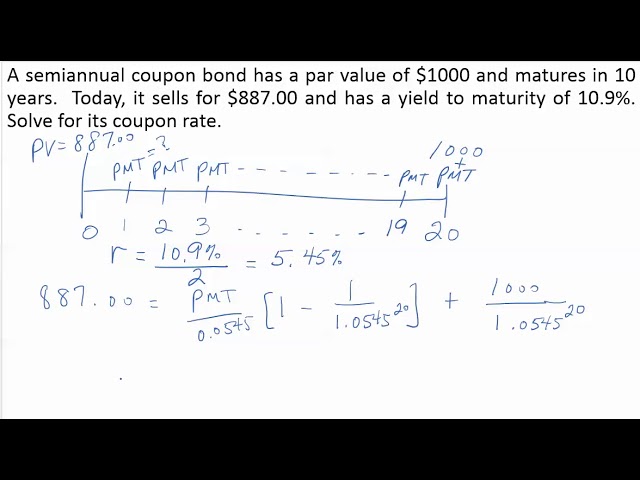
The coupon rate formula is a mathematical equation used to calculate the interest rate on a bond. It is expressed as a percentage of the face value of the bond, and it is paid to the bondholder on a regular basis. For example, a bond with a face value of $1,000 and a coupon rate of 5% would pay the bondholder $50 in interest each year.
The coupon rate formula is an important tool for investors, as it allows them to compare the interest rates offered by different bonds. It can also be used to calculate the yield-to-maturity of a bond, which is the effective annual return on investment. Historically, the coupon rate formula has played a significant role in the development of the bond market, as it provides a standardized way to calculate the interest rate on a bond.
This article will provide a step-by-step guide on how to calculate the coupon rate formula. We will also discuss the importance of the coupon rate formula, and we will provide some examples of how it can be used in practice.
How to Calculate Coupon Rate Formula
The coupon rate formula is an important tool for investors, as it allows them to compare the interest rates offered by different bonds. It can also be used to calculate the yield-to-maturity of a bond, which is the effective annual return on investment.
- Face value: The principal amount of the bond.
- Coupon rate: The annual interest rate paid on the bond, expressed as a percentage of the face value.
- Coupon payment: The amount of interest paid on the bond each year.
- Maturity date: The date on which the bond matures and the face value is repaid.
- Yield-to-maturity: The effective annual return on investment, taking into account the coupon rate and the price of the bond.
- Default risk: The risk that the issuer of the bond will not be able to make the promised interest and principal payments.
- Callable: A bond that the issuer has the option to redeem before the maturity date.
- Puts: A bond that gives the investor the option to sell the bond back to the issuer at a specified price before the maturity date.
- Zero-coupon bond: A bond that does not pay any interest payments, but is sold at a discount to the face value.
- Perpetual bond: A bond that never matures and pays interest payments indefinitely.
These are just a few of the key aspects of the coupon rate formula. By understanding these aspects, investors can make more informed decisions about which bonds to invest in.
Face value
The face value of a bond is the principal amount of the bond, which is the amount that the issuer of the bond promises to repay to the bondholder at the maturity date. The coupon rate is the annual interest rate paid on the bond, expressed as a percentage of the face value. The coupon payment is the amount of interest paid on the bond each year, which is calculated by multiplying the face value by the coupon rate.
The face value of the bond is a critical component of the coupon rate formula, as it is used to calculate the coupon payment. Without the face value, it would not be possible to calculate the coupon payment, which is an important factor for investors to consider when making investment decisions.
For example, a bond with a face value of $1,000 and a coupon rate of 5% would pay the bondholder $50 in interest each year. This is because the coupon payment is calculated by multiplying the face value by the coupon rate, which in this case is $1,000 x 5% = $50.
Understanding the relationship between the face value of a bond and the coupon rate formula is important for investors, as it allows them to compare the interest rates offered by different bonds and make more informed investment decisions.
Coupon rate
The coupon rate is a critical component of the coupon rate formula, as it is used to calculate the coupon payment. Without the coupon rate, it would not be possible to calculate the coupon payment, which is an important factor for investors to consider when making investment decisions.
For example, a bond with a face value of $1,000 and a coupon rate of 5% would pay the bondholder $50 in interest each year. This is because the coupon payment is calculated by multiplying the face value by the coupon rate, which in this case is $1,000 x 5% = $50.
Understanding the relationship between the coupon rate and the coupon rate formula is important for investors, as it allows them to compare the interest rates offered by different bonds and make more informed investment decisions.
In addition to its role in the coupon rate formula, the coupon rate is also used to calculate the yield-to-maturity of a bond. The yield-to-maturity is the effective annual return on investment, taking into account the coupon rate and the price of the bond. Investors use the yield-to-maturity to compare the returns offered by different bonds and to make investment decisions.
Coupon payment
The coupon payment is an important component of the coupon rate formula, as it is the amount of interest that is paid to the bondholder each year. The coupon rate is the annual interest rate paid on the bond, expressed as a percentage of the face value. The coupon payment is calculated by multiplying the face value by the coupon rate. For example, a bond with a face value of $1,000 and a coupon rate of 5% would pay the bondholder $50 in interest each year.
The coupon payment is a critical component of the coupon rate formula, as it is used to calculate the yield-to-maturity of a bond. The yield-to-maturity is the effective annual return on investment, taking into account the coupon rate and the price of the bond. Investors use the yield-to-maturity to compare the returns offered by different bonds and to make investment decisions.
Understanding the relationship between the coupon payment and the coupon rate formula is important for investors, as it allows them to compare the interest rates offered by different bonds and make more informed investment decisions. In addition, understanding this relationship can help investors to calculate the yield-to-maturity of a bond, which is an important factor to consider when making investment decisions.
Maturity date
The maturity date is a critical component of the coupon rate formula, as it is used to calculate the total amount of interest that will be paid over the life of the bond. The coupon rate is the annual interest rate paid on the bond, expressed as a percentage of the face value. The coupon payment is calculated by multiplying the face value by the coupon rate. The total amount of interest paid over the life of the bond is calculated by multiplying the coupon payment by the number of years until the maturity date.
For example, a bond with a face value of $1,000, a coupon rate of 5%, and a maturity date of 10 years would pay the bondholder $50 in interest each year. The total amount of interest paid over the life of the bond would be $500.
Understanding the relationship between the maturity date and the coupon rate formula is important for investors, as it allows them to compare the total amount of interest that will be paid over the life of different bonds. This information can be used to make more informed investment decisions.
Yield-to-maturity
The yield-to-maturity (YTM) is a critical component of the coupon rate formula, as it is used to calculate the total return on investment for a bond. The YTM is the annual rate of return that an investor can expect to receive if they hold the bond until maturity. It is calculated by taking into account the coupon rate, the price of the bond, and the maturity date.
The coupon rate is the annual interest rate paid on the bond, expressed as a percentage of the face value. The price of the bond is the current market price of the bond. The maturity date is the date on which the bond matures and the face value is repaid.
The following formula is used to calculate the YTM:
“`YTM = (C + (FV – PV) / N) / ((FV + PV) / 2)“`Where: C = the annual coupon payment FV = the face value of the bond PV = the present value of the bond N = the number of years until maturityFor example, consider a bond with a face value of $1,000, a coupon rate of 5%, and a maturity date of 10 years. The current market price of the bond is $900. The YTM for this bond can be calculated as follows:“`YTM = (50 + (1000 – 900) / 10) / ((1000 + 900) / 2) = 5.68%“`This means that an investor who purchases this bond can expect to receive an annual return of 5.68% if they hold the bond until maturity.
The YTM is an important metric for investors to consider when making investment decisions. It provides a way to compare the returns offered by different bonds and to make informed decisions about which bonds to purchase.
Default risk
Default risk is a critical component of the coupon rate formula, as it is used to calculate the risk premium that is added to the risk-free rate to determine the coupon rate. The risk premium is a compensation for the risk that the issuer of the bond will not be able to make the promised interest and principal payments. The higher the default risk, the higher the risk premium, and the higher the coupon rate.
There are a number of factors that can affect the default risk of a bond, including the financial health of the issuer, the economic conditions, and the political stability of the country in which the bond is issued. For example, a bond issued by a company with a strong financial track record and a stable business model will have a lower default risk than a bond issued by a company with a weak financial track record and a volatile business model.
Understanding the relationship between default risk and the coupon rate formula is important for investors, as it allows them to make more informed investment decisions. Investors should carefully consider the default risk of a bond before investing, and they should make sure that they are comfortable with the level of risk that they are taking.
There are a number of ways to reduce the risk of default, including diversifying your portfolio, investing in bonds with a high credit rating, and investing in bonds with a short maturity date. By taking these steps, investors can help to protect themselves from the financial consequences of a default.
Callable
A callable bond is a bond that the issuer has the option to redeem before the maturity date. This can be beneficial for the issuer if interest rates fall, as they can refinance the bond at a lower interest rate and save money on interest payments. However, it can be detrimental to the investor, as they may be forced to sell the bond before they are ready, and they may not be able to find a replacement bond with a similar interest rate.
When calculating the coupon rate formula, it is important to take into account the possibility that the bond may be called before the maturity date.
If the bond is called, the investor will receive the face value of the bond plus any accrued interest. The accrued interest is the interest that has accrued since the last interest payment date.
Understanding the relationship between callable bonds and the coupon rate formula is important for investors, as it allows them to make more informed investment decisions. Investors should carefully consider the call features of a bond before investing, and they should make sure that they are comfortable with the risk that the bond may be called before they are ready to sell it.
Puts
The presence of puts can affect the coupon rate formula.
If a bond has a put feature, the investor has the option to sell the bond back to the issuer at a specified price before the maturity date. This can be beneficial for the investor if interest rates rise, as they can sell the bond back to the issuer and reinvest the proceeds in a higher-yielding bond. However, it can be detrimental to the issuer, as they may have to pay back the bond at a time when interest rates are high and refinancing is expensive.
When calculating the coupon rate formula, it is important to take into account the possibility that the bond may be put before the maturity date.
If the bond is put, the investor will receive the put price, which is typically set at a premium to the face value of the bond. The put price is designed to compensate the investor for giving up the option to hold the bond until maturity.
Understanding the relationship between puts and the coupon rate formula is important for investors, as it allows them to make more informed investment decisions. Investors should carefully consider the put features of a bond before investing, and they should make sure that they are comfortable with the risk that the bond may be put before they are ready to sell it.
Zero-coupon bond
Zero-coupon bonds are an important part of the bond market and can be used to calculate the coupon rate formula. They are a type of bond that does not pay any interest payments, but are sold at a discount to the face value. This means that the investor earns a return on the bond through the difference between the purchase price and the face value when the bond matures.
- No interest payments: Zero-coupon bonds do not pay any interest payments throughout their lifetime. This can be beneficial for investors who are looking for a low-risk investment, as they do not have to worry about interest rate fluctuations.
- Sold at a discount: Zero-coupon bonds are sold at a discount to the face value. This discount is equal to the present value of the future interest payments that would have been paid on a traditional bond with the same face value and maturity date.
- Example: A zero-coupon bond with a face value of $1,000 and a maturity date of 10 years might be sold at a price of $500. This means that the investor would earn a return of $500 over the 10-year period, or 5% per year.
- Implications for coupon rate formula: Zero-coupon bonds can be used to calculate the coupon rate formula by using the following formula: Coupon rate = (Face value – Purchase price) / (Years to maturity * Face value). This formula can be used to calculate the coupon rate that would be paid on a traditional bond with the same face value and maturity date as the zero-coupon bond.
Zero-coupon bonds can be a valuable tool for investors who are looking for a low-risk investment with a fixed return. They can also be used to calculate the coupon rate formula, which can be helpful for investors who are comparing different types of bonds.
Perpetual bond
In the context of “how to calculate coupon rate formula,” perpetual bonds present a unique set of considerations. Unlike traditional bonds, which have a fixed maturity date, perpetual bonds continue to pay interest payments indefinitely, making their valuation and coupon rate calculation distinct.
- No Maturity Date: Perpetual bonds lack a predetermined maturity date, meaning that they continue to exist and pay interest in perpetuity.
- Stable Income: Investors value perpetual bonds for their consistent stream of income, making them attractive to those seeking long-term, reliable returns.
- Pricing Considerations: The absence of a maturity date affects the pricing of perpetual bonds, as traditional valuation methods based on discounted future cash flows may not fully capture their value.
- Interest Rate Risk: Perpetual bonds are sensitive to interest rate fluctuations, and their prices can be impacted by changes in the prevailing interest rate environment.
Understanding these aspects of perpetual bonds is crucial when calculating their coupon rate using specialized valuation models that account for their unique characteristics. Additionally, investors should consider the potential impact of interest rate risk and factor it into their investment decisions.
Frequently Asked Questions
This section addresses common questions and concerns related to “how to calculate coupon rate formula.” It aims to clarify key concepts and provide additional insights.
Question 1: What is the coupon rate formula, and how is it used?
Answer: The coupon rate formula calculates the annual interest payment rate on a bond, expressed as a percentage of the bond’s face value. It is used by investors to compare bond yields and make informed investment decisions.
Question 2: What factors influence the coupon rate of a bond?
Answer: The coupon rate is determined by various factors, including the bond’s creditworthiness, maturity date, prevailing interest rates, and market demand.
Question 3: How does the coupon rate affect bond pricing?
Answer: The coupon rate has an inverse relationship with bond prices. Higher coupon rates typically lead to lower bond prices, and vice versa.
Question 4: What is the difference between a coupon bond and a zero-coupon bond?
Answer: Coupon bonds pay regular interest payments, while zero-coupon bonds do not. Instead, zero-coupon bonds are sold at a discount to their face value and provide a return at maturity.
Question 5: How can I use the coupon rate formula to calculate bond yields?
Answer: To calculate bond yields, you can use the coupon rate formula and incorporate factors like bond price, maturity, and interest rates.
Question 6: What are the limitations of using the coupon rate formula?
Answer: The coupon rate formula does not consider all factors that can affect bond yields, such as inflation, default risk, and call provisions.
In summary, the coupon rate formula provides a valuable tool for investors to assess bond characteristics and make informed decisions. However, it is essential to consider the formula’s limitations and other relevant factors when evaluating bonds.
The following section will delve into the practical applications of the coupon rate formula and provide examples of its usage in real-world scenarios.
Tips for Calculating Coupon Rate Formula
This section provides practical tips to help you accurately calculate the coupon rate formula and gain valuable insights into bond investments.
Tip 1: Understand the key components of the coupon rate formula, including face value, coupon rate, and maturity date.Tip 2: Use a financial calculator or online tool to simplify the calculation process.Tip 3: Consider the bond’s creditworthiness and market conditions when interpreting the coupon rate.Tip 4: Compare the coupon rates of different bonds to assess their relative attractiveness.Tip 5: Be aware of the limitations of the coupon rate formula and supplement it with other analysis.Tip 6: Regularly monitor the bond market and stay informed about factors that can affect coupon rates.Tip 7: Consult with a financial advisor if you need personalized guidance in calculating coupon rates and making bond investments.
By following these tips, you can effectively calculate coupon rates, evaluate bond investments, and make informed decisions that align with your financial goals.
The next section of this article will provide a comprehensive analysis of real-world case studies, demonstrating the practical applications of the coupon rate formula and its impact on investment strategies.
Conclusion
This comprehensive analysis on how to calculate coupon rate formula has provided valuable insights into the intricacies of bond investments. The coupon rate formula, a fundamental aspect of bond evaluation, enables investors to assess the attractiveness and suitability of different bonds based on their interest payments and other key characteristics.
Throughout the article, we have explored the significance of the coupon rate formula and its applications in real-world scenarios. The formula’s ability to quantify the periodic interest payments and its inverse relationship with bond prices empowers investors with crucial information for decision-making. Moreover, the consideration of factors such as creditworthiness, maturity, and market conditions further enhances the accuracy and effectiveness of coupon rate calculations.