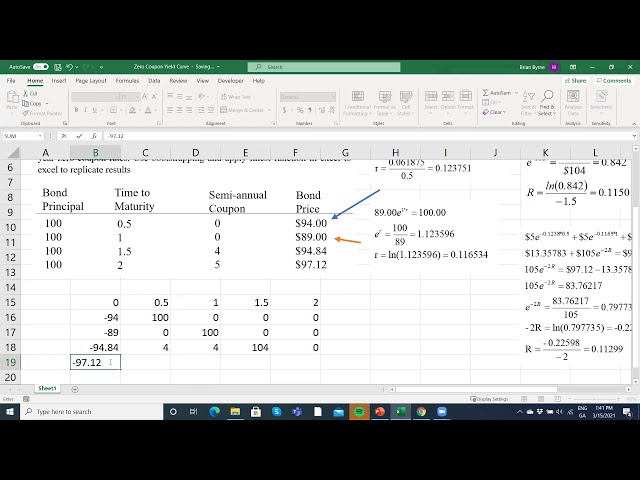
Calculating discount factor zero rate involves determining the present value of a future cash flow using a discount rate that equates to zero. For instance, a $100 payment to be received in one year, discounted at a discount factor zero rate of 10%, would have a present value of $90.91.
This calculation is crucial in finance, allowing for the comparison of cash flows occurring at different points in time. Understanding it provides benefits such as informed investment decisions, accurate project evaluations, and effective risk management. Historically, the concept of discounting future cash flows originated with the work of Irving Fisher in the early 20th century.
In this article, we will explore the formula, applications, and implications of calculating discount factor zero rate, delving into its significance for financial analysis and decision-making.
How to Calculate Discount Factor Zero Rate
Calculating discount factor zero rate requires a comprehensive understanding of various key aspects. These aspects encompass the formula, applications, implications, and significance of this calculation in financial analysis and decision-making.
- Formula
- Applications
- Implications
- Significance
- Present Value
- Future Cash Flows
- Discount Rate
- Time Value of Money
- Investment Decisions
Understanding these aspects is crucial for accurately determining the present value of future cash flows and making informed financial decisions. By exploring the formula, applications, and implications of discount factor zero rate, individuals can gain deeper insights into the time value of money and its impact on financial planning.
Formula
The formula for calculating the discount factor zero rate plays a pivotal role in determining the present value of future cash flows. It establishes the mathematical relationship between the future value, the discount rate, and the time period involved.
- Present Value: The present value is the current worth of a future cash flow, discounted back to the present using the discount factor zero rate.
- Future Cash Flows: The future cash flows represent the anticipated payments or receipts at specified points in time.
- Discount Rate: The discount rate is the rate of return used to discount future cash flows back to the present value.
- Time Period: The time period refers to the duration between the present and the date of the future cash flow.
By incorporating these elements into the formula, the discount factor zero rate provides a means to compare cash flows occurring at different points in time, facilitating informed financial decisions and accurate project evaluations.
Applications
Calculating discount factor zero rate finds diverse applications across various financial domains, enabling informed decision-making and accurate analysis. These applications encompass:
- Investment Appraisal: Discount factor zero rate is crucial in evaluating the viability of investment opportunities by determining the present value of future cash flows, facilitating informed decisions on capital allocation.
- Project Evaluation: It aids in assessing the profitability and feasibility of projects by calculating the net present value, which considers the time value of money and provides insights into the project’s potential.
- Risk Management: Discount factor zero rate plays a significant role in quantifying and managing financial risks associated with future cash flows, enabling businesses to make informed decisions regarding risk mitigation strategies.
- Bond Pricing: The calculation of discount factor zero rate is essential in determining the fair value of bonds, as it helps establish the present value of future coupon payments and the principal amount.
Collectively, these applications underscore the versatility and significance of discount factor zero rate in financial analysis, empowering individuals and organizations to make sound financial decisions and optimize outcomes.
Implications
Understanding the implications of discount factor zero rate calculations is crucial for accurate financial decision-making. These implications encompass a wide range of factors that can impact the outcomes and interpretations of financial analysis.
- Investment Decisions: Discount factor zero rate plays a pivotal role in evaluating the viability of investment opportunities. By considering the time value of money and future cash flows, it helps investors make informed decisions on capital allocation and potential returns.
- Project Feasibility: Calculating discount factor zero rate is essential for project evaluation. It enables businesses to assess the profitability and feasibility of projects by determining the net present value, which incorporates the time value of money and provides insights into the project’s potential.
- Risk Assessment: Discount factor zero rate is a critical component in quantifying and managing financial risks associated with future cash flows. It helps businesses evaluate the potential impact of risks on future earnings and make informed decisions regarding risk mitigation strategies.
- Bond Valuation: The calculation of discount factor zero rate is vital in determining the fair value of bonds. It establishes the present value of future coupon payments and the principal amount, enabling investors to make informed decisions on bond purchases and sales.
These implications underscore the significance and versatility of discount factor zero rate calculations in finance. By considering these factors, individuals and organizations can enhance their financial decision-making processes, optimize outcomes, and navigate financial markets with greater confidence and understanding.
Significance
The significance of discount factor zero rate calculation lies in its fundamental role within financial analysis and decision-making. It enables individuals and organizations to assess the time value of money and accurately compare cash flows occurring at different points in time.
As a critical component of capital budgeting, project evaluation, and risk management, discount factor zero rate calculation provides valuable insights into the potential returns and risks associated with investment opportunities and projects. By incorporating the time value of money, it allows for informed decision-making and the optimization of financial outcomes.
In the context of bond valuation, discount factor zero rate calculation is essential for determining the fair value of bonds. It establishes the present value of future coupon payments and the principal amount, enabling investors to make informed decisions on bond purchases and sales.
Understanding the significance of discount factor zero rate calculation empowers individuals and organizations to navigate financial markets with greater confidence and make informed decisions that align with their financial goals.
Present Value
Present Value is a fundamental concept in finance that represents the current worth of a future sum of money, discounted back to the present. It is closely intertwined with the calculation of discount factor zero rate, as the latter is used to determine the present value of future cash flows.
Discount factor zero rate, also known as the zero-coupon yield, is the rate that equates the present value of a future cash flow to its face value. By incorporating the time value of money, discount factor zero rate allows for the comparison of cash flows occurring at different points in time. The calculation of discount factor zero rate is critical in determining the present value, as it establishes the appropriate discount rate to apply to future cash flows.
In practical applications, present value plays a crucial role in investment analysis and project evaluation. For instance, when evaluating an investment opportunity, analysts calculate the present value of the expected future cash flows to determine the net present value (NPV). A positive NPV indicates that the investment is potentially profitable, considering the time value of money.
In summary, present value and discount factor zero rate are inextricably linked concepts. Discount factor zero rate is used to determine the present value of future cash flows, enabling informed decision-making in various financial contexts. Understanding this relationship is essential for accurate financial analysis and effective investment strategies.
Future Cash Flows
Future Cash Flows play a pivotal role in the calculation of discount factor zero rate, establishing a fundamental connection between the two concepts. Discount factor zero rate, also known as the zero-coupon yield, represents the rate that equates the present value of a future cash flow to its face value. To determine the present value, it is crucial to consider the time value of money, which is reflected in the calculation of discount factor zero rate.
In practical applications, future cash flows are critical components of various financial analyses, including investment appraisals and project evaluations. For instance, when assessing an investment opportunity, analysts project the expected future cash flows and apply a discount factor zero rate to determine the net present value (NPV). A positive NPV indicates that the investment is potentially profitable, considering the time value of money.
Real-life examples further illustrate the significance of future cash flows in the calculation of discount factor zero rate. In bond pricing, the present value of future coupon payments and the principal amount are determined using the discount factor zero rate. This calculation helps establish the fair value of the bond, influencing investment decisions and portfolio management strategies.
Understanding the connection between future cash flows and discount factor zero rate is essential for accurate financial analysis and informed decision-making. By considering the time value of money and incorporating future cash flows into the calculation, individuals and organizations can effectively evaluate investment opportunities, assess project feasibility, and navigate financial markets with greater confidence.
Discount Rate
The discount rate is a critical component of calculating the discount factor zero rate. The discount factor zero rate is the rate that equates the present value of a future cash flow to its face value. The discount rate represents the opportunity cost of capital, reflecting the return that could be earned by investing in an alternative investment with similar risk.
When calculating the discount factor zero rate, the discount rate is used to determine the present value of future cash flows. A higher discount rate results in a lower present value, while a lower discount rate results in a higher present value. This is because a higher discount rate implies a higher opportunity cost of capital, making future cash flows less valuable in present terms.
In practice, the discount rate is often determined based on market conditions and the riskiness of the investment. For example, a company with a higher perceived risk may have to offer a higher discount rate to attract investors. Similarly, during periods of high-interest rates, the discount rate used to evaluate investments may also be higher.
Understanding the relationship between the discount rate and the discount factor zero rate is essential for accurate financial analysis. By considering the discount rate and its impact on the present value of future cash flows, investors and analysts can make informed decisions about investment opportunities and project evaluations.
Time Value of Money
The concept of Time Value of Money (TVM) lies at the core of calculating the discount factor zero rate. TVM recognizes that the value of money diminishes over time due to inflation and the potential for earning interest. This principle plays a critical role in determining the present value of future cash flows, as it accounts for the opportunity cost of holding money over time.
In calculating the discount factor zero rate, TVM is incorporated through the use of a discount rate. The discount rate represents the rate at which future cash flows are discounted back to their present value. A higher discount rate implies a greater time value of money, resulting in a lower present value for future cash flows. Conversely, a lower discount rate places less emphasis on the time value of money, leading to a higher present value.
Real-life examples abound where TVM directly influences the calculation of the discount factor zero rate. Consider bond pricing: investors determine the present value of future coupon payments and the principal amount by applying the discount factor zero rate, which is derived from the prevailing market interest rates. These interest rates reflect the time value of money, as investors seek a return on their investment that compensates them for the loss of purchasing power over time.
Understanding the connection between TVM and the discount factor zero rate is crucial for informed financial decision-making. By considering the time value of money, investors can accurately assess the present value of future cash flows, evaluate investment opportunities, and make sound financial plans. This understanding also enables businesses to optimize their capital budgeting decisions, ensuring that projects with positive net present values are prioritized, considering the time value of money.
Investment Decisions
Investment Decisions play a pivotal role in the application of discount factor zero rate calculations. Understanding how to calculate discount factor zero rate is essential for making informed investment choices and evaluating the potential returns and risks associated with various investment opportunities.
- Capital Budgeting: Calculating discount factor zero rate is crucial in capital budgeting, enabling businesses to assess the viability of long-term investment projects. By determining the present value of future cash flows, companies can make informed decisions on capital allocation and project selection.
- Project Evaluation: Discount factor zero rate calculations are utilized in project evaluation to determine the net present value (NPV) of a project. NPV measures the profitability of a project by considering the time value of money and provides insights into its potential financial impact.
- Risk Assessment: Calculating discount factor zero rate aids in quantifying and managing investment risks. By discounting future cash flows, investors can assess the potential impact of risks on investment returns and make informed decisions regarding risk mitigation strategies.
- Bond Valuation: Discount factor zero rate calculations are essential in bond valuation, as they help determine the present value of future coupon payments and the principal amount. This information enables investors to make informed decisions on bond purchases and sales and evaluate the fair value of bonds.
In summary, understanding how to calculate discount factor zero rate is fundamental for making sound investment decisions. By considering the time value of money and incorporating future cash flows into the calculation, individuals and organizations can enhance their investment strategies, optimize outcomes, and navigate financial markets with greater confidence and understanding.
Frequently Asked Questions
The following FAQs provide answers to common questions and clarifications on key aspects of calculating discount factor zero rate:
Question 1: What is the purpose of calculating discount factor zero rate?
Answer: Calculating discount factor zero rate allows for the determination of the present value of future cash flows, considering the time value of money. This calculation is crucial for various financial applications, including investment analysis and project evaluation.
Question 2: How is discount factor zero rate related to the discount rate?
Answer: Discount factor zero rate is derived from the discount rate, which represents the opportunity cost of capital. A higher discount rate corresponds to a lower present value for future cash flows, emphasizing the time value of money.
Question 3: What factors influence the calculation of discount factor zero rate?
Answer: The calculation considers the future cash flows, the time period involved, and the applicable discount rate. These factors are crucial for determining the present value and assessing the value of future cash flows.
Question 4: How is discount factor zero rate utilized in investment decisions?
Answer: By calculating discount factor zero rate, investors can evaluate the potential returns and risks associated with investment opportunities. This information aids in decision-making regarding capital allocation and project selection.
Question 5: What is the significance of discount factor zero rate in project evaluation?
Answer: Discount factor zero rate plays a critical role in determining the net present value (NPV) of a project. NPV measures the profitability of a project by considering the time value of money and future cash flows.
Question 6: How does discount factor zero rate impact bond valuation?
Answer: In bond valuation, discount factor zero rate is used to determine the present value of future coupon payments and the principal amount. This calculation helps investors assess the fair value of bonds and make informed decisions regarding bond purchases and sales.
These FAQs provide a concise overview of the key aspects and applications of discount factor zero rate calculation. Understanding these concepts is essential for effective financial analysis and decision-making.
The next section will delve into the practical applications of discount factor zero rate in various financial contexts, further exploring its significance and implications.
Tips for Calculating Discount Factor Zero Rate
Understanding how to calculate discount factor zero rate is essential for accurate financial analysis and decision-making. Here are five detailed tips to guide you through the process:
Tip 1: Determine the Future Cash Flows: Identify and quantify the expected cash flows that will occur in the future. These cash flows can be regular payments, such as coupon payments on a bond, or a single lump sum payment.
Tip 2: Establish the Time Period: Determine the duration between the present and the date when each future cash flow will be received. This time period is crucial for applying the appropriate discount factor.
Tip 3: Select the Discount Rate: Choose a discount rate that reflects the opportunity cost of capital or the required rate of return for the investment. The discount rate should be based on market conditions and the riskiness of the cash flows.
Tip 4: Apply the Discount Factor Formula: Use the formula Discount Factor = 1 / (1 + Discount Rate) ^ Time Period to calculate the discount factor for each future cash flow.
Tip 5: Calculate the Present Value: Multiply each future cash flow by its corresponding discount factor to determine its present value. Summing up all the present values will give you the total present value of the future cash flows.
These tips provide a practical framework for calculating discount factor zero rate, enabling informed financial decisions. By considering the time value of money and incorporating future cash flows into the calculation, individuals and organizations can enhance their financial strategies and navigate financial markets with greater confidence.
In the concluding section, we will explore real-world examples and case studies that further demonstrate the applications of discount factor zero rate calculation in various financial contexts.
Conclusion
In summary, calculating discount factor zero rate is a crucial skill in finance, enabling the determination of the present value of future cash flows and informed decision-making. This article has explored the formula, applications, implications, and significance of discount factor zero rate, providing valuable insights into its role in financial analysis and investment strategies.
Key takeaways include the understanding that discount factor zero rate is derived from the discount rate and considers the time value of money. Its applications extend to investment appraisal, project evaluation, risk management, and bond pricing. By incorporating future cash flows and applying the appropriate discount factor, individuals and organizations can evaluate the potential returns and risks associated with various financial opportunities.
Understanding how to calculate discount factor zero rate empowers investors, financial analysts, and business professionals to make informed decisions, optimize outcomes, and navigate financial markets with greater confidence. It is a fundamental concept that forms the basis of sound financial planning and effective capital allocation.