Calculating Duration of Zero Coupon Bonds: A Comprehensive Guide
Calculating duration is a crucial technique for understanding the sensitivity of a bond’s price to changes in interest rates. In the context of zero-coupon bonds, which pay no regular interest payments and make a single payment at maturity, calculating duration becomes particularly important.
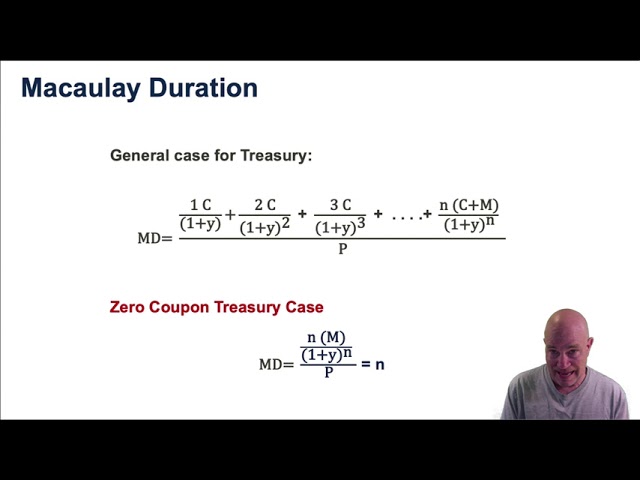
The duration of a bond measures the weighted average time until each of its cash flows is received. For zero-coupon bonds, this calculation is simplified due to the absence of regular interest payments. The duration of a zero-coupon bond is equal to the time until maturity, which is a key factor in determining its price and risk profile.
Understanding how to calculate the duration of zero-coupon bonds is essential for fixed-income investors and portfolio managers alike, as it allows for more informed decision-making and risk management. In this article, we will explore the formula and steps involved in calculating the duration of zero-coupon bonds, providing insights into their behavior and implications.
How to Calculate Duration of Zero Coupon Bond
Calculating the duration of zero-coupon bonds is important for understanding their price sensitivity to interest rate changes. Here are 8 key aspects to consider:
- Time to maturity
- Present value of cash flows
- Weighted average time
- Interest rate sensitivity
- Price volatility
- Investment horizon
- Risk management
- Portfolio optimization
These aspects are crucial for bond investors and portfolio managers to make informed decisions. Duration helps measure the impact of interest rate changes on a bond’s price, enabling investors to manage risk and optimize their portfolios. Understanding these aspects is essential for successful fixed-income investing.
Time to Maturity
Time to maturity is a fundamental aspect in calculating the duration of zero-coupon bonds. It represents the period until the bond reaches its maturity date and makes a single lump-sum payment. Understanding time to maturity is crucial for investors and portfolio managers.
- Maturity Date: The specific date on which the zero-coupon bond matures and the final payment is made.
- Remaining Term: The duration from the present date to the maturity date, which directly influences the bond’s price and sensitivity to interest rate changes.
- Interest Rate Environment: Time to maturity becomes more significant in fluctuating interest rate environments, as it determines the potential impact of rate changes on the bond’s value.
- Investment Strategy: Investors consider time to maturity when aligning bond investments with their investment horizon and risk tolerance.
In summary, time to maturity is a critical factor in calculating the duration of zero-coupon bonds. It encompasses the maturity date, remaining term, prevailing interest rate environment, and alignment with investment strategies. Understanding these facets enables investors to make informed decisions and effectively manage their fixed-income portfolios.
Present Value of Cash Flows
The present value of cash flows plays a pivotal role in calculating the duration of zero-coupon bonds. The duration, a measure of interest rate sensitivity, relies heavily on the timing and magnitude of future cash flows. In the context of zero-coupon bonds, where there are no periodic interest payments, the present value of the single lump sum payment at maturity is the sole determinant of bond value.
To calculate the duration of a zero-coupon bond, one needs to discount the future cash flow (maturity payment) back to the present using the prevailing market interest rate. This process determines the present value, which effectively represents the current worth of the future payment. The higher the market interest rate, the lower the present value, and vice versa. Thus, duration is directly influenced by the present value of the cash flow.
In practical terms, understanding the relationship between present value and duration empowers investors with the ability to assess the impact of interest rate fluctuations on their bond investments. For example, if interest rates rise, the present value of the future cash flow decreases, leading to a shorter duration and lower price for the bond. Conversely, if interest rates fall, the present value increases, resulting in a longer duration and higher price.
By comprehending the interplay between present value and duration, investors can make informed decisions about their bond portfolios. They can adjust the duration of their holdings based on their risk tolerance and interest rate outlook. This understanding enables them to navigate interest rate changes more effectively and achieve their investment goals.
Weighted Average Time
Weighted average time is a crucial element in the formula for calculating the duration of zero-coupon bonds. It encapsulates the time-weighted distribution of the present value of future cash flows, providing insights into the bond’s sensitivity to interest rate fluctuations.
- Time to Maturity: The time remaining until the bond matures, representing the endpoint of the weighted average calculation.
- Present Value: The value of each future cash flow discounted back to the present using prevailing interest rates.
- Weighting: The proportion of the total present value represented by each cash flow, determined by its time to maturity.
- Summation: The weighted average time is calculated by multiplying each time to maturity by its weight and then summing the results.
Understanding weighted average time is vital for investors as it provides insights into how interest rate changes will impact the bond’s price. A longer weighted average time implies a greater sensitivity to interest rate fluctuations, while a shorter weighted average time indicates a lower sensitivity. This information is crucial for making informed investment decisions and managing risk effectively.
Interest rate sensitivity
Interest rate sensitivity is a crucial aspect of calculating the duration of zero-coupon bonds, as it measures the bond’s price volatility in response to interest rate changes. A bond’s duration indicates its sensitivity to interest rate fluctuations, with a longer duration implying a greater sensitivity.
- Price impact: Interest rate changes have an inverse impact on bond prices. When interest rates rise, zero-coupon bond prices fall, and vice versa. This is because higher interest rates make newly issued bonds more attractive, reducing the demand for existing bonds with lower interest rates.
- Time to maturity: The time to maturity of a zero-coupon bond significantly influences its interest rate sensitivity. Bonds with longer maturities tend to be more sensitive to interest rate changes compared to shorter-term bonds.
- Present value: The present value of a zero-coupon bond’s future cash flows is inversely related to interest rates. Higher interest rates result in a lower present value, leading to a shorter duration and reduced price sensitivity.
- Investment strategy: Understanding interest rate sensitivity is crucial for investors to align their bond investments with their investment horizon and risk tolerance. Investors seeking to minimize interest rate risk may opt for bonds with shorter durations, while those seeking higher returns may consider bonds with longer durations.
In summary, interest rate sensitivity is a fundamental consideration when calculating the duration of zero-coupon bonds. It is influenced by factors such as price impact, time to maturity, present value, and investment strategy. By understanding the relationship between interest rates and bond prices, investors can make informed decisions and effectively manage their fixed-income portfolios.
Price volatility
Price volatility is a critical aspect to consider when calculating the duration of zero-coupon bonds, as it measures the potential fluctuations in a bond’s price due to changes in interest rates. Understanding price volatility is crucial for investors to make informed decisions and manage risk effectively.
- Interest rate sensitivity: Zero-coupon bonds are particularly sensitive to interest rate changes, as their prices move inversely to interest rates. This means that when interest rates rise, zero-coupon bond prices fall, and vice versa.
- Time to maturity: The time until a bond matures also affects its price volatility. Generally, bonds with longer maturities have greater price volatility compared to shorter-term bonds, as they are exposed to a wider range of interest rate fluctuations.
- Market conditions: The overall market conditions can influence the price volatility of zero-coupon bonds. In periods of economic uncertainty or market turmoil, bond prices may experience increased volatility as investors seek safe haven assets or adjust their risk appetite.
- Credit risk: The creditworthiness of the bond issuer can also impact price volatility. Bonds issued by issuers with lower credit ratings may have higher price volatility, as investors demand a higher return to compensate for the increased risk of default.
Understanding these facets of price volatility enables investors to make informed decisions about zero-coupon bond investments. By considering the potential price fluctuations, investors can align their investment strategies with their risk tolerance and financial goals.
Investment horizon
Investment horizon plays a crucial role in calculating the duration of zero-coupon bonds. It refers to the period over which an investor plans to hold a bond investment, influencing the bond’s risk and return profile.
- Time frame: The investment horizon encompasses the duration for which an investor intends to hold a zero-coupon bond. This can range from short-term (less than a year) to long-term (over 10 years).
- Risk tolerance: Investors with longer investment horizons tend to have a higher tolerance for risk, as they can ride out market fluctuations over time. Conversely, those with shorter investment horizons may prefer bonds with shorter durations to minimize risk.
- Interest rate expectations: Investors with longer investment horizons need to consider potential interest rate changes over the bond’s life. Rising interest rates can lead to price declines for zero-coupon bonds, so investors should align their investment horizon with their interest rate outlook.
- Financial goals: The investment horizon should align with the investor’s financial goals. For instance, investors saving for retirement may choose longer-term bonds to match their long-term investment horizon.
Understanding investment horizon is essential for calculating the duration of zero-coupon bonds. By considering the duration of their investment horizon relative to the bond’s maturity date and interest rate environment, investors can make informed decisions that align with their risk tolerance and financial goals.
Risk management
When calculating the duration of zero-coupon bonds, risk management is paramount. It encompasses various strategies and techniques employed to assess and mitigate potential risks associated with bond investments. Understanding these facets enables investors to make informed decisions and manage their portfolios effectively.
- Interest rate risk: Interest rate fluctuations can impact the price of zero-coupon bonds. Calculating duration helps investors gauge the sensitivity of a bond’s price to interest rate changes, allowing them to manage their exposure to this risk.
- Credit risk: Zero-coupon bonds issued by entities with lower credit ratings carry a higher risk of default. Duration analysis can provide insights into the potential impact of credit risk on the bond’s value.
- Inflation risk: Inflation can erode the real value of future cash flows from zero-coupon bonds. Duration analysis helps investors assess how inflation may affect the bond’s returns over time.
- Liquidity risk: Zero-coupon bonds may have lower liquidity than other types of bonds, making it more difficult to sell them quickly. Duration analysis can help investors understand the potential liquidity constraints and plan accordingly.
By considering these risk management aspects, investors can make informed decisions about the duration of zero-coupon bonds they invest in. This analysis empowers them to align their investment strategies with their risk tolerance and financial goals, maximizing returns while minimizing potential losses.
Portfolio optimization
In the context of calculating the duration of zero-coupon bonds, portfolio optimization plays a pivotal role in managing risk and maximizing returns. It involves strategically allocating assets within a portfolio to achieve specific investment goals. This comprehensive approach considers various factors, including duration, to enhance the overall performance of the portfolio.
- Diversification: Diversifying a portfolio by incorporating zero-coupon bonds with varying durations can reduce overall risk. By spreading investments across different maturities, investors can mitigate the impact of interest rate fluctuations on the portfolio’s value.
- Immunization: Immunization is a portfolio optimization technique that aims to minimize interest rate risk. By carefully matching the duration of a portfolio to the investor’s investment horizon, it is possible to neutralize the impact of interest rate changes on the portfolio’s value.
- Yield Curve Positioning: Understanding the shape of the yield curve is crucial for portfolio optimization. By investing in zero-coupon bonds with durations that correspond to the expected path of interest rates, investors can enhance returns and manage risk effectively.
- Rebalancing: Periodically rebalancing a portfolio is essential to maintain the desired asset allocation and duration profile. As interest rates and market conditions change, adjusting the portfolio’s composition ensures that it continues to align with the investor’s risk tolerance and financial goals.
In summary, portfolio optimization is a multifaceted process that involves diversification, immunization, yield curve positioning, and rebalancing. By incorporating these strategies, investors can enhance the performance of their zero-coupon bond portfolios, reduce risk, and achieve their financial objectives. Understanding the duration of zero-coupon bonds is a critical component of portfolio optimization, enabling investors to make informed decisions and optimize their investment strategies.
Frequently Asked Questions on Calculating Duration of Zero Coupon Bonds
This FAQ section provides answers to common questions and clarifies key aspects related to calculating the duration of zero-coupon bonds.
Question 1: What is duration, and why is it important?
Answer: Duration measures a bond’s price sensitivity to interest rate changes. It is crucial for understanding the potential impact of interest rate fluctuations on a bond’s value.
Question 2: How is duration calculated for zero-coupon bonds?
Answer: For zero-coupon bonds, duration is equal to the time until maturity, as there are no periodic interest payments.
Question 3: What factors influence the duration of zero-coupon bonds?
Answer: The primary factor influencing duration is time to maturity. Bonds with longer maturities have longer durations.
Question 4: How does duration affect bond prices?
Answer: Duration and bond prices move inversely. When interest rates rise, bond prices fall, and vice versa. Bonds with longer durations are more sensitive to these changes.
Question 5: Why is duration important for investors?
Answer: Duration helps investors assess the risk and return profile of zero-coupon bonds. It allows them to make informed decisions about their bond investments.
Question 6: How can investors use duration in their investment strategies?
Answer: Investors can use duration to diversify their portfolios, manage interest rate risk, and optimize their overall investment returns.
These FAQs provide a concise overview of the key aspects related to calculating the duration of zero-coupon bonds. Understanding these concepts is essential for investors seeking to make informed decisions and effectively manage their fixed-income portfolios.
The next section of this article will delve deeper into the practical applications of duration in fixed-income investing.
Practical Applications of Duration in Fixed-Income Investing
This section presents actionable tips to effectively utilize duration in fixed-income investing.
Tip 1: Assess Interest Rate Risk: Calculate the duration of bonds to gauge their sensitivity to interest rate changes. This helps investors understand the potential impact of rate fluctuations on their portfolios.
Tip 2: Diversify with Duration: Diversify portfolios by investing in bonds with varying durations. This mitigates risk as bonds with different durations react differently to interest rate changes.
Tip 3: Immunize Against Rate Risk: Use immunization strategies to neutralize interest rate risk. Match the duration of a portfolio to the investment horizon to minimize the impact of rate fluctuations.
Tip 4: Position Along the Yield Curve: Study the yield curve to make informed investment decisions. Invest in bonds with durations that correspond to the expected path of interest rates.
Tip 5: Rebalance Regularly: Periodically rebalance portfolios to maintain desired asset allocation and duration profile. Adjust holdings as interest rates and market conditions change.
Tip 6: Consider Issuer Creditworthiness: Duration analysis should incorporate the creditworthiness of bond issuers. Bonds issued by entities with lower credit ratings may carry higher risk and should be considered accordingly.
Tip 7: Monitor Economic Indicators: Stay informed about economic indicators that may influence interest rates. Monitor inflation data, GDP growth, and central bank policies to make informed investment decisions.
Tip 8: Seek Professional Advice: Consult with financial professionals to analyze duration and make appropriate investment decisions. They can provide personalized guidance based on individual circumstances and risk tolerance.
By applying these tips, investors can enhance their fixed-income investment strategies, manage risk effectively, and strive to achieve their financial goals.
The following section will further explore advanced concepts and strategies related to duration in fixed-income investing.
Conclusion
In summary, calculating the duration of zero-coupon bonds provides valuable insights into their behavior and implications. By understanding the formula, time to maturity, present value, and weighted average time, investors can determine a bond’s interest rate sensitivity and price volatility. This knowledge enables effective risk management and portfolio optimization.
Key points to remember include:
- Duration measures the price sensitivity of a bond to interest rate changes, with longer durations indicating greater sensitivity.
- Zero-coupon bonds have a single maturity date, making their duration equal to the time until maturity.
- Understanding duration helps investors align their bond investments with their risk tolerance and investment horizon.
Calculating the duration of zero-coupon bonds is a crucial skill for fixed-income investors. By incorporating duration analysis into their investment strategies, investors can make informed decisions, manage risk, and enhance their portfolio performance.