Calculating Forward Rates with Discount Factors: A Guide to Interest Rate Determination
Forward rate calculation using discount factors is a vital financial tool for assessing future interest rates. In simple terms, it involves using present values to predict future cash flows. Take, for example, a bank anticipating a customer’s loan repayment in one year. By employing discount factors, the bank can estimate the present value of the future payment, aiding in accurate assessment and risk management.
Understanding forward rate calculation is imperative for finance professionals, as it provides insights into future interest rate trends and assists in financial planning and decision-making. Its historical development dates back to the 1980s, with the advent of financial derivative instruments like interest rate swaps. These instruments introduced the concept of forward rates, enabling traders to hedge against interest rate fluctuations.
This article delves into the basics of forward rate calculation using discount factors, highlighting essential principles and practical applications. We will explore various aspects, including the formula, its derivation, and real-world examples. Whether you’re a finance student, an aspiring analyst, or an industry professional, this guide will provide valuable insights and enhance your understanding of forward rate dynamics.
How to Calculate Forward Rate Using Discount Factor
Calculating forward rates using discount factors is a vital skill in finance, enabling professionals to predict future interest rates and make informed financial decisions. Essential aspects to consider include:
- Discount Factor
- Forward Rate Formula
- Zero-Coupon Bond
- Annuity
- Compounding
- Present Value
- Time Value of Money
- Risk-Free Rate
Understanding these aspects is crucial for accurate forward rate calculation. Discount factors, for example, represent the present value of a future cash flow, while the forward rate formula establishes the relationship between spot and forward rates. Zero-coupon bonds and annuities provide practical applications of forward rate calculation, showcasing how it aids in pricing and valuing financial instruments. Compounding, present value, and time value of money are fundamental concepts that underpin forward rate calculations.
Discount Factor
In the context of forward rate calculation, the discount factor plays a central role in determining the present value of future cash flows. It represents the relationship between the spot rate and the forward rate, providing valuable insights into future interest rate movements.
- Present Value Calculation
The discount factor is crucial for calculating the present value of future cash flows. By multiplying the future cash flow by the discount factor, we can determine its present value, which is essential for decision-making and financial planning. - Zero-Coupon Bonds
Discount factors are directly related to zero-coupon bond pricing. The price of a zero-coupon bond is simply the present value of its future cash flow, which is determined using the appropriate discount factor. - Compounding Effect
Discount factors incorporate the effect of compounding, which is the earning of interest on interest. This is crucial for accurately calculating the present value of cash flows that occur over multiple periods. - Risk-Free Rate
The discount factor is typically based on the risk-free rate, which represents the return on a hypothetical investment with no risk. This assumption simplifies the calculation and provides a standardized reference point for comparing forward rates.
In summary, the discount factor is a fundamental concept in forward rate calculation, providing a means to determine the present value of future cash flows and aiding in the pricing of financial instruments. Its close association with zero-coupon bonds, the compounding effect, and the risk-free rate highlights its importance in financial analysis and decision-making.
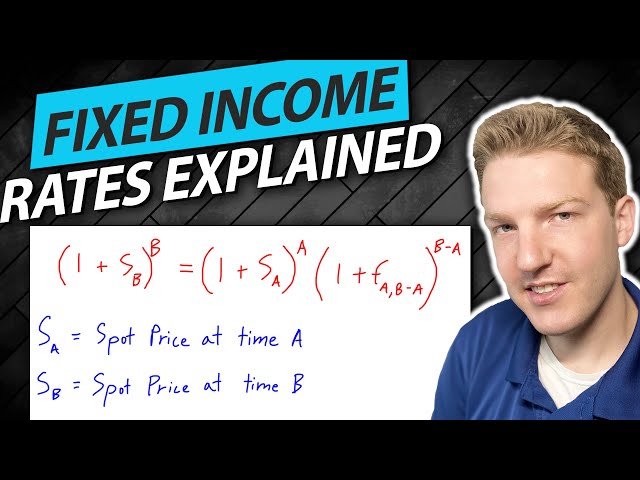
Forward Rate Formula
The forward rate formula is a crucial aspect of calculating forward rates using discount factors. It establishes the mathematical relationship between spot rates and forward rates, providing insights into future interest rate movements and aiding financial decision-making. Here are four key facets of the forward rate formula:
- Spot Rate
The spot rate represents the current interest rate for immediate borrowing or lending. It serves as the starting point for forward rate calculation and reflects the prevailing market conditions. - Forward Rate
The forward rate is the interest rate that is expected to prevail at a specific future date. It is derived from the spot rate and other factors that influence interest rate movements. - Discount Factor
The discount factor is a mathematical function that converts future cash flows into their present values. It is calculated using the spot rate and the time period between the present and future dates. - Time to Maturity
The time to maturity is the period between the present date and the date when the future cash flow is expected to occur. It is a crucial factor in determining the appropriate discount factor and forward rate.
These facets of the forward rate formula are interconnected and essential for accurate forward rate calculation. By understanding these components and their relationships, financial professionals can effectively forecast future interest rates and make informed decisions regarding investments, borrowing, and other financial strategies.
Zero-Coupon Bond
In the context of forward rate calculation using discount factors, zero-coupon bonds play a crucial role. These bonds, also known as deep discount bonds or accrual bonds, have unique characteristics that make them valuable in forward rate determination.
- No Coupon Payments
Zero-coupon bonds do not make periodic coupon payments like traditional bonds. Instead, they are issued at a deep discount to their face value and redeemed at maturity for the full face value. - Present Value
The price of a zero-coupon bond is simply the present value of its future cash flow, which is determined using the appropriate discount factor. This makes zero-coupon bonds ideal for forward rate calculation, as they provide a direct link between spot rates and forward rates. - Forward Rate Estimation
By analyzing the prices of zero-coupon bonds with different maturities, investors can derive forward rates for various future periods. This information is valuable for forecasting interest rate movements and making informed investment decisions. - Pricing and Yield-to-Maturity
The forward rate calculation using discount factors is essential for pricing zero-coupon bonds and determining their yield-to-maturity. This information is crucial for investors seeking attractive returns and managing interest rate risk.
In summary, zero-coupon bonds are unique financial instruments that are closely tied to forward rate calculation using discount factors. Their distinct characteristics, such as the absence of coupon payments and their deep discount pricing, make them valuable tools for investors and financial analysts seeking to understand and forecast future interest rate movements.
Annuity
In the context of forward rate calculation using discount factors, annuities play a crucial role in understanding and valuing future cash flows. An annuity refers to a series of equal payments made at regular intervals, typically annually or semi-annually, over a specified period.
- Types of Annuities
Annuities can be classified into two main types: ordinary annuities, where payments are made at the end of each period, and annuities due, where payments are made at the beginning of each period.
- Present Value of an Annuity
The present value of an annuity is the current value of a series of future payments, calculated using the appropriate discount factor and considering the time value of money.
- Forward Rate and Annuity
Forward rates can be derived from the prices of annuities. By analyzing the prices of annuities with different maturities, investors can deduce forward rates for various future periods.
- Annuity Contracts
Annuities are often used in financial contracts, such as pensions and insurance policies, to provide a guaranteed stream of income over a specific time frame.
In summary, annuities are essential components in forward rate calculation using discount factors, providing a means to value future cash flows and derive forward rates. Their applications extend to a variety of financial contexts, including pensions, insurance, and investment planning.
Compounding
Compounding, a fundamental concept in finance, plays a crucial role in forward rate calculation using discount factors. It refers to the phenomenon where interest is earned not only on the principal amount but also on the accumulated interest from previous periods. This effect leads to exponential growth of investments over time and is a key consideration in long-term financial planning.
- Time Value of Money
Compounding embodies the time value of money, highlighting the importance of considering the impact of inflation and interest rates on future cash flows. It demonstrates how the value of money diminishes over time due to inflation and how interest earned can mitigate this effect. - Exponential Growth
Compounding leads to exponential growth of investments. Even modest interest rates can result in substantial returns over extended periods, making it a powerful tool for wealth accumulation. - Investment Horizons
The impact of compounding is particularly significant for long-term investments. The longer the investment horizon, the more pronounced the effect of compounding, highlighting the importance of starting to invest early. - Discount Factor Calculation
Compounding is intricately linked to the calculation of discount factors. Discount factors, used to determine the present value of future cash flows, incorporate the effects of compounding, ensuring accurate valuation of long-term investments.
In summary, compounding is an essential aspect of forward rate calculation using discount factors, capturing the exponential growth of investments over time and influencing the valuation of long-term cash flows. Understanding the implications of compounding is critical for informed financial decision-making and effective investment planning.
Present Value
In the context of forward rate calculation using discount factors, present value plays a crucial role in determining the current worth of future cash flows. By incorporating the time value of money and discounting future cash flows back to the present, it provides valuable insights for financial decision-making and investment analysis.
- Time Value of Money
Present value reflects the concept of time value of money, which recognizes that the value of a future sum of money is less than its value today due to the potential earnings it could have generated if invested. - Discounting
The process of calculating present value involves discounting future cash flows using an appropriate discount rate. This rate represents the cost of capital or the rate of return that could be earned on alternative investments. - Investment Appraisal
Present value is widely used in investment appraisal to compare and evaluate different investment options. By calculating the present value of future cash flows, investors can determine the net present value (NPV) and make informed decisions regarding project selection. - Bond Pricing
Present value is also essential in bond pricing. The present value of a bond’s future coupon payments and principal repayment at maturity determines its current market value.
Overall, present value is a fundamental concept in forward rate calculation using discount factors, providing a basis for evaluating future cash flows and making sound financial decisions. Its applications extend to a wide range of financial contexts, including investment analysis, project appraisal, and bond valuation.
Time Value of Money
The concept of Time Value of Money (TVM) holds great significance in the realm of forward rate calculation using discount factors. TVM recognizes that the value of money fluctuates over time due to factors such as inflation and opportunity cost. As a result, a sum of money today is worth more than the same sum in the future.
In the context of forward rate calculation, TVM plays a critical role in determining the present value of future cash flows. Forward rates, which represent the anticipated interest rates at specific future dates, are calculated using discount factors that incorporate TVM. Discount factors are derived from spot rates and account for the time value of money, ensuring that future cash flows are appropriately valued in today’s terms.
A practical example of TVM in forward rate calculation is the pricing of zero-coupon bonds. These bonds do not pay periodic coupons but are issued at a discount to their face value and redeemed at maturity for the full face value. The price of a zero-coupon bond is determined by discounting its future cash flow back to the present using an appropriate discount factor that reflects the time value of money and the prevailing forward rate.
Understanding the relationship between TVM and forward rate calculation is essential for various financial applications, including investment analysis, portfolio management, and risk assessment. By incorporating TVM into forward rate calculations, financial professionals can make informed decisions that account for the time value of money and enhance the accuracy of their financial projections.
Risk-Free Rate
In the context of forward rate calculation using discount factors, the risk-free rate is a critical input that forms the foundation for determining future interest rates. It represents the theoretical rate of return on an investment with no risk of default, serving as a benchmark against which other investments are compared.
- Government Bonds
Government bonds issued by highly creditworthy governments, such as U.S. Treasury bonds, are often considered risk-free investments and are used as a proxy for the risk-free rate. - Inflation Expectations
The risk-free rate is closely tied to inflation expectations. Central banks typically set interest rates with the aim of controlling inflation, and the risk-free rate reflects market expectations of future inflation. - Economic Conditions
Economic conditions, such as GDP growth and unemployment rates, can influence the risk-free rate. A strong economy with low unemployment tends to lead to higher risk-free rates, while a weak economy with high unemployment tends to lead to lower risk-free rates. - Market Liquidity
The liquidity of the market for risk-free investments can also affect the risk-free rate. A liquid market with high trading volume tends to result in lower risk-free rates, as investors are more willing to lend at lower rates when they can easily buy and sell their investments.
Understanding the concept of the risk-free rate and its various facets is crucial for accurate forward rate calculation. By incorporating these factors into the calculation, financial professionals can enhance the precision of their forecasts and make more informed decisions regarding interest rate risk management.
FAQs
This section provides answers to frequently asked questions about forward rate calculation using discount factors, addressing common concerns and clarifying important aspects of the process.
Question 1: What is a discount factor?
Answer: A discount factor is a mathematical function that converts future cash flows into their present values, considering the time value of money and the prevailing interest rates.
Question 2: How is the forward rate formula derived?
Answer: The forward rate formula is derived from the relationship between spot rates, discount factors, and the time to maturity of the underlying cash flow.
Question 3: What role do zero-coupon bonds play in forward rate calculation?
Answer: Zero-coupon bonds provide a direct link between spot rates and forward rates, as their prices are determined by discounting their future cash flows using appropriate discount factors.
Question 4: How does compounding affect forward rate calculation?
Answer: Compounding considers the exponential growth of investments over time, which influences the present value of future cash flows and the calculation of discount factors.
Question 5: What is the relationship between the risk-free rate and forward rate calculation?
Answer: The risk-free rate serves as the foundation for forward rate calculation, as it represents the theoretical rate of return on an investment with no risk of default.
Question 6: How can forward rate calculation be used in practice?
Answer: Forward rate calculation finds applications in various financial contexts, such as pricing interest rate derivatives, managing interest rate risk, and making informed investment decisions.
In summary, these FAQs have addressed key aspects of forward rate calculation using discount factors, providing a deeper understanding of the concepts involved and their practical applications. Moving forward, we will explore advanced topics and techniques related to forward rate calculation, enabling readers to further enhance their knowledge and skills in this area.
Transition: Delving deeper into forward rate calculation, the next section will discuss the different types of forward rate agreements and their significance in financial markets.
Tips for Calculating Forward Rates Using Discount Factors
This section provides practical tips to enhance the accuracy and efficiency of forward rate calculation using discount factors.
Tip 1: Understand the underlying concepts
Grasp the principles of time value of money, compounding, and the relationship between spot rates and forward rates.
Tip 2: Choose appropriate discount factors
Select discount factors that align with the specific cash flow and time period being considered.
Tip 3: Consider compounding effects
Account for the exponential growth of investments over time, which can significantly impact forward rate calculations.
Tip 4: Incorporate inflation expectations
Adjust discount factors based on market expectations of future inflation to enhance the accuracy of forward rate estimates.
Tip 5: Utilize financial calculators or software
Leverage technology to streamline calculations and minimize errors in forward rate determination.
Tip 6: Stay updated on market trends
Monitor economic and financial news to stay informed about factors that can influence forward rates, such as central bank policies and geopolitical events.
Tip 7: Validate results using multiple methods
Cross-check forward rate calculations using different approaches to ensure accuracy and reliability.
Tip 8: Seek professional guidance when needed
Consult with financial professionals or experts for assistance with complex forward rate calculations or interpretations.
By following these tips, you can enhance the accuracy, efficiency, and overall effectiveness of your forward rate calculations using discount factors.
These tips lay the groundwork for the concluding section, which will delve into advanced techniques and applications of forward rate calculation, empowering you with the knowledge and skills to navigate complex financial markets.
Conclusion
This in-depth exploration of forward rate calculation using discount factors has illuminated key principles and practical applications. We have examined the fundamental concepts of time value of money, compounding, and the relationship between spot rates and forward rates. Moreover, we have highlighted the significance of discount factors in valuing future cash flows and determining forward rates. By understanding these concepts and applying the discussed techniques, financial professionals can enhance the accuracy and effectiveness of their forward rate calculations.
In summary, forward rate calculation using discount factors is a vital skill for navigating financial markets. It provides valuable insights into future interest rate movements, aids in investment decision-making, and facilitates risk management. By embracing the principles and techniques outlined in this article, you can unlock a deeper understanding of forward rate dynamics and position yourself for success in the ever-evolving financial landscape.