Accounting for interest rates is a crucial aspect of financial management, involving the calculation of interest charges and earnings for borrowed funds or investments. For instance, a homeowner with a mortgage needs to factor in the interest rate charged on their loan to determine their monthly payments.
Understanding how to calculate interest rates is essential for borrowers to manage their debts effectively and for investors to maximize their returns. Historically, the development of compound interest has played a significant role in financial calculations, allowing for the accumulation of interest on previously accrued interest.
In this article, we delve into the methods for calculating interest rates, exploring different formulas and scenarios that apply to various financial transactions. By providing a comprehensive guide to interest rate calculations, we aim to empower readers with the knowledge and skills to make informed financial decisions.
Accounting for Interest Rate Calculations
Understanding the key aspects of accounting for interest rate calculations is crucial for making informed financial decisions.
- Principal: The initial amount borrowed or invested.
- Rate: The percentage of interest charged or earned.
- Time: The duration for which the interest is calculated.
- Compounding: The frequency at which interest is applied to the principal.
- Simple Interest: Interest calculated only on the principal.
- Compound Interest: Interest calculated on the principal plus accrued interest.
- Annuity: A series of equal payments made at regular intervals.
- Present Value: The current worth of a future sum of money.
These aspects play a vital role in various financial transactions, such as mortgages, loans, investments, and annuities. By considering these factors, individuals and businesses can accurately calculate interest charges and earnings, allowing for effective financial planning and decision-making.
Principal
In the context of accounting for interest rate calculations, the principal refers to the initial amount borrowed or invested. It serves as the foundation upon which interest charges or earnings are calculated, significantly impacting the overall financial outcome.
- Loan Amount: In a loan agreement, the principal represents the amount borrowed from a lender, excluding any interest or fees.
- Investment Amount: When investing, the principal refers to the initial capital invested, which could be allocated to stocks, bonds, or other financial instruments.
- Present Value: In the context of annuities or future cash flows, the principal represents the present value, which is the current worth of a future sum of money.
- Down Payment: When purchasing a property or asset, the principal can also include a down payment, which reduces the amount of money borrowed.
Understanding the concept of principal is crucial for accurate interest rate calculations. By considering the initial amount borrowed or invested, individuals and businesses can effectively plan their financial obligations, returns, and long-term financial goals.
Rate
In the realm of accounting for interest rate calculations, the rate holds paramount importance, acting as the driving force behind the accrual of interest charges or earnings. It represents the percentage applied to the principal amount over a specified period, directly influencing the overall cost of borrowing or the returns on investments.
The rate serves as a critical component of interest rate calculations, determining the magnitude of interest charges or earnings. In the case of loans, a higher interest rate translates to a greater amount of interest paid over the loan term, increasing the overall cost of borrowing. Conversely, a lower interest rate results in reduced interest payments, making the loan more affordable.
Real-life examples abound, showcasing the impact of interest rates on financial transactions. Consider a loan of $10,000 with a 5% annual interest rate. Over a one-year period, the interest charges would amount to $500. If the interest rate were doubled to 10%, the interest charges would surge to $1,000 for the same period, demonstrating the direct relationship between interest rate and interest charges.
Understanding the interplay between rate and interest rate calculations is crucial for informed financial decision-making. Individuals and businesses must carefully consider the interest rate when evaluating loans, investments, and other financial commitments. By factoring in the potential impact of interest rates over the life of the agreement, they can make prudent choices that align with their financial goals.
Time
Within the context of “accounting how to calculate interest rate,” the time duration plays a pivotal role in determining the overall interest charges or earnings. It encompasses the length of the loan or investment period and can be dissected into various facets or components.
- Loan Term/Investment Period: The loan term or investment period signifies the duration for which the funds are borrowed or invested, directly impacting the total interest accrued.
- Compounding Frequency: Compounding refers to the frequency at which interest is added to the principal, influencing the overall interest earned or paid over the time duration.
- Maturity Date: The maturity date marks the end of the loan or investment period, determining when the principal and any accrued interest are due.
- Discount Period: In certain financial transactions, such as bond issuance, the discount period represents the time between the bond’s purchase and its maturity, affecting the calculation of interest payments.
These facets of time duration play a crucial role in accounting for interest rate calculations, impacting the overall cost of borrowing or the returns on investments. By considering the time value of money and the specific time-related components involved, individuals and businesses can accurately assess the financial implications and make informed decisions.
Compounding
Regarding “accounting how to calculate interest rate,” compounding holds great significance in determining the trajectory of interest accrual over time. It signifies the frequency at which interest is added to the principal, shaping the overall interest earned or paid, with several crucial facets to consider:
- Annual Compounding: Interest is added to the principal once a year, resulting in a straightforward calculation of interest earned or paid.
- Semi-annual Compounding: Interest is added twice a year, leading to a higher effective interest rate compared to annual compounding.
- Quarterly Compounding: Interest is added four times a year, further increasing the effective interest rate and the overall interest earned or paid.
- Continuous Compounding: Interest is added continuously, resulting in the highest effective interest rate and maximizing the interest earned or paid.
Understanding the concept of compounding is vital for accurate interest rate calculations, as it directly influences the total interest accrued or paid over the life of a loan or investment. By considering the frequency of compounding, individuals and businesses can make informed decisions, comparing different financial products and strategies to optimize their financial outcomes.
Simple Interest
In the realm of “accounting how to calculate interest rate,” simple interest holds a fundamental position, representing a straightforward method of calculating interest charges or earnings based solely on the principal amount. Unlike compound interest, simple interest does not consider the accrual of interest on previously earned interest, resulting in a linear growth of interest over time.
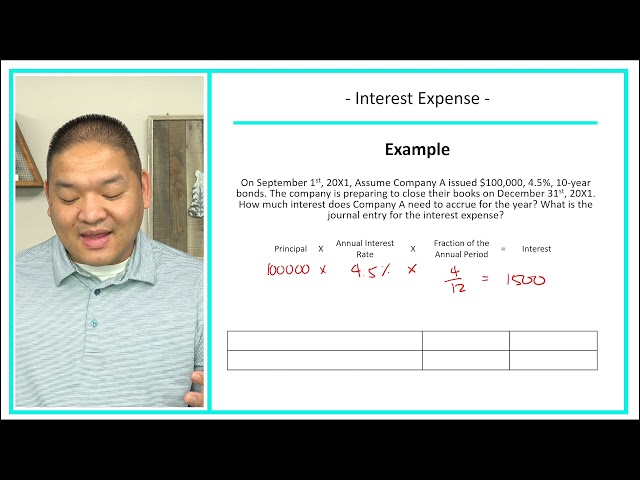
- Formula: Simple interest is calculated using the formula I = P R T, where I represents the interest, P is the principal, R is the annual interest rate, and T is the time period.
- Fixed Interest Rate: Simple interest employs a fixed interest rate that remains constant throughout the loan or investment period, ensuring predictable interest payments or earnings.
- Non-compounding: As mentioned earlier, simple interest does not involve compounding, meaning interest is not added to the principal, leading to a consistent interest amount over the time duration.
- Short-term Applicability: Simple interest is commonly used in short-term financial transactions, such as short-term loans or savings accounts, where the time period is relatively short, and the impact of compounding is minimal.
Understanding the nuances of simple interest is crucial for accurate interest rate calculations and informed financial decision-making. Its straightforward formula and predictable nature make it a suitable choice for certain financial scenarios, particularly those involving shorter time periods or fixed interest rates.
Compound Interest
Within the realm of “accounting how to calculate interest rate,” compound interest emerges as a cornerstone concept. Unlike simple interest, which solely considers the principal amount, compound interest incorporates the accrual of interest on previously earned interest, leading to exponential growth over time. This intricate relationship between compound interest and interest rate calculations warrants careful examination.
Compound interest serves as a critical component of interest rate calculations, particularly in long-term financial transactions. Its inclusion significantly alters the calculation process, as it takes into account the snowball effect of interest accumulating on interest. This characteristic makes compound interest a powerful force, capable of magnifying returns on investments or amplifying the cost of borrowing over extended periods.
Real-life examples abound, showcasing the profound impact of compound interest. Consider an investment of $1,000 earning 5% annual interest compounded annually. After one year, the interest earned is $50. However, in the second year, interest is calculated not only on the initial $1,000 but also on the $50 earned in the first year, resulting in a slightly higher interest amount of $52.50. This process continues each year, with interest snowballing due to compounding, leading to a substantial nest egg over the long term.
Understanding the mechanics of compound interest is not just an academic pursuit; it holds practical significance for financial decision-making. Individuals planning for retirement or long-term investments can harness the power of compound interest to maximize their returns. Conversely, borrowers need to be aware of the potential impact of compound interest on their loan obligations, ensuring they can manage the growing interest charges over time.
Annuity
Within the domain of “accounting how to calculate interest rate,” annuities hold a prominent position, representing a series of equal payments made at regular intervals. They play a critical role in various financial transactions, necessitating a thorough understanding of their components and implications.
- Installment Loans: Many loans, such as mortgages and auto loans, are structured as annuities, with regular payments that cover both principal and interest.
- Retirement Planning: Annuities can serve as a retirement income stream, providing a steady flow of payments during retirement years.
- Investment Vehicles: Certain investment vehicles, such as structured notes and some types of bonds, make periodic payments to investors.
- Present Value: The present value of an annuity represents the current worth of a series of future payments, discounted back to the present using an appropriate interest rate.
Understanding the concept of annuities is crucial for accurately calculating interest rates in various financial scenarios. Whether it’s determining the interest portion of loan payments, valuing retirement income streams, or assessing the present value of future cash flows, annuities play a vital role in interest rate calculations, empowering individuals and businesses to make informed financial decisions.
Present Value
Within the context of “accounting how to calculate interest rate,” present value holds a crucial position, representing the current worth of a future sum of money, discounted back to the present using an appropriate interest rate. This concept serves as a cornerstone in various financial calculations, impacting investment decisions, loan evaluations, and more.
- Discounted Cash Flow: Present value plays a vital role in discounted cash flow analysis, a method used to assess the value of future cash flows by considering their present worth.
- Investment Appraisal: When evaluating investment opportunities, present value helps determine the net present value, indicating the current worth of future cash inflows and outflows, aiding in decision-making.
- Loan Valuation: In the context of loans, present value calculations are used to determine the loan’s net present value, considering both the principal and interest payments, to assess its overall cost.
- Bond Pricing: Present value is essential in bond pricing, as it helps determine the fair value of a bond by considering its future coupon payments and maturity value, discounted back to the present.
In summary, present value serves as a fundamental concept in “accounting how to calculate interest rate,” enabling individuals and businesses to make informed financial decisions. By understanding the present value of future cash flows, they can effectively evaluate investments, assess loans, price bonds, and conduct various other financial calculations.
FAQs on Accounting for Interest Rate Calculations
The following frequently asked questions (FAQs) provide concise answers to common queries and clarify aspects of “accounting how to calculate interest rate.” These questions anticipate reader concerns and offer insights into the core concepts, helping readers solidify their understanding.
Question 1: What is the formula for calculating simple interest?
The formula for simple interest is I = P R T, where I represents the interest, P is the principal amount, R is the annual interest rate, and T is the time period.
Question 6: How is the present value of an annuity calculated?
The present value of an annuity is calculated by multiplying the periodic payment by the present value factor, which is determined using the interest rate and the number of payment periods.
These FAQs provide a concise overview of the key concepts surrounding “accounting how to calculate interest rate.” They address common questions and offer clear explanations, enabling readers to grasp the fundamentals more effectively.
In the next section, we will delve deeper into the nuances of interest rate calculations, exploring advanced concepts and their practical applications in various financial scenarios.
Tips for Accounting Interest Rate Calculations
To ensure accurate and efficient interest rate calculations, consider implementing the following tips:
Tip 1: Identify the Type of Interest: Determine whether simple or compound interest applies to your calculation, as the formulas and outcomes differ.
Tip 2: Calculate the Time Period: Accurately determine the time period over which interest accrues, considering factors like loan terms or investment horizons.
Tip 3: Use the Correct Interest Rate: Ensure you apply the appropriate interest rate, whether it’s an annual percentage rate (APR) or a daily rate.
Tip 4: Consider Compounding Frequency: If dealing with compound interest, determine the compounding frequency, as it significantly impacts the .
Tip 5: Convert Rates: If necessary, convert interest rates between different time periods (e.g., annual to monthly) to align with the calculation requirements.
Tip 6: Use Financial Calculators: Utilize financial calculators or software to streamline calculations, minimize errors, and enhance efficiency.
Tip 7: Verify Results: Always double-check your calculations using different methods or tools to ensure accuracy and avoid costly errors.
By following these tips, you can enhance the accuracy and efficiency of your interest rate calculations, leading to more informed financial decisions.
In the concluding section, we will explore advanced techniques for interest rate calculations, enabling you to tackle complex financial scenarios with confidence.
Conclusion
This comprehensive exploration of “accounting how to calculate interest rate” has illuminated the fundamental concepts, formulas, and techniques involved in this critical aspect of financial management. We have emphasized the importance of understanding the types of interest (simple and compound), calculating time periods accurately, and applying the correct interest rates.
Moreover, we have highlighted the significance of considering compounding frequency, converting rates between different time periods, and utilizing financial calculators for efficiency and accuracy. By following the tips and insights provided, individuals and businesses can confidently tackle interest rate calculations in various financial scenarios.