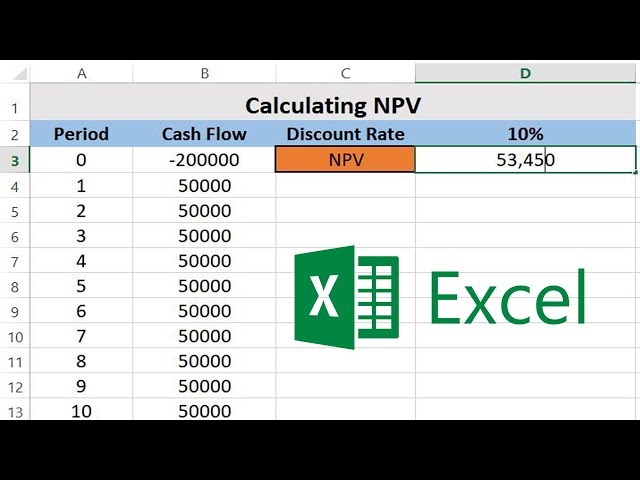
How to calculate net present value (NPV) discount rate in Excel is a crucial financial analysis technique used to assess the profitability of potential investments. NPV is the difference between the present value of cash inflows and outflows over a period of time. The discount rate is a key factor in calculating NPV, as it represents the rate at which future cash flows are discounted back to their present value.
Calculating NPV and discount rate in Excel is essential for evaluating the financial viability of projects and making informed investment decisions. Understanding the process and factors involved in these calculations can provide valuable insights for businesses and individuals seeking to optimize their financial outcomes.
This article will delve into the steps involved in calculating NPV discount rate in Excel, including the formula and considerations for determining an appropriate discount rate. We will also explore the significance of NPV analysis in investment decision-making and highlight practical applications in various industries.
How to Calculate NPV Discount Rate in Excel
Understanding the key aspects of calculating NPV discount rate in Excel is crucial for making sound investment decisions. These aspects encompass various dimensions related to the calculation process and its implications.
- Formula: NPV = -Initial Investment + Sum of Present Value of Future Cash Flows
- Discount Rate: The rate used to discount future cash flows back to their present value
- Project Cash Flows: The estimated inflows and outflows of cash over the project’s life
- Time Value of Money: The concept that money today is worth more than the same amount in the future
- Risk and Uncertainty: The consideration of potential risks and uncertainties that may affect future cash flows
- Excel Functions: Utilizing Excel functions such as NPV() and IRR() for efficient calculations
- Interpretation: Analyzing the NPV result to determine the project’s profitability
- Sensitivity Analysis: Testing the NPV’s sensitivity to changes in assumptions and variables
These key aspects are interconnected and play a vital role in accurately calculating NPV discount rate in Excel. By considering each aspect thoroughly, businesses and individuals can enhance their financial decision-making and maximize investment returns. For example, understanding the time value of money highlights the importance of discounting future cash flows to their present value, ensuring a fair comparison of investment options with varying time horizons. Additionally, performing sensitivity analysis allows investors to assess the impact of potential changes in assumptions, such as discount rate or project cash flows, on the NPV, providing valuable insights for risk management and contingency planning.
Formula
The formula for calculating Net Present Value (NPV) forms the cornerstone of the “how to calculate NPV discount rate in Excel” process. It encapsulates the fundamental principle that the value of future cash flows must be discounted back to their present value to accurately assess the profitability of a potential investment.
- Initial Investment: The initial outlay required to undertake the investment project.
- Sum of Present Value of Future Cash Flows: The discounted value of all future cash inflows and outflows over the project’s lifetime.
- Discount Rate: The rate used to discount future cash flows, typically based on the Weighted Average Cost of Capital (WACC) or an appropriate risk-adjusted rate.
- Time Horizon: The total period over which the project’s cash flows are to be considered.
Understanding the intricate relationship between these components is crucial for accurate NPV calculations. The initial investment represents the upfront cost of the project, while the sum of present value of future cash flows captures the time value of money and the impact of the discount rate in determining the project’s profitability. Selecting an appropriate discount rate is critical as it reflects the risk and opportunity cost of capital, influencing the present value of future cash flows and ultimately the NPV.
Discount Rate
The discount rate plays a pivotal role in calculating NPV. It represents the cost of capital or the minimum acceptable rate of return required by investors. The discount rate is used to determine the present value of future cash flows, which are then summed up to calculate the NPV. A higher discount rate will result in a lower NPV, and vice versa. Therefore, the choice of an appropriate discount rate is critical in evaluating investment opportunities.
In practice, the discount rate is often derived from the weighted average cost of capital (WACC) or a risk-adjusted rate. The WACC considers the cost of both debt and equity financing, weighted by their respective proportions in the capital structure. Alternatively, a risk-adjusted rate can be used, which incorporates an additional premium to reflect the project’s specific risk profile.
Understanding the relationship between the discount rate and NPV is essential for making informed investment decisions. By manipulating the discount rate, investors can assess the sensitivity of the NPV to changes in the cost of capital or risk. This analysis helps in identifying projects that are robust to changes in the economic environment or the company’s financial situation.
Project Cash Flows
Project cash flows are the backbone of NPV calculations, representing the lifeblood of any investment or project. Understanding and accurately forecasting these cash flows are crucial for reliable NPV assessments.
- Operating Cash Flows: Cash generated from the project’s primary operations, including revenue, expenses, and working capital changes.
- Investment Cash Flows: Outlays and receipts related to capital expenditures, such as purchasing equipment or expanding facilities.
- Financing Cash Flows: Flows associated with debt or equity financing, including loan payments, interest expenses, and dividends.
- Terminal Cash Flow: The estimated cash flow at the end of the project’s life, often representing the salvage value of assets or proceeds from liquidation.
Accurately estimating project cash flows requires careful consideration of historical data, industry benchmarks, and assumptions about future market conditions. Overestimating cash flows can lead to an inflated NPV, while underestimating them can result in a conservative or even negative NPV. Therefore, it is essential to adopt a realistic and well-informed approach when projecting cash flows for NPV calculations.
Time Value of Money
In the realm of NPV calculations, the concept of Time Value of Money (TVM) is paramount. TVM acknowledges the fundamental principle that the value of money today outweighs its equivalent value in the future.
- Present Value: The current worth of a future sum of money, discounted at a specific interest rate. Understanding present value is crucial for comparing future cash flows to current investments.
- Future Value: The value of an investment at a specified future date, considering the effects of compounding interest. This concept helps determine the potential growth of investments over time.
- Discount Rate: The interest rate used to calculate the present value of future cash flows. The discount rate significantly influences the NPV and should be carefully determined.
- Compounding: The process of earning interest on both the principal amount and the accumulated interest. Compounding can exponentially increase the value of investments over time.
Comprehending TVM’s facets unveils the significance of considering the time value of money when evaluating investments. By incorporating TVM principles into NPV analysis, investors can make informed decisions that account for the impact of time on the value of money.
Risk and Uncertainty
When calculating NPV, it is imperative to consider the inherent risks and uncertainties that may influence future cash flows. These factors can significantly impact the accuracy of NPV calculations and should be carefully evaluated to make informed investment decisions.
- Economic Conditions: External economic factors, such as inflation, recession, and changes in interest rates, can significantly impact project cash flows. Accurately forecasting these conditions is crucial for realistic NPV calculations.
- Market Risk: Shifts in market demand, competition, and technological advancements can affect the revenue and profitability of a project. Understanding market dynamics and potential disruptions is essential for mitigating.
- Operational Risks: Internal factors, such as operational inefficiencies, supply chain disruptions, and regulatory changes, can disrupt project execution and impact cash flows. Identifying and addressing these risks is crucial for ensuring project success.
- Financial Risk: Fluctuations in exchange rates, interest rates, and credit availability can affect project costs and financing options. Managing financial risks through hedging and diversification strategies is essential for safeguarding project viability.
By incorporating risk and uncertainty considerations into NPV calculations, investors can make more informed decisions that account for potential challenges and opportunities. Sensitivity analysis and scenario planning techniques can help assess the impact of various risk factors on project outcomes, enabling investors to make robust investment choices.
Excel Functions
Excel functions play a pivotal role in streamlining the calculation of NPV and discount rate in Excel. These functions provide convenient and efficient ways to automate calculations, reducing the risk of errors and saving valuable time.
- NPV Function
The NPV() function directly calculates the net present value of a series of cash flows. It takes into account the initial investment and the discount rate to determine the project’s profitability.
- IRR Function
The IRR() function calculates the internal rate of return, which is the discount rate that makes the NPV of a project equal to zero. It is useful for determining the minimum acceptable rate of return for an investment.
- Combination of NPV and IRR Functions
Combining the NPV() and IRR() functions allows for a comprehensive analysis of investment opportunities. By comparing the NPV and IRR, investors can gain insights into the project’s profitability and risk profile.
- Sensitivity Analysis with Excel Functions
Excel functions enable sensitivity analysis, which involves changing input variables to observe the impact on NPV and IRR. This analysis helps investors assess the robustness of their investment decisions.
In conclusion, Excel functions like NPV() and IRR() are essential tools for efficient and accurate NPV and discount rate calculations. They simplify complex calculations, enhance decision-making, and provide valuable insights into investment opportunities.
Interpretation
Interpreting the NPV result is a critical component of “how to calculate NPV discount rate in Excel” because it provides insights into the project’s profitability and viability. The NPV calculation considers the time value of money and incorporates cash flows over the project’s life, making it a comprehensive measure of a project’s worth. By analyzing the NPV result, decision-makers can determine whether a project is financially feasible and aligns with their investment objectives.
In practice, a positive NPV indicates that the project is expected to generate a return that exceeds the cost of capital. This implies that the project is likely to be profitable and may be considered for further evaluation and investment. Conversely, a negative NPV suggests that the project is not expected to generate sufficient returns to cover the cost of capital, indicating that it may not be a viable investment option.
Understanding the interpretation of NPV results is essential for making informed investment decisions. It allows businesses and individuals to compare different project proposals, prioritize investments, and allocate resources effectively. Moreover, by considering the NPV result in conjunction with other financial metrics and qualitative factors, decision-makers can gain a comprehensive understanding of the project’s potential risks, rewards, and overall alignment with strategic objectives.
Sensitivity Analysis
Sensitivity analysis is an indispensable aspect of NPV calculations, as it allows investors to assess the robustness of their investment decisions in the face of changing assumptions and variables. By examining how the NPV responds to variations in key parameters, investors can gain valuable insights into the project’s risk profile and potential outcomes.
- Discount Rate Sensitivity: Testing the impact of different discount rates on the NPV provides insights into the project’s sensitivity to the cost of capital and market conditions.
- Cash Flow Sensitivity: Analyzing the NPV’s response to changes in cash flow projections helps investors understand the impact of variations in revenue, expenses, and other cash flow components.
- Project Life Sensitivity: Examining the NPV over different project durations sheds light on the project’s sensitivity to its operational timeline and potential end-of-life scenarios.
- Input Variable Sensitivity: Sensitivity analysis can be applied to any input variable used in the NPV calculation, allowing investors to assess the impact of changes in assumptions such as inflation, tax rates, or market growth.
By conducting sensitivity analysis, investors can identify critical factors that significantly affect the NPV, enabling them to make informed decisions about project selection, risk mitigation, and resource allocation. This analysis helps investors navigate uncertainty, prioritize investments, and increase the likelihood of achieving their financial goals.
FAQs on Calculating NPV Discount Rate in Excel
The following FAQs are designed to clarify common questions and provide additional insights on how to calculate NPV discount rate in Excel:
Question 1: What is the purpose of calculating NPV discount rate in Excel?
Answer: Calculating NPV discount rate in Excel helps determine the present value of future cash flows, considering the time value of money. This metric is crucial for evaluating the profitability and viability of investment projects.
Question 2: What factors should be considered when selecting a discount rate?
Answer: The discount rate should reflect the project’s risk profile, cost of capital, and the prevailing market conditions. Common methods for determining the discount rate include using the weighted average cost of capital (WACC) or a risk-adjusted rate.
Question 3: How do I incorporate project cash flows into the NPV calculation?
Answer: Project cash flows include operating, investment, and financing flows. These cash flows are estimated over the project’s lifespan and discounted back to their present value using the selected discount rate.
Question 4: What is the significance of sensitivity analysis in NPV calculations?
Answer: Sensitivity analysis involves testing the NPV’s response to changes in key assumptions and variables. It helps identify critical factors influencing the project’s profitability and allows investors to make informed decisions in the face of uncertainty.
Question 5: How can I interpret the NPV result to make investment decisions?
Answer: A positive NPV indicates that the project is expected to generate returns exceeding the cost of capital, suggesting its potential profitability. Conversely, a negative NPV suggests that the project may not be financially viable and should be carefully considered.
Question 6: Are there any Excel functions available to simplify NPV calculations?
Answer: Yes, Excel provides the NPV() function to directly calculate net present value. Additionally, the IRR() function can be used to determine the project’s internal rate of return, which provides further insights into its profitability.
These FAQs provide a concise overview of the key aspects of calculating NPV discount rate in Excel. Understanding these concepts is essential for making informed investment decisions and maximizing project returns. The following section will delve deeper into the practical applications of NPV analysis in various industries, showcasing its relevance and versatility in financial decision-making.
Tips for Calculating NPV Discount Rate in Excel
This section provides practical tips to guide you through the process of calculating NPV discount rate in Excel, ensuring accuracy and efficiency.
Tip 1: Gather Accurate Project Information: Before initiating your calculation, ensure you have gathered all relevant project data, including projected cash flows, initial investment, and project duration.
Tip 2: Determine an Appropriate Discount Rate: The discount rate should reflect the project’s risk and the cost of capital. Consider using the weighted average cost of capital (WACC) or a risk-adjusted rate.
Tip 3: Structure Cash Flows in a Clear Format: Organize your cash flow data in an Excel spreadsheet, ensuring each cash flow is identified by its timing and amount.
Tip 4: Use the NPV Function: Excel’s NPV() function simplifies the calculation. Enter the discount rate, cash flow values, and initial investment as arguments to obtain the NPV.
Tip 5: Conduct Sensitivity Analysis: Test the robustness of your NPV calculation by varying critical assumptions, such as the discount rate and cash flow estimates.
Tip 6: Consider Inflation: If the project spans multiple years, adjust cash flows for inflation to accurately capture the time value of money.
Tip 7: Interpret Results Carefully: Analyze the NPV in conjunction with other financial metrics and qualitative factors to make well-informed investment decisions.
Tip 8: Utilize Excel’s Help Resources: Excel provides extensive help documentation and tutorials on NPV calculations. Refer to these resources for additional guidance.
By following these tips, you can enhance the accuracy and efficiency of your NPV discount rate calculations in Excel, leading to more informed investment decisions and improved project outcomes.
The insights gained from these tips will serve as a solid foundation as we delve into the practical applications of NPV analysis in various industries, showcasing its versatility and importance in financial decision-making.
Conclusion
To conclude, understanding “how to calculate NPV discount rate in Excel” is crucial for making informed investment decisions. This method allows for the evaluation of a project’s profitability by considering the time value of money and future cash flows. Throughout this article, we have explored key concepts such as discount rate determination, project cash flow analysis, and sensitivity analysis.
Two main points to emphasize are: (1) selecting an appropriate discount rate is essential, as it directly impacts the NPV calculation, and (2) sensitivity analysis provides valuable insights into the NPV’s behavior under varying assumptions. These interconnected aspects enable investors to assess the risks and rewards of investment opportunities more accurately.
Recognizing the importance of NPV analysis empowers individuals and organizations to make judicious financial decisions. By incorporating these concepts into investment evaluations, we can increase the likelihood of successful project outcomes and maximize returns.