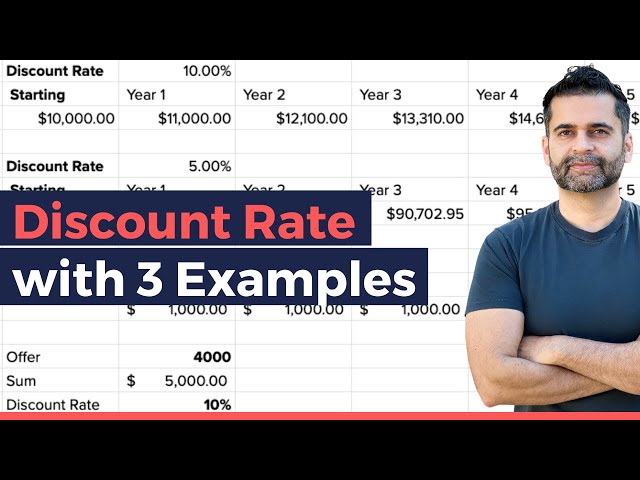
The discount rate formula for net present value (NPV) in Excel is a mathematical equation used to calculate the present value of a series of future cash flows. It is a critical tool in capital budgeting and investment analysis to determine the profitability of a project.
The formula, which factors in the time value of money, is particularly relevant to long-term investments. For instance, a project that generates positive cash flows in the future may not be as valuable as it appears if the discount rate is high, indicating a higher opportunity cost of capital. Historically, the discount rate concept emerged from the idea of compound interest, which recognizes that money grows exponentially over time.
This article delves into the components of the discount rate formula, its significance, and its application in Excel for evaluating investment opportunities.
Discount Rate Formula for NPV in Excel
To effectively evaluate investment opportunities, understanding the essential aspects of the discount rate formula for NPV in Excel is crucial. These aspects encompass fundamental concepts that shape the formula’s application and interpretation.
- Time Value of Money
- Future Cash Flows
- Discount Rate
- Present Value
- Net Present Value
- Capital Budgeting
- Investment Analysis
- IRR and Payback Period
The discount rate, a key determinant of NPV, reflects the opportunity cost of capital and the risk associated with the investment. It influences the present value of future cash flows, which are discounted to account for the time value of money. NPV, by aggregating these discounted cash flows, provides a comprehensive measure of a project’s profitability and is widely used in capital budgeting and investment analysis alongside other metrics like IRR and payback period.
Time Value of Money
The concept of Time Value of Money (TVM) underpins the Discount Rate Formula for Net Present Value (NPV) in Excel. TVM recognizes that the value of money today is not equivalent to its value in the future due to the potential for growth through investment or the impact of inflation. This principle forms the foundation for understanding the role of the discount rate in NPV calculations.
The discount rate, often derived from the cost of capital or hurdle rate, is applied to future cash flows to determine their present value. This adjustment reflects the time value of money and allows for the comparison of cash flows occurring at different points in time. By discounting future cash flows, the NPV formula brings them to a common reference point, enabling the assessment of a project’s overall profitability.
In practice, TVM plays a crucial role in evaluating long-term investments, such as infrastructure projects or capital equipment purchases. By incorporating the discount rate, the NPV formula considers the time value of money and helps decision-makers determine if the potential returns justify the investment. This understanding is critical for making informed financial decisions and ensuring efficient allocation of resources.
Future Cash Flows
Future Cash Flows are an integral component of the Discount Rate Formula for Net Present Value (NPV) in Excel. They represent the anticipated stream of income or expenses over a specified period, and their accurate estimation is crucial for effective investment analysis.
- Types of Cash Flows
Future cash flows can be classified as operating, investing, or financing activities, each with unique characteristics and implications for NPV calculations. - Time Horizon
The time horizon of future cash flows is a critical consideration, as the timing and duration of inflows and outflows impact their present value. - Risk and Uncertainty
Future cash flows are inherently uncertain, and the discount rate should reflect the perceived risk associated with these cash flows. - Growth and Inflation
The potential for growth or inflation can affect the real value of future cash flows, which should be considered when determining the appropriate discount rate.
In the context of NPV calculations, future cash flows are discounted using the discount rate to determine their present value. This adjustment brings cash flows from different points in time to a common reference point, enabling a comprehensive assessment of a project’s profitability. Understanding the various facets of future cash flows and their relationship with the discount rate is essential for accurate NPV calculations and informed investment decisions.
Discount Rate
The Discount Rate stands as a pivotal concept in the Discount Rate Formula for Net Present Value (NPV) in Excel, playing a central role in investment analysis and capital budgeting. It represents the rate at which future cash flows are discounted to determine their present value, providing a basis for evaluating the profitability of long-term projects.
Within the Discount Rate Formula for NPV in Excel, the Discount Rate serves as a critical component, acting as the benchmark against which future cash flows are adjusted. This adjustment reflects the time value of money, acknowledging that the value of a dollar today is not equivalent to its value in the future due to potential growth or inflation. By incorporating the Discount Rate, the formula effectively translates future cash flows to a common reference point, enabling a meaningful comparison of projects with varying cash flow patterns.
In practical applications, the Discount Rate is often derived from the weighted average cost of capital (WACC), which considers both debt and equity financing costs. It can also be influenced by factors such as inflation, risk-free rates, and market conditions. Understanding the relationship between the Discount Rate and the Discount Rate Formula for NPV in Excel is essential for accurate project evaluation and informed decision-making.
In summary, the Discount Rate is a crucial element of the Discount Rate Formula for NPV in Excel, serving as the benchmark for discounting future cash flows and assessing project profitability. Its proper application requires careful consideration of factors such as the time value of money, WACC, and market conditions. By comprehending this relationship, financial analysts and investors can make informed investment decisions and optimize long-term project outcomes.
Present Value
In the realm of financial analysis and investment decision-making, Present Value (PV) holds significant importance, particularly in the context of the Discount Rate Formula for Net Present Value (NPV) in Excel. PV represents the current worth of a future sum of money, adjusted to reflect the time value of money and the applicable discount rate. This concept is fundamental to capital budgeting and project evaluation, where long-term cash flows are assessed for their profitability.
Within the Discount Rate Formula for NPV in Excel, PV plays a pivotal role as the discounted value of future cash flows. The discount rate, often derived from the cost of capital, represents the rate at which future cash flows are adjusted to account for their present value. By incorporating PV into the formula, analysts can compare projects with varying cash flow patterns and time horizons, enabling informed investment decisions.
The relationship between Present Value and the Discount Rate Formula for NPV in Excel is reciprocal. On the one hand, the discount rate directly influences the PV of future cash flows. A higher discount rate results in a lower PV, and vice versa. This adjustment reflects the opportunity cost of capital and the risk associated with the investment. On the other hand, the PV of future cash flows, when summed up, determines the NPV of a project. A positive NPV indicates a profitable investment, while a negative NPV suggests otherwise.
In practice, understanding the connection between Present Value and the Discount Rate Formula for NPV in Excel is crucial for evaluating long-term investments, such as infrastructure projects, equipment purchases, or real estate developments. Financial analysts use this formula to assess the viability of potential projects, compare alternative investment options, and make informed recommendations. By considering the time value of money and the appropriate discount rate, decision-makers can optimize resource allocation and maximize returns.
Net Present Value
Within the realm of financial analysis and investment decision-making, Net Present Value (NPV) stands as a critical metric used to evaluate the profitability of long-term projects and investments. Its calculation relies heavily on the discount rate formula for NPV in Excel, which incorporates the time value of money and the applicable discount rate to determine the present value of future cash flows.
The discount rate, often derived from the weighted average cost of capital (WACC), serves as the benchmark against which future cash flows are discounted to their present value. This adjustment reflects the opportunity cost of capital and the risk associated with the investment. By incorporating the discount rate into the formula, analysts can compare projects with varying cash flow patterns and time horizons, enabling informed investment decisions.
In practice, NPV is widely used in capital budgeting, where long-term projects are assessed for their potential profitability. A positive NPV indicates that the project is expected to generate positive returns, while a negative NPV suggests that the project may not be financially viable. Financial analysts use the discount rate formula for NPV in Excel to calculate the NPV of potential projects, compare alternative investment options, and make informed recommendations.
Understanding the connection between NPV and the discount rate formula for NPV in Excel is crucial for making sound investment decisions. By considering the time value of money and the appropriate discount rate, decision-makers can optimize resource allocation and maximize returns. This understanding empowers financial analysts and investors to evaluate long-term projects effectively, mitigate risks, and achieve their financial goals.
Capital Budgeting
Capital budgeting is a critical process in financial management that involves evaluating and selecting long-term investment projects. The discount rate formula for Net Present Value (NPV) in Excel plays a central role in capital budgeting by enabling the comparison of alternative investment options and determining their potential profitability.
The discount rate, often derived from the weighted average cost of capital (WACC), is a crucial component of the discount rate formula for NPV in Excel. It represents the minimum acceptable rate of return required by investors and reflects the opportunity cost of capital. By incorporating the discount rate into the formula, analysts can adjust future cash flows to their present value, allowing for the comparison of projects with varying cash flow patterns and time horizons.
In practice, the discount rate formula for NPV in Excel is widely used in capital budgeting to evaluate projects such as infrastructure development, equipment purchases, or research and development initiatives. By calculating the NPV of each project, analysts can determine which investments are expected to generate positive returns and align with the organization’s strategic objectives. This understanding helps decision-makers allocate resources effectively, prioritize projects with the highest potential returns, and mitigate financial risks.
In summary, the discount rate formula for NPV in Excel is an essential tool for capital budgeting, providing a framework for evaluating long-term investment projects and making informed decisions. By incorporating the discount rate into the formula, analysts can adjust future cash flows to their present value, compare alternative investment options, and determine their potential profitability. This understanding empowers financial managers to optimize resource allocation, maximize returns, and achieve the organization’s long-term financial goals.
Investment Analysis
Investment analysis is a critical component of the discount rate formula for Net Present Value (NPV) in Excel. It involves evaluating the potential profitability and risk associated with long-term investment projects. By incorporating the discount rate into the formula, analysts can adjust future cash flows to their present value, enabling the comparison of alternative investment options and informed decision-making.
In practice, investment analysis within the discount rate formula for NPV in Excel is used to assess a wide range of projects, including infrastructure development, equipment purchases, and research and development initiatives. Analysts consider factors such as the project’s expected cash flows, the timing of these cash flows, and the associated risks. By calculating the NPV of each project, decision-makers can determine which investments are expected to generate positive returns and align with the organization’s strategic objectives.
The practical applications of this understanding extend to various industries and sectors. For example, in the energy sector, investment analysis using the discount rate formula for NPV in Excel helps evaluate renewable energy projects, such as solar and wind farms. Analysts consider factors such as the project’s upfront costs, ongoing operating expenses, and expected energy production to determine the project’s financial viability. Similarly, in the healthcare sector, investment analysis is used to assess the profitability of new medical technologies and treatments, considering factors such as research and development costs, regulatory approvals, and market demand.
In summary, investment analysis is a critical component of the discount rate formula for NPV in Excel, providing a framework for evaluating long-term investment projects and making informed decisions. By incorporating the discount rate into the formula, analysts can adjust future cash flows to their present value, compare alternative investment options, and determine their potential profitability. This understanding empowers financial managers to optimize resource allocation, maximize returns, and achieve the organization’s long-term financial goals.
IRR and Payback Period
Internal Rate of Return (IRR) and Payback Period are two widely used metrics in capital budgeting and investment analysis. They provide valuable insights into the profitability and liquidity of long-term projects, and their relationship with the discount rate formula for Net Present Value (NPV) in Excel is crucial for informed decision-making.
IRR represents the discount rate at which the NPV of a project equals zero. It indicates the annualized rate of return an investment is expected to generate. Payback Period, on the other hand, measures the time it takes for an investment to generate enough cash flows to cover its initial cost. Both IRR and Payback Period complement the NPV analysis by providing additional perspectives on a project’s financial viability.
The discount rate used in the NPV formula directly influences the IRR and Payback Period calculations. A higher discount rate will result in a lower IRR and a longer Payback Period. Conversely, a lower discount rate will lead to a higher IRR and a shorter Payback Period. This relationship highlights the importance of carefully considering the appropriate discount rate when evaluating projects using these metrics.
In practical applications, IRR is particularly useful for comparing projects with different risk profiles and time horizons. Projects with a higher IRR are generally considered more desirable, as they promise a higher rate of return. Payback Period, on the other hand, is more focused on the liquidity of an investment. Projects with a shorter Payback Period are often preferred, as they provide a quicker return on investment. By considering both IRR and Payback Period alongside NPV, investors can make informed decisions that align with their risk tolerance and investment goals.
FAQs on Discount Rate Formula for NPV in Excel
This FAQ section addresses common questions and clarifies important aspects related to the discount rate formula for Net Present Value (NPV) in Excel. These questions aim to provide a deeper understanding of the formula’s components, applications, and implications.
Question 1: What is the purpose of the discount rate in the NPV formula?
The discount rate represents the time value of money and is used to adjust future cash flows to their present value. It reflects the opportunity cost of capital and the risk associated with the investment.
Question 2: How is the discount rate determined?
The discount rate can be derived from various sources, such as the weighted average cost of capital (WACC), the risk-free rate, or industry benchmarks. The appropriate discount rate depends on the specific project and its risk profile.
Question 3: What are the applications of the NPV formula?
The NPV formula is widely used in capital budgeting and investment analysis to evaluate the profitability of long-term projects. It helps decision-makers compare alternative investment options and make informed choices.
Question 4: How does the discount rate affect the NPV calculation?
A higher discount rate results in a lower NPV, while a lower discount rate leads to a higher NPV. This is because the discount rate adjusts future cash flows to their present value, and a higher discount rate places less weight on future cash flows.
Question 5: What is the relationship between NPV and IRR?
IRR (Internal Rate of Return) is the discount rate at which the NPV of a project equals zero. Projects with a higher IRR are generally considered more desirable, as they promise a higher rate of return.
Question 6: How can I use Excel to calculate NPV?
Excel provides the NPV function, which allows users to easily calculate the NPV of a project. The function takes the discount rate, a range of cash flows, and an optional date as arguments.
These FAQs provide essential insights into the discount rate formula for NPV in Excel. By understanding the components and applications of this formula, readers can confidently evaluate investment opportunities and make informed financial decisions.
In the next section, we will delve deeper into the practical applications of the discount rate formula for NPV in Excel, exploring real-world examples and case studies to illustrate its use in capital budgeting and investment analysis.
Tips for Applying the Discount Rate Formula for NPV in Excel
To ensure accurate and effective application of the discount rate formula for Net Present Value (NPV) in Excel, consider the following tips:
Tip 1: Determine the Appropriate Discount Rate: Carefully determine the discount rate based on the project’s risk profile and opportunity cost of capital.
Tip 2: Consider Inflation: Adjust the discount rate for inflation to account for the impact of rising prices on future cash flows.
Tip 3: Use Consistent Time Periods: Ensure that all cash flows are expressed using the same time period (e.g., annual, quarterly) for accurate discounting.
Tip 4: Include All Relevant Cash Flows: Consider both positive and negative cash flows, including initial investment, operating expenses, and salvage value.
Tip 5: Use Sensitivity Analysis: Perform sensitivity analysis by varying the discount rate and key assumptions to assess the impact on NPV.
Tip 6: Compare NPV to Other Metrics: Evaluate NPV alongside other metrics such as IRR and Payback Period for a comprehensive investment analysis.
Tip 7: Use Excel Functions: Utilize Excel’s NPV function to simplify calculations and minimize errors.
Tip 8: Seek Professional Advice: Consider consulting financial professionals for guidance on complex projects or when dealing with high levels of uncertainty.
By following these tips, you can enhance the accuracy and reliability of your NPV calculations, leading to more informed investment decisions.
In the concluding section, we will explore real-world case studies to demonstrate the practical application of the discount rate formula for NPV in Excel and its significance in capital budgeting and investment analysis.
Conclusion
Throughout this article, we have explored the intricacies of the discount rate formula for Net Present Value (NPV) in Excel. This formula serves as a cornerstone of capital budgeting and investment analysis, enabling us to evaluate the profitability of long-term projects by considering the time value of money.
Key insights gained from our exploration include the importance of determining an appropriate discount rate that reflects the project’s risk profile and the opportunity cost of capital. We also highlighted the need to consider inflation and use consistent time periods for accurate NPV calculations. Furthermore, it is crucial to incorporate all relevant cash flows and perform sensitivity analysis to assess the impact of varying assumptions on the NPV.