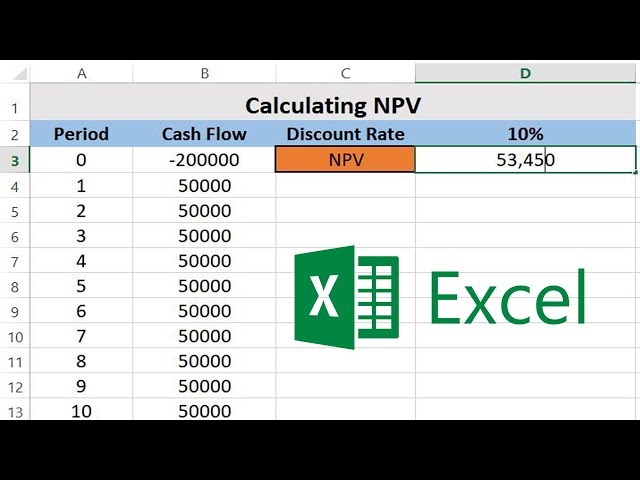
Net Present Value (NPV) is a financial metric used to determine the profitability of a project or investment. It takes into account the time value of money by discounting future cash flows back to the present at a given discount rate. Calculating NPV in Excel is a common task for financial analysts and investors, allowing them to make informed decisions about potential investments.
Understanding how to calculate NPV with discount rate in Excel is essential for financial analysis and decision-making. It helps individuals assess the viability of projects, compare different investment options, and optimize capital allocation. The concept of NPV was first introduced by Irving Fisher in his 1930 book “The Theory of Interest,” where he discussed the importance of considering the time value of money in investment decisions.
This article will provide a comprehensive guide on how to calculate NPV with discount rate in Excel, including step-by-step instructions, examples, and best practices. By the end of this article, readers will have a solid understanding of this fundamental financial calculation and its practical applications.
How to Calculate NPV with Discount Rate in Excel
Understanding the various dimensions of NPV calculation in Excel is crucial for accurate financial analysis and decision-making.
- Project Cash Flows
- Discount Rate
- Time Value of Money
- Excel Functions (NPV, IRR)
- Sensitivity Analysis
- Investment Analysis
- Capital Budgeting
- Project Evaluation
- Financial Modeling
- Investment Appraisal
These aspects are interconnected and play a vital role in NPV calculation. Project cash flows, discount rate, and time value of money are the foundational elements, while Excel functions simplify the calculation process. Sensitivity analysis helps assess the impact of changing variables, and NPV is widely used in investment analysis, capital budgeting, project evaluation, and financial modeling. Understanding these aspects enables financial professionals to make informed decisions and optimize investment outcomes.
Project Cash Flows
Project cash flows are the backbone of NPV calculations. They represent the inflows and outflows of cash associated with a project over its lifetime. Accurately estimating project cash flows is crucial for reliable NPV analysis.
- Initial Investment: The initial investment is the upfront cost of a project, which includes expenses such as equipment, setup costs, and working capital.
- Operating Cash Flows: Operating cash flows are the cash generated from the project’s ongoing operations, typically calculated using historical data or financial projections.
- Terminal Cash Flow: The terminal cash flow represents the project’s salvage value or proceeds from the sale of assets at the end of its useful life.
- Financing Cash Flows: Financing cash flows include any debt or equity financing received or repaid during the project’s lifetime.
Understanding the components of project cash flows allows for more precise NPV calculations. By carefully considering initial investments, operating cash flows, terminal cash flows, and financing cash flows, financial analysts can better assess the profitability and viability of a project.
Discount Rate
Discount rate plays a pivotal role in NPV calculation in Excel. It represents the rate at which future cash flows are discounted back to the present to determine the project’s net present value. The discount rate is a critical component of NPV calculation as it directly affects the present value of future cash flows. A higher discount rate will result in a lower NPV, while a lower discount rate will lead to a higher NPV.
The choice of discount rate is crucial and should be carefully considered based on the project’s risk profile, industry norms, and the cost of capital. In practice, discount rates are often determined using the weighted average cost of capital (WACC) or comparable market rates for similar projects. Accurately estimating the discount rate is essential for reliable NPV calculations.
By understanding the relationship between discount rate and NPV, financial analysts can make informed decisions about project viability and investment opportunities. NPV analysis helps prioritize projects, allocate capital effectively, and mitigate financial risks. It is a valuable tool for project evaluation, capital budgeting, and investment decision-making.
Time Value of Money
In the realm of financial analysis, the time value of money (TVM) is a fundamental principle that underpins the calculation of Net Present Value (NPV) in Excel. TVM recognizes that the value of money changes over time, affected by factors such as inflation, interest rates, and investment opportunities. Understanding TVM is essential for accurately assessing the profitability of projects and making sound investment decisions.
Within the context of NPV calculation, TVM plays a critical role. NPV is a metric used to determine the present value of future cash flows, discounted back to the current date using a specified discount rate. The discount rate, which is typically derived from the cost of capital or market interest rates, represents the time value of money and reflects the opportunity cost of investing in a project.
Real-life examples abound that demonstrate the impact of TVM on NPV calculations. Consider an investment that offers a future cash flow of $10,000 in five years. If the prevailing discount rate is 5%, the present value of this cash flow is approximately $7,835. This is because the $10,000 received in five years is worth less than $10,000 today due to the time value of money. A higher discount rate would further reduce the present value, reflecting the higher opportunity cost of capital.
Comprehending the relationship between TVM and NPV calculation is crucial for financial analysts, investors, and business professionals. It enables them to make informed decisions about project viability, capital allocation, and investment strategies. By incorporating TVM into NPV calculations, they can accurately assess the present value of future cash flows, mitigate financial risks, and optimize returns on investment.
Excel Functions (NPV, IRR)
When calculating Net Present Value (NPV) in Excel, two essential functions come into play: NPV and IRR. These functions are purpose-built to simplify and enhance the NPV calculation process, making it more accessible and efficient for financial analysts and investors.
The NPV function in Excel takes an array of cash flows and a discount rate as inputs and calculates the NPV of the project. The formula for NPV is: “`=NPV(discount_rate, cash_flow_range)“` where: `discount_rate` is the discount rate to be applied to the cash flows `cash_flow_range` is the range of cells containing the cash flows
On the other hand, the IRR function in Excel calculates the Internal Rate of Return (IRR) of a project, which is the discount rate that makes the NPV of the project equal to zero. The formula for IRR is:
=IRR(cash_flow_range)
where: * `cash_flow_range` is the range of cells containing the cash flows
Understanding the relationship between these Excel functions and the broader concept of NPV calculation is crucial for accurate financial analysis and decision-making. By leveraging these functions, financial professionals can efficiently assess the profitability and viability of projects, compare investment options, and make informed capital allocation decisions.
Sensitivity Analysis
Sensitivity analysis is a critical component of NPV calculation in Excel because it allows analysts to assess the impact of changes in input variables on the NPV. By varying the values of key assumptions, such as the discount rate, cash flows, and project duration, analysts can determine how sensitive the NPV is to these changes and identify potential risks and opportunities.
In practice, sensitivity analysis is often performed using a data table in Excel. The analyst enters different values for the input variables into the data table, and Excel automatically recalculates the NPV for each set of values. This allows the analyst to easily visualize how the NPV changes in response to different assumptions.
For example, an analyst might perform a sensitivity analysis on the discount rate to determine how changes in the cost of capital affect the NPV of a project. By varying the discount rate from, say, 5% to 10%, the analyst can see how the NPV changes. If the NPV is highly sensitive to changes in the discount rate, it indicates that the project is risky and may be vulnerable to changes in the market environment.
Sensitivity analysis is an essential tool for financial analysts and investors because it helps them to make more informed decisions about potential investments. By understanding how the NPV changes in response to different assumptions, analysts can better assess the risks and rewards of a project and make more informed capital allocation decisions.
Investment Analysis
Investment analysis is a fundamental aspect of calculating Net Present Value (NPV) in Excel, as it provides a systematic framework for evaluating the financial viability and potential return on investment of a project or investment opportunity.
- Project Evaluation: Investment analysis is used to assess the potential profitability and risks associated with a project. It involves evaluating factors such as project cash flows, initial investment costs, operating expenses, and potential returns.
- Capital Budgeting: Investment analysis plays a crucial role in capital budgeting decisions, where companies evaluate and select investment projects that align with their strategic objectives and maximize shareholder value.
- Risk Assessment: Investment analysis helps in identifying and quantifying potential risks associated with an investment. It involves analyzing factors such as market volatility, competition, regulatory changes, and operational risks.
- Scenario Planning: Investment analysis allows for scenario planning, where analysts can evaluate the impact of different assumptions and variables on the NPV of a project. This helps in making informed decisions and mitigating potential risks.
Overall, investment analysis provides a structured approach to evaluate and compare investment opportunities, considering factors such as profitability, risk, and alignment with strategic goals. It enables investors and financial analysts to make informed decisions about capital allocation and maximize the potential return on their investments.
Capital Budgeting
Capital Budgeting is a critical aspect of calculating Net Present Value (NPV) in Excel, as it provides a framework for evaluating and selecting long-term investment projects with the goal of maximizing shareholder value.
- Project Evaluation: Assessing the potential profitability, risks, and cash flows associated with a project to determine its financial viability and alignment with strategic objectives.
- Resource Allocation: Deciding how to allocate limited financial resources among competing investment opportunities, considering factors such as NPV, return on investment, and risk.
- Risk Management: Identifying and mitigating potential risks associated with capital projects, such as market fluctuations, technological changes, and regulatory shifts.
- Performance Monitoring: Tracking the progress of capital projects and comparing actual results to projections to ensure that they are meeting expectations and delivering the desired return on investment.
These facets of Capital Budgeting are interconnected and essential for making sound investment decisions. By considering these factors and calculating NPV using Excel, businesses can prioritize projects, allocate resources effectively, manage risks, and maximize the value they create for shareholders.
Project Evaluation
Project Evaluation lies at the heart of calculating Net Present Value (NPV) in Excel. It involves a comprehensive assessment of a project’s financial viability, risks, and potential returns. This evaluation is critical because it provides the basis for determining whether a project is worth pursuing and how it aligns with the organization’s strategic objectives.
NPV, calculated using Excel, plays a central role in Project Evaluation. It quantifies the present value of a project’s future cash flows, taking into account the time value of money and an appropriate discount rate. By comparing the NPV to the initial investment cost, organizations can determine if a project is likely to generate a positive return and contribute to shareholder value.
Real-life examples abound where Project Evaluation using NPV has guided critical investment decisions. Consider a company contemplating a new product launch. Through Project Evaluation, they would assess the potential sales revenue, production costs, marketing expenses, and other relevant cash flows associated with the launch. Using Excel to calculate the NPV, they would determine whether the project is financially feasible and aligns with their growth strategy.
Understanding the connection between Project Evaluation and NPV calculation is essential for making sound investment decisions. It allows organizations to prioritize projects, allocate resources effectively, and mitigate financial risks. By incorporating Project Evaluation into their NPV calculations, businesses can maximize their chances of success and drive long-term profitability.
Financial Modeling
Financial Modeling plays a pivotal role in the context of calculating Net Present Value (NPV) using Excel. It provides a structured framework for representing and analyzing the financial performance of a project or investment over time, considering various factors and assumptions.
Financial Modeling serves as the foundation for NPV calculation, as it enables the projection of future cash flows, estimation of initial investment costs, and incorporation of relevant financial assumptions. Without a robust financial model, calculating NPV accurately would be challenging, potentially leading to unreliable or misleading results.
Real-life examples showcase the practical applications of Financial Modeling in NPV calculation. Consider a company evaluating a new product launch. The financial model would incorporate assumptions about sales volume, production costs, marketing expenses, and other relevant factors. These assumptions would then drive the projection of future cash flows, which would be discounted back to the present using an appropriate discount rate to calculate the NPV.
Understanding the connection between Financial Modeling and NPV calculation is crucial for financial analysts, investors, and business professionals. It empowers them to make informed decisions about project viability, capital allocation, and investment strategies. By leveraging Financial Modeling, they can accurately assess the profitability and risks associated with potential investments, maximizing returns and mitigating financial risks.
Investment Appraisal
Investment Appraisal plays a crucial role in the context of calculating Net Present Value (NPV) using Excel. NPV is a critical metric used to evaluate the financial viability of a project or investment, and Investment Appraisal provides a comprehensive framework for assessing its profitability, risks, and potential returns. Without Investment Appraisal, calculating NPV would be challenging, as it requires a thorough understanding of the project’s financial aspects and implications.
Investment Appraisal involves analyzing various factors, such as the initial investment cost, projected cash flows, and the appropriate discount rate. These factors are incorporated into the NPV calculation, which determines whether the project is financially feasible and aligns with the organization’s strategic objectives. By considering these factors, Investment Appraisal helps to mitigate financial risks and make informed investment decisions.
In real-life scenarios, Investment Appraisal using NPV has guided critical investment decisions across industries. For instance, a company evaluating a new product launch would use Investment Appraisal to assess the potential sales revenue, production costs, marketing expenses, and other relevant cash flows. Using Excel to calculate the NPV, they would determine whether the project is likely to generate a positive return and contribute to shareholder value.
In conclusion, understanding the connection between Investment Appraisal and NPV calculation is essential for financial professionals, investors, and business decision-makers. It empowers them to make informed decisions about project viability, capital allocation, and investment strategies. By incorporating Investment Appraisal into their NPV calculations, they can accurately assess the profitability and risks associated with potential investments, maximizing returns and mitigating financial risks.
Frequently Asked Questions (FAQs) on Calculating NPV with Discount Rate in Excel
This FAQ section aims to provide concise answers to common queries or areas of confusion related to calculating Net Present Value (NPV) with discount rate in Excel.
Question 1: What is the purpose of calculating NPV?
Answer: NPV is used to determine the present value of a project’s future cash flows, considering the time value of money and an appropriate discount rate. It helps assess the profitability and viability of an investment or project.
Question 2: How do I choose the appropriate discount rate?
Answer: The discount rate should reflect the cost of capital or the required rate of return for the project. It can be based on the weighted average cost of capital (WACC) or comparable market rates.
Question 3: What if the NPV is negative?
Answer: A negative NPV indicates that the project’s present value of future cash flows is less than the initial investment. This suggests that the project may not be financially viable or may not meet the desired rate of return.
Question 4: How does the discount rate affect NPV?
Answer: A higher discount rate will result in a lower NPV, while a lower discount rate will lead to a higher NPV. This is because the discount rate represents the opportunity cost of capital.
Question 5: Can I use Excel to calculate NPV?
Answer: Yes, Excel has a built-in NPV function that allows you to easily calculate the NPV of a project. You can use the formula “=NPV(discount_rate, cash_flow_range)” to calculate the NPV.
Question 6: What are some best practices for calculating NPV?
Answer: Best practices include using accurate cash flow projections, considering inflation, and performing sensitivity analysis to assess the impact of changes in assumptions.
These FAQs provide a foundation for understanding the key aspects of calculating NPV with discount rate in Excel. In the next section, we will explore advanced techniques and applications of NPV analysis for investment decision-making.
Tips for Calculating NPV with Discount Rate in Excel
To enhance your NPV calculations, consider these valuable tips:
Tip 1: Use Accurate Cash Flow ProjectionsAccurate cash flow projections are crucial for reliable NPV calculations. Consider historical data, industry benchmarks, and potential risks.
Tip 2: Consider InflationInflation can impact future cash flows. Adjust cash flows for inflation to ensure a realistic NPV calculation.
Tip 3: Perform Sensitivity AnalysisSensitivity analysis helps assess the impact of changing assumptions on NPV. Vary discount rates, cash flows, and project duration to evaluate potential risks and opportunities.
Tip 4: Use Excel FunctionsExcel provides NPV and IRR functions to simplify and enhance NPV calculations. Utilize these functions to automate calculations and improve accuracy.
Tip 5: Consider Multiple Discount RatesUsing a single discount rate may not capture the full range of potential outcomes. Consider using multiple discount rates to assess the robustness of your NPV calculations.
Tip 6: Incorporate Risk PremiumsRisk premiums can be added to the discount rate to account for project-specific risks. This adjustment helps ensure that the NPV reflects the potential risks involved.
Tip 7: Use Scenario AnalysisScenario analysis allows you to evaluate different sets of assumptions and their impact on NPV. This technique provides a comprehensive understanding of potential outcomes.
Tip 8: Seek Professional AdviceFor complex or high-value projects, consider seeking advice from financial professionals. They can provide guidance on appropriate discount rates, cash flow projections, and risk assessment.
These tips empower you to perform robust and accurate NPV calculations, leading to more informed investment decisions. In the final section, we will explore advanced applications of NPV analysis for capital budgeting and investment appraisal.
Conclusion
This comprehensive guide has delved into the intricacies of calculating Net Present Value (NPV) with discount rate in Excel. We explored the fundamental concepts, including project cash flows, discount rate, time value of money, and Excel functions. By understanding these building blocks, financial analysts and investors can accurately assess the profitability and viability of projects.
Key takeaways include the importance of using accurate cash flow projections, considering inflation, and performing sensitivity analysis to mitigate risks and enhance decision-making. Additionally, leveraging Excel functions and incorporating risk premiums can further refine NPV calculations. These techniques empower professionals to make informed investment decisions, prioritize projects, and allocate capital effectively.