Calculating Present Value with Discount Rate in Excel: A Comprehensive Guide for Financial Analysis
Calculating present value with a discount rate in Microsoft Excel is a fundamental financial modeling technique used to determine the current worth of future cash flows. For instance, if you anticipate receiving $1,000 in two years, its present value at a 5% discount rate would be $907.18. Understanding this concept is vital for investment decisions, loan analysis, and financial planning.
The importance of present value calculations lies in their ability to compare cash flows occurring at different points in time. By discounting future cash flows back to their present value, investors can make informed decisions about the attractiveness of investment opportunities. The development of electronic spreadsheets, such as Excel, has revolutionized the process of present value calculations, making it more accessible and efficient.
This article will provide a comprehensive guide to calculating present value with a discount rate in Microsoft Excel, covering the formula, step-by-step instructions, and practical examples. By understanding these techniques, you can leverage Excel’s capabilities for accurate and efficient financial analysis.
How to Calculate Present Value with Discount Rate in Excel
Understanding the key aspects of calculating present value with discount rate in Excel is essential for accurate financial analysis and decision-making.
- Formula
- Discount Rate
- Cash Flows
- Time Value of Money
- NPV Function
- IRR Function
- PV Function
- PMT Function
- RATE Function
- Annuity
These aspects encompass the core concepts, functions, and calculations involved in present value analysis. By understanding the formula, discount rate, and time value of money, individuals can accurately determine the present value of future cash flows and make informed financial decisions. Excel’s built-in functions, such as NPV, IRR, PV, PMT, and RATE, provide efficient tools for performing these calculations.
Formula
The formula used to calculate present value with discount rate in Excel is the cornerstone of financial analysis, providing the mathematical foundation for determining the current worth of future cash flows. Understanding its components and implications is crucial for accurate financial modeling and decision-making.
- Present Value (PV)
The present value is the discounted value of a future cash flow, representing its current worth considering the time value of money and the discount rate.
- Discount Rate (r)
The discount rate is the rate used to discount future cash flows back to their present value, reflecting the cost of capital or the required rate of return.
- Cash Flows (CF)
Cash flows represent the inflows or outflows of money over time, which are discounted to determine their present value.
- Time (t)
The time variable captures the duration between the present and the period when the cash flow occurs, influencing the present value calculation.
These elements work together in the present value formula: PV = CF / (1 + r)^t. By understanding the interplay of these components, individuals can accurately calculate present values and make informed financial decisions. The formula provides a framework for analyzing investments, loans, and other financial scenarios, enabling individuals to assess the time value of money and make sound financial choices.
Discount Rate
In the realm of present value calculations with discount rate in Excel, the discount rate holds paramount importance. It serves as the linchpin, influencing the present value of future cash flows and shaping financial decision-making. To grasp the intricacies of present value calculations, delving into the multifaceted nature of the discount rate is essential.
- Cost of Capital
The discount rate often reflects the cost of capital for a project or investment, representing the minimum return required by investors or lenders.
- Risk-Free Rate
The risk-free rate, such as government bond yields, serves as a benchmark against which the discount rate is often compared.
- Inflation
Inflation erodes the value of money over time, and the discount rate should consider inflationary expectations to accurately reflect future cash flows.
- Project Risk
The discount rate should incorporate a risk premium to account for the uncertainty and potential volatility associated with a particular project or investment.
Understanding these facets of the discount rate empowers individuals to make informed decisions when calculating present values in Excel. By carefully considering the cost of capital, risk-free rate, inflation, and project risk, they can derive accurate present values that serve as the foundation for sound financial analysis and decision-making.
Cash Flows
Cash flows are the lifeblood of present value calculations in Excel. They represent the inflows and outflows of money over time, which are subsequently discounted to determine their present value. Understanding the nuances of cash flows is essential for accurate financial modeling and decision-making.
- Operating Cash Flows
Operating cash flows encompass the cash generated from a company’s core operations, including revenue, expenses, and working capital changes.
- Investing Cash Flows
Investing cash flows reflect the cash used in acquiring or disposing of long-term assets, such as property, plant, and equipment.
- Financing Cash Flows
Financing cash flows represent the cash used to raise capital or repay debt, including issuing stocks, bonds, or taking out loans.
- Free Cash Flows
Free cash flows measure the cash available to a company after accounting for operating, investing, and financing activities.
These cash flow classifications provide a comprehensive view of a company’s financial health and performance. By analyzing cash flows in conjunction with the discount rate, individuals can make informed decisions about investments, loans, and other financial scenarios, ensuring that the time value of money is appropriately considered.
Time Value of Money
In calculating present value with discount rate in Excel, the concept of time value of money (TVM) plays a pivotal role. TVM recognizes that the value of money changes over time, influencing the present value of future cash flows. Understanding TVM’s multifaceted nature is crucial for accurate financial modeling and decision-making.
- Present vs. Future Value
TVM acknowledges that a dollar today is worth more than a dollar in the future due to the potential for investment and earning interest.
- Discounting Future Cash Flows
To determine the present value of future cash flows, they must be discounted using an appropriate discount rate, which reflects the cost of capital or required rate of return.
- Impact on Investment Decisions
TVM helps investors evaluate the present value of future returns from investments, enabling them to make informed choices about allocating their capital.
- Loan and Mortgage Calculations
TVM is essential for calculating the present value of loan payments, determining the total cost of borrowing, and comparing different loan options.
Understanding and incorporating TVM into present value calculations in Excel empowers individuals to make sound financial decisions. It provides a framework for evaluating the time-dependent value of money, ensuring that the present worth of future cash flows is accurately assessed.
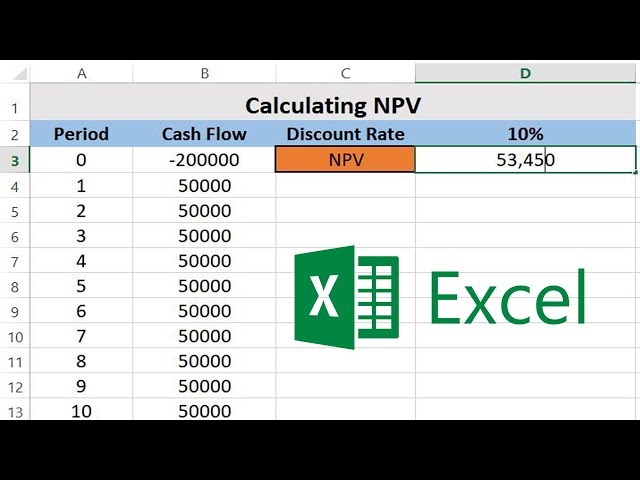
NPV Function
The NPV function in Excel is a critical component of calculating present value with discount rate, serving as a powerful tool for evaluating the financial viability of investments and projects. Its significance stems from its ability to incorporate the time value of money and discount future cash flows back to their present value, allowing for accurate assessment of an investment’s profitability.
The NPV function takes two primary arguments: an array of cash flows and a discount rate. By inputting the cash flows and the appropriate discount rate, the NPV function calculates the present value of the investment. A positive NPV indicates that the investment is expected to generate a positive return, while a negative NPV suggests that the investment may not be financially viable.
In practical applications, the NPV function plays a crucial role in capital budgeting and investment analysis. It enables businesses and individuals to compare different investment opportunities, assess the potential profitability of projects, and make informed decisions about allocating their capital. By incorporating the NPV function into present value calculations, users can gain a comprehensive understanding of the time-dependent value of money and make sound financial choices.
IRR Function
The IRR (Internal Rate of Return) function in Microsoft Excel is inextricably linked to the calculation of present value with discount rate. It plays a pivotal role in assessing the profitability and financial viability of investments and projects, making it a critical component of present value analysis in Excel.
The IRR function calculates the discount rate at which the net present value (NPV) of a series of cash flows equals zero. This discount rate represents the internal rate of return on the investment. A positive IRR indicates that the investment is expected to generate a positive return, while a negative IRR suggests that the investment may not be financially viable.
In practical applications, the IRR function is widely used in capital budgeting and investment analysis. It enables businesses and individuals to compare different investment opportunities, assess the potential profitability of projects, and make informed decisions about allocating their capital. By understanding the relationship between the IRR function and present value calculations, users can gain a comprehensive understanding of the time-dependent value of money and make sound financial choices.
The IRR function is often used in conjunction with the NPV function to evaluate investments. The NPV function calculates the present value of an investment at a specified discount rate, while the IRR function calculates the discount rate that results in a zero NPV. This combination of functions provides a powerful tool for evaluating the financial viability of investments and making informed capital budgeting decisions.
PV Function
The PV function in Microsoft Excel is intricately intertwined with the calculation of present value using a discount rate, forming a cornerstone of financial modeling and analysis. Its significance lies in its ability to determine the present value of a future sum of money, taking into account the time value of money and a specified discount rate. Without the PV function, calculating present value would be a complex and error-prone task, making it a critical component of this financial calculation.
Real-life applications of the PV function abound in various financial scenarios. For instance, in investment analysis, it is used to assess the present value of future cash flows generated by an investment, enabling investors to make informed decisions about potential returns. Similarly, in loan analysis, the PV function plays a vital role in calculating the present value of future loan payments, helping borrowers understand the true cost of borrowing.
Understanding the connection between the PV function and present value calculations is essential for anyone involved in financial planning, investment analysis, or loan assessment. By harnessing the capabilities of the PV function in Excel, individuals can accurately determine the present value of future cash flows, make informed financial decisions, and optimize their financial strategies.
PMT Function
In calculating present value (PV) with discount rate using Microsoft Excel, the PMT function plays a crucial role. It calculates the periodic payment amount, such as monthly mortgage payments or annual loan installments, required to pay off a loan or investment over a specified period, taking into account the loan amount, interest rate, and loan term.
- Loan Repayment Schedule
The PMT function is instrumental in creating loan repayment schedules, providing a detailed breakdown of each payment’s principal and interest components, assisting borrowers in understanding the amortization of their loans.
- Investment Analysis
In investment analysis, the PMT function helps assess the periodic payments required to achieve a specific investment goal, such as saving for retirement or a down payment on a house, considering factors like investment horizon, interest rates, and risk tolerance.
- Real-Life Applications
Beyond financial calculations, the PMT function finds practical applications in various fields. For instance, in project management, it can be used to calculate regular payments for equipment leasing or software subscriptions.
These facets highlight the versatility and significance of the PMT function in the context of calculating present value with discount rate in Excel. Understanding its components and applications empowers individuals to make informed financial decisions, plan for the future, and optimize their financial strategies.
RATE Function
The RATE function in Microsoft Excel is an integral part of calculating present value with discount rate, providing a versatile tool for financial analysis and decision-making. Its significance lies in determining the interest rate or yield of an investment or loan, considering the present value, future value, and number of periods.
- Calculating Interest Rates
The RATE function is commonly used to calculate the interest rate or yield of an investment or loan, given its present value, future value, and the number of periods. This is particularly useful for comparing different investment options or assessing the cost of borrowing.
- Annuity Payments
In the context of annuities, the RATE function helps determine the periodic interest rate or yield, considering the present value of the annuity, the number of payments, and the future value.
- Loan Analysis
For loan analysis, the RATE function can be used to calculate the annual percentage rate (APR) or effective interest rate of a loan, providing insights into the true cost of borrowing.
- Investment Planning
In investment planning, the RATE function can be leveraged to determine the required rate of return or yield to achieve a specific investment goal, considering the present value, future value, and investment horizon.
These facets collectively demonstrate the versatility and significance of the RATE function in calculating present value with discount rate in Excel. By understanding its components and applications, individuals can make informed financial decisions, optimize investment strategies, and assess the true cost of borrowing, empowering them to navigate financial scenarios with confidence.
Annuity
An annuity is a series of equal payments made at regular intervals, often annually or monthly. Annuities play a prominent role in calculating present value with discount rate in Excel, as they represent a common financial instrument used in various scenarios, such as retirement planning, loan payments, and investment analysis.
The significance of annuities in present value calculations lies in their predictable cash flow pattern. By knowing the amount and timing of future payments, individuals can accurately determine their present value using the appropriate discount rate. The present value of an annuity is the current worth of all future payments, discounted back to the present using the specified discount rate. This calculation is essential for evaluating the overall value and financial viability of annuity-based investments or financial commitments.
Real-life examples of annuities abound in personal finance and investment. For instance, a monthly pension payment received during retirement is an annuity. Similarly, regular loan payments made to pay off a mortgage or car loan represent an annuity. In each case, the present value calculation helps individuals understand the current worth of these future cash flows, considering the time value of money and the applicable discount rate.
Understanding the connection between annuities and present value calculations in Excel empowers individuals to make informed financial decisions. By accurately determining the present value of annuities, they can effectively compare different investment options, assess the affordability of loans, and plan for future financial goals. This understanding forms the foundation for sound financial planning and prudent investment strategies.
Frequently Asked Questions
This section addresses common questions and clarifies aspects related to calculating present value with discount rate in Excel.
Question 1: What is the formula for calculating present value with discount rate in Excel?
Answer: The formula is PV = FV / (1 + r)^n, where PV is the present value, FV is the future value, r is the discount rate, and n is the number of periods.
Question 2: How do I choose the appropriate discount rate for my calculations?
Answer: The discount rate should reflect the cost of capital or required rate of return, considering factors such as inflation, project risk, and alternative investment opportunities.
Question 3: Can I use Excel functions to simplify present value calculations?
Answer: Yes, Excel provides functions like NPV, IRR, PV, PMT, and RATE, which can automate calculations and enhance accuracy.
Question 4: How do I handle irregular cash flows in present value calculations?
Answer: For irregular cash flows, you can create a timeline and discount each cash flow individually, or use interpolation methods to estimate cash flows for intermediate periods.
Question 5: What are some real-world applications of present value calculations?
Answer: Present value calculations are used in investment analysis, loan evaluation, project appraisal, and financial planning, among others.
Question 6: How can present value calculations help me make informed financial decisions?
Answer: By determining the present worth of future cash flows, present value calculations allow for comparison of investment alternatives, assessment of loan affordability, and planning for financial goals.
These FAQs provide essential insights into the concepts and practical applications of present value calculations with discount rate in Excel, empowering individuals to make informed financial decisions and effectively manage their financial resources.
In the next section, we will delve deeper into the practical implementation of present value calculations in Excel, providing step-by-step instructions and examples to further enhance your understanding.
Tips for Calculating Present Value with Discount Rate in Excel
To enhance your understanding of present value calculations in Excel, consider these practical tips that provide actionable guidance and insights.
Tip 1: Choose an Appropriate Discount Rate
Determine the discount rate that accurately reflects the cost of capital or required rate of return for your specific investment or project.
Tip 2: Consider the Time Value of Money
Recognize that the value of money changes over time, and discount future cash flows to determine their present worth.
Tip 3: Check for Consistent Units
Ensure that the discount rate and cash flows are expressed in the same units (e.g., annual, monthly) to avoid errors.
Tip 4: Utilize Excel Functions
Leverage Excel’s built-in functions like NPV, IRR, and PV to automate calculations and enhance accuracy.
Tip 5: Handle Irregular Cash Flows
For cash flows that occur at irregular intervals, create a timeline or use interpolation methods to estimate cash flows for intermediate periods.
Tip 6: Consider Inflation
In long-term calculations, account for inflation by adjusting the discount rate or cash flows to reflect the impact of rising prices.
Tip 7: Sensitivity Analysis
Perform sensitivity analysis by varying the discount rate or cash flows to assess the impact on the present value and identify potential risks.
Tip 8: Seek Professional Advice
For complex or high-value calculations, consider consulting with a financial professional to ensure accuracy and reliability.
By incorporating these tips into your present value calculations, you can enhance the accuracy and reliability of your financial analysis, and make informed decisions based on a comprehensive understanding of the time value of money.
In the concluding section, we will discuss best practices for using present value calculations to evaluate investment opportunities and make sound financial choices.
Conclusion
Throughout this comprehensive guide, we have explored the intricacies of calculating present value with discount rate in Excel, uncovering its significance in financial analysis and decision-making. We have delved into the core concepts, formula, and practical applications of present value calculations, equipping you with the knowledge and skills to accurately assess the time value of money and make informed financial choices.
Remember, the key to successful present value calculations lies in choosing an appropriate discount rate, considering the time value of money, and utilizing Excel functions to enhance accuracy. By incorporating these principles into your financial analysis, you can effectively evaluate investment opportunities, assess loan affordability, and plan for your financial future with confidence.