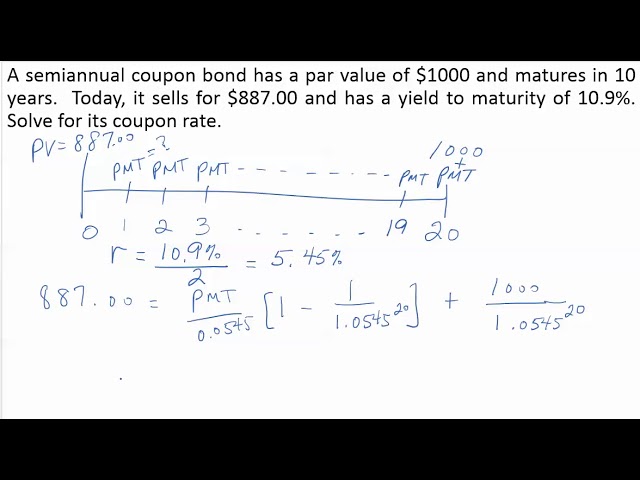
The process of calculating the coupon payment, an important aspect of bond valuation and investment analysis.
This calculation helps investors determine the recurring interest payments they will receive, providing a reliable stream of income and enhancing the value of their investment.
Coupon payment calculations have evolved since the early days of bonds, when they were calculated manually using complex formulas and tables. Today, technology and financial calculators make this process more accessible and efficient.
How to Calculate the Coupon Payment of a Bond
Coupon payment calculations involve several key aspects, each playing a crucial role in determining the regular interest payments received by bondholders.
- Face Value
- Coupon Rate
- Payment Frequency
- Maturity Date
- Accrued Interest
- Bond Price
- Yield to Maturity
- Present Value
- Future Value
- Tax Implications
Understanding these aspects enables investors to assess the potential income and risks associated with bond investments. By considering factors such as the bond’s face value, coupon rate, and payment frequency, investors can make informed decisions about which bonds align with their financial goals.
Face Value
In the realm of bond calculations, the face value, also known as par value, holds significant importance. It serves as the principal amount, the foundation upon which coupon payments are determined.
- Fixed Amount: The face value remains constant throughout the bond’s life, providing a clear reference point for calculating periodic coupon payments.
- Bond Issuance: At the time of bond issuance, the face value represents the amount borrowed by the issuer from investors.
- Maturity Payment: Upon maturity, bondholders receive the face value, marking the repayment of the principal amount.
- Bond Quotation: The face value is often used as the basis for bond quotations, providing a standardized reference for comparing bonds with different values.
These facets of face value collectively contribute to its pivotal role in bond calculations, enabling investors to assess the potential returns and make informed investment decisions.
Coupon Rate
Within the context of bond calculations, the coupon rate plays a central role in determining the periodic interest payments received by bondholders. It represents the annual rate of interest expressed as a percentage of the bond’s face value.
- Fixed Rate: Bonds typically carry a fixed coupon rate, providing consistent interest payments throughout the bond’s life.
- Variable Rate: Some bonds have floating or variable coupon rates, which fluctuate based on market conditions or a specified index.
- Zero Coupon: Zero-coupon bonds do not pay periodic interest payments, but are issued at a deep discount to their face value, with the difference representing the return to investors.
- Step-Up/Step-Down: Some bonds feature step-up or step-down coupon rates, where the rate changes at specified intervals during the bond’s life.
Understanding the nuances of coupon rates is crucial for investors to assess the potential income stream and risk associated with bond investments. These rates directly influence the coupon payment calculations, ultimately affecting the total return on investment.
Payment Frequency
Payment frequency plays a crucial role in determining the coupon payment of a bond. It refers to the number of times per year that the bondholder receives interest payments. The most common payment frequencies are semi-annual (twice a year) and annual (once a year), but bonds can also have monthly, quarterly, or even daily payment frequencies.
To calculate the coupon payment, the annual coupon rate is divided by the payment frequency. For example, a bond with a 5% annual coupon rate and semi-annual payments would have a coupon payment of 2.5%. This is because the bondholder receives half of the annual interest payment every six months.
Payment frequency has several practical implications. First, it affects the total number of coupon payments that the bondholder will receive over the life of the bond. Second, it influences the timing of the interest payments, which can be important for investors who need to match their cash flow needs with their investment income. Finally, payment frequency can impact the bond’s price and yield. Bonds with more frequent payment frequencies tend to have higher prices and lower yields than bonds with less frequent payment frequencies.
Maturity Date
In the realm of bond calculations, the maturity date holds significant importance for determining the coupon payment schedule and overall return on investment. It marks the specific date on which the bond matures, signifying the end of its lifespan and the repayment of the principal amount to the bondholder.
- Bond Expiration: Maturity date represents the expiration or termination of the bond contract, after which the bond ceases to exist as a financial instrument.
- Principal Repayment: On the maturity date, the bond issuer is obligated to repay the face value or principal amount of the bond to the holder.
- Interest Payment Cessation: Upon maturity, the bond stops paying periodic interest payments, as the principal has been fully repaid.
- Investment Horizon: The maturity date defines the investment horizon for bondholders, indicating the duration until they receive their principal back.
Understanding the implications of the maturity date is crucial for investors to assess the potential risks and rewards associated with bond investments. It influences factors such as investment duration, interest income predictability, and the potential for capital appreciation or loss.
Accrued Interest
Accrued interest is an important aspect to consider when calculating the coupon payment of a bond. It represents the interest that has accumulated on a bond since the last coupon payment date but has not yet been paid to the bondholder.
- Calculation: Accrued interest is calculated by multiplying the bond’s annual coupon rate by the number of days since the last coupon payment date, divided by the number of days in the coupon payment period.
- Example: A bond with a 5% annual coupon rate that pays interest semi-annually has accrued $25 of interest 90 days after the last coupon payment date, assuming a 180-day coupon payment period.
- Payment: Accrued interest is typically paid to the bondholder at the next coupon payment date, along with the regular coupon payment.
- Bond Price: Accrued interest is added to the bond’s purchase price to determine the bond’s total cost, which affects the bond’s yield to maturity calculation.
Understanding accrued interest is crucial for accurate coupon payment calculations and for assessing the bond’s overall value. It ensures that bondholders receive the correct amount of interest, taking into account the time that has elapsed since the last payment date.
Bond Price
Bond price plays a crucial role in determining the coupon payment of a bond. The coupon payment is calculated as a fixed percentage of the bond’s face value, which is typically equal to the bond’s issue price. As a result, changes in bond price directly affect the coupon payment. When bond prices rise, the coupon payment increases proportionally. Conversely, when bond prices fall, the coupon payment decreases.
In real-life scenarios, bond prices fluctuate based on various factors such as interest rate changes, inflation, and economic conditions. When interest rates rise, bond prices tend to fall, leading to lower coupon payments. Conversely, when interest rates fall, bond prices tend to rise, leading to higher coupon payments. Understanding this relationship is essential for investors to assess the potential impact of bond price changes on their coupon payments and overall investment returns.
Practically, calculating the coupon payment with respect to bond price involves determining the bond’s yield to maturity (YTM). YTM represents the annualized rate of return an investor can expect to receive by holding the bond until its maturity date. The YTM is calculated using the bond’s price, face value, coupon rate, and time to maturity. By knowing the YTM and the bond’s face value, investors can calculate the annual coupon payment as the product of the two.
Yield to Maturity
Yield to maturity (YTM) is a crucial concept in bond calculations, closely tied to the determination of the coupon payment. It represents the annualized rate of return an investor can expect to receive if they hold the bond until its maturity date and reinvest the coupon payments at the same rate.
- Components of YTM: YTM comprises several components, including the bond’s price, face value, coupon rate, and time to maturity.
- Bond Pricing: YTM is inversely related to bond prices. When YTM increases, bond prices decrease, and vice versa. This relationship influences the coupon payment calculation.
- Investment Strategy: YTM is a key consideration for investors planning to hold bonds until maturity. It helps them assess the potential return on their investment.
- Bond Comparison: YTM enables investors to compare different bonds and make informed decisions based on their risk tolerance and return expectations.
Understanding YTM is essential for calculating the coupon payment accurately and making informed investment decisions. It provides valuable insights into the bond’s value, return potential, and risk profile, helping investors navigate the bond market effectively.
Present Value
In the realm of bond calculations, present value plays a crucial role in determining the coupon payment. Present value represents the current worth of a future sum of money, discounted at a specific rate of return. In the context of bonds, it is used to calculate the value of the bond’s future coupon payments and the repayment of the principal at maturity.
The calculation of the coupon payment involves determining the present value of each future coupon payment and the present value of the principal repayment at maturity. The sum of these present values gives the bond’s current market value. The discount rate used in this calculation is typically the bond’s yield to maturity (YTM), which reflects the market’s required rate of return for the bond.
By understanding the relationship between present value and coupon payment calculations, investors can assess the fair value of bonds and make informed investment decisions. Present value analysis allows investors to compare bonds with different maturities, coupon rates, and credit risks, enabling them to construct a diversified bond portfolio that meets their investment goals and risk tolerance.
Future Value
In the context of calculating the coupon payment of a bond, Future Value plays a crucial role in determining the present value of future cash flows. It represents the value of a sum of money at a specified future date, taking into account the effects of compounding interest. Understanding Future Value is essential for accurately calculating coupon payments and assessing the overall value of a bond investment.
- Maturity Value: The future value of the bond’s face value at maturity, representing the principal repayment.
- Coupon Payments: The future value of each future coupon payment, discounted back to the present.
- Time Value of Money: The concept that money has different values at different points in time due to the potential for earning interest.
- Discount Rate: The rate used to discount future cash flows back to their present value, typically the bond’s yield to maturity.
By incorporating Future Value into coupon payment calculations, investors can assess the time value of money and make informed decisions about the attractiveness of bond investments. It provides a comprehensive view of the bond’s future cash flows, enabling investors to evaluate the potential return on their investment and manage their investment portfolios effectively.
Tax Implications
Tax implications play a significant role in calculating the coupon payment of a bond, affecting the net return received by investors. These implications vary depending on factors such as the investor’s tax bracket, the type of bond, and the tax laws of the jurisdiction in which the bond is issued.
- Taxable Interest: Coupon payments on most bonds are subject to income tax, which reduces the after-tax return for investors.
- Tax-Free Interest: Municipal bonds issued by state and local governments are typically exempt from federal income tax, making them attractive to investors seeking tax-advantaged income.
- Capital Gains: When a bond is sold for a profit, the capital gains realized are subject to capital gains tax, which can further impact the overall return on investment.
- Tax-Deferred Growth: Bonds held in tax-advantaged accounts such as IRAs and 401(k) plans allow for tax-deferred growth, where interest earnings are not taxed until withdrawal.
Understanding the tax implications associated with bond investments is crucial for investors to accurately calculate their after-tax returns and make informed investment decisions. By considering the potential tax liabilities and benefits, investors can optimize their bond portfolios to minimize taxes and maximize their overall investment returns.
Frequently Asked Questions
This FAQ section addresses common queries and misconceptions related to calculating the coupon payment of a bond.
Question 1: What is the formula for calculating the coupon payment?
The coupon payment is calculated by multiplying the bond’s annual coupon rate by its face value and dividing by the number of payment periods per year.
Question 2: How does the payment frequency affect the coupon payment?
The payment frequency determines the number of times per year that the bondholder receives interest payments. More frequent payments result in smaller coupon payments, while less frequent payments result in larger coupon payments.
Question 3: What is the relationship between the bond price and the coupon payment?
The bond price and the coupon payment are inversely related. When bond prices rise, coupon payments decrease, and vice versa. This is because the total return on a bond is composed of both the coupon payments and the capital gain or loss when the bond is sold.
Question 4: How are taxes applied to coupon payments?
Coupon payments are generally subject to income tax, which can reduce the after-tax return on a bond investment. However, municipal bonds may offer tax-free interest payments.
Question 5: What is the difference between the coupon rate and the yield to maturity?
The coupon rate is the fixed interest rate specified on the bond, while the yield to maturity is the annualized rate of return an investor can expect to receive if they hold the bond until maturity.
Question 6: How can I calculate the present value of a bond’s coupon payments?
To calculate the present value of a bond’s coupon payments, you can use the formula: PV = C * [1 – (1 + r)^-n] / r, where C is the coupon payment, r is the yield to maturity, and n is the number of periods until maturity.
These FAQs provide a concise overview of the key aspects involved in calculating the coupon payment of a bond. Understanding these concepts is essential for making informed investment decisions and evaluating the potential risks and returns associated with bond investments.
In the next section, we will explore other important factors to consider when analyzing bonds, including credit risk and interest rate risk.
Tips for Calculating Bond Coupon Payments
This section provides practical and actionable tips to assist you in accurately calculating bond coupon payments. By following these tips, you can enhance your understanding of bond investments and make informed financial decisions.
Tip 1: Identify the Bond’s Face Value: Determine the principal amount of the bond, which serves as the basis for coupon payment calculations.
Tip 2: Determine the Annual Coupon Rate: Establish the fixed percentage of the face value that represents the annual interest payment.
Tip 3: Consider the Payment Frequency: Ascertain the number of times per year that coupon payments are made, as this affects the calculation of the individual payments.
Tip 4: Calculate Accrued Interest: Account for any interest accumulated since the last coupon payment date.
Tip 5: Understand the Impact of Bond Price: Recognize the inverse relationship between bond price and coupon payments, as price fluctuations influence the calculation.
Tip 6: Calculate Yield to Maturity (YTM): Determine the annualized rate of return expected if the bond is held until maturity, which is used in coupon payment calculations.
Tip 7: Consider Tax Implications: Be aware of the potential tax liabilities associated with coupon payments, as they can impact your net returns.
Tip 8: Seek Professional Advice: When in doubt, consult with a financial advisor or bond specialist to ensure accurate calculations and investment decisions.
These tips provide a comprehensive approach to calculating bond coupon payments, empowering you to make informed investment choices. Understanding these concepts is essential for navigating the bond market effectively.
In the final section, we will delve into additional strategies for analyzing bond investments, including risk assessment and portfolio diversification.
Conclusion
This comprehensive guide has explored the intricacies of calculating bond coupon payments, providing a solid foundation for understanding bond investments. We have highlighted the importance of factors such as face value, coupon rate, payment frequency, and yield to maturity in determining the periodic interest payments received by bondholders.
Understanding how to calculate coupon payments is crucial for investors seeking a reliable stream of income and evaluating the overall value of bond investments. By considering the interconnections between these factors, investors can make informed decisions about which bonds align with their financial goals and risk tolerance.