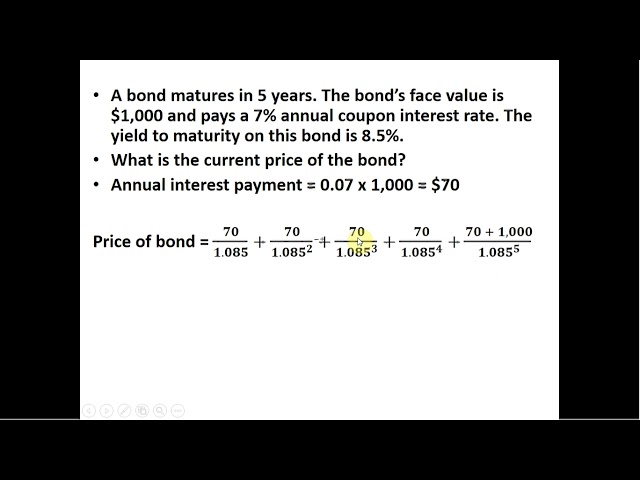
Determining “how to calculate the long coupon” entails understanding the methodology used to compute the interest payment made on a long-term bond. For instance, if a bond with a face value of $1,000 and a 10% coupon rate has a term of 20 years, its annual coupon payment would be $100 ($1,000 x 10%).
Calculating the long coupon is crucial for investors as it provides a basis for comparing bond yields, assessing potential returns, and making informed investment decisions. Historically, the development of fixed-income securities has significantly contributed to the growth and stability of financial markets.
This article will delve into the intricacies of calculating the long coupon, exploring its importance, benefits, and the historical context that shaped its significance in the financial landscape.
How to Calculate the Long Coupon
Understanding the essential aspects of calculating the long coupon is crucial for informed investment decisions. These aspects encompass:
- Coupon Rate
- Face Value
- Maturity Date
- Payment Frequency
- Accrued Interest
- Yield to Maturity
- Present Value
- Bond Pricing
- Coupon Payment Calculation
- Long Coupon versus Short Coupon
These aspects are interconnected, influencing the calculation and interpretation of the long coupon. Understanding their interplay provides a comprehensive view of bond valuation and investment strategies. For instance, the coupon rate and maturity date determine the bond’s yield to maturity, which is a key factor in assessing its attractiveness to investors.
Coupon Rate
In the context of calculating the long coupon, the coupon rate is pivotal, influencing the bond’s value and returns. It represents the annual interest payment made on a bond, expressed as a percentage of its face value.
- Fixed versus Floating
Coupon rates can be fixed, remaining constant throughout the bond’s life, or floating, adjusting periodically based on a benchmark rate.
- Impact on Bond Price
Higher coupon rates generally lead to higher bond prices, as investors are willing to pay a premium for bonds with more attractive interest payments.
- Credit Risk
Bonds with higher coupon rates may also indicate higher credit risk, as issuers with lower creditworthiness may offer higher interest rates to attract investors.
- Yield to Maturity
The coupon rate is a key factor in determining a bond’s yield to maturity, which represents the annualized return an investor can expect if they hold the bond until its maturity date.
Understanding the nuances of coupon rates is essential for investors to make informed decisions when valuing and selecting bonds. By considering the interplay between coupon rates and other factors such as maturity date and credit risk, investors can optimize their bond portfolios for their specific investment goals and risk tolerance.
Face Value
When calculating the long coupon, the face value plays a crucial role in determining the bond’s value and subsequent coupon payments. It represents the principal amount borrowed by the issuer and repaid to the bondholder at maturity.
- Bond’s Principal
The face value essentially reflects the principal amount that the bondholder lends to the issuer when purchasing the bond.
- Coupon Calculation Basis
The face value serves as the base for calculating the bond’s coupon payments. The coupon rate, expressed as a percentage, is applied to the face value to determine the annual interest payment.
- Bond Pricing
The face value is a key factor in determining the bond’s price in the market. Bonds with higher face values tend to have higher prices, assuming other factors remain constant.
- Maturity Value
At the bond’s maturity date, the issuer repays the face value to the bondholder, marking the end of the bond’s life cycle.
Understanding the significance of face value empowers investors to accurately calculate the long coupon and make informed investment decisions. By considering the face value alongside other factors such as the coupon rate and maturity date, investors can assess the potential returns and risks associated with different bonds and strategize their bond portfolio accordingly.
Maturity Date
The maturity date of a bond is the date on which the principal amount, or face value, of the bond is repaid to the bondholder. It is a crucial component of how to calculate the long coupon, as it determines the duration over which the bond will pay interest and the final repayment of the principal.
The maturity date directly affects the calculation of the long coupon because it determines the number of coupon payments that will be made over the life of the bond. The long coupon is the total amount of interest that a bond will pay over its lifetime. To calculate the long coupon, the coupon rate (expressed as a percentage) is multiplied by the face value of the bond and then by the number of years until the maturity date.
For example, consider a bond with a face value of $1,000, a coupon rate of 5%, and a maturity date of 10 years. The annual coupon payment would be $50 ($1,000 x 5%). The long coupon would be $500 ($50 x 10 years).
Understanding the relationship between the maturity date and how to calculate the long coupon is essential for investors to accurately assess the potential returns and risks associated with different bonds. By considering the maturity date alongside other factors such as the coupon rate and face value, investors can make informed investment decisions about which bonds to buy and sell.
Payment Frequency
Payment frequency is a crucial aspect of how to calculate the long coupon. It refers to the number of times per year that a bond pays interest to its holders and influences the total amount of interest received over the bond’s lifetime.
- Annual
Bonds that pay interest once a year are the most common type, and their long coupon is calculated as the face value multiplied by the coupon rate.
- Semi-Annual
Bonds that pay interest twice a year have a slightly higher long coupon because the interest is compounded more frequently.
- Quarterly
Bonds that pay interest four times a year have an even higher long coupon due to the increased compounding effect.
- Monthly
Bonds that pay interest monthly have the highest long coupon because the interest is compounded most frequently.
Understanding the impact of payment frequency on how to calculate the long coupon empowers investors to make informed investment decisions. By considering payment frequency alongside other factors such as the coupon rate and maturity date, investors can select bonds that align with their specific investment goals and risk tolerance.
Accrued Interest
Accrued interest is critical to understanding how to calculate a long coupon. It refers to the interest that has been earned on a bond but not yet paid out to the bondholder. Accrued interest accumulates daily and is typically paid out along with the regular coupon payment.
Accrued interest arises because bond interest payments are usually made semi-annually or annually, while interest accrues continuously. This means that when a bond is purchased between coupon payment dates, the buyer is entitled to receive the accrued interest that has accumulated since the last payment date.
Accrued interest is calculated by multiplying the bond’s annual coupon rate by the face value of the bond and then by the number of days since the last coupon payment date, divided by the number of days in the year (365 or 366).
Understanding accrued interest is essential for accurate bond pricing and yield calculations. It also helps investors determine the total return they will receive when they purchase a bond.
Yield to Maturity
Yield to Maturity (YTM) holds a central position in understanding how to calculate the long coupon. It represents the annualized return an investor can expect to earn if they hold a bond until its maturity date. YTM considers the bond’s coupon payments, current market price, and time to maturity.
The relationship between YTM and how to calculate the long coupon is reciprocal. YTM influences the value of the long coupon, as higher YTM leads to lower bond prices and vice versa. This is because investors demand a higher return for bonds with longer maturities and higher risks.
Real-life examples abound. Consider a bond with a face value of $1,000, a coupon rate of 5%, and a maturity of 10 years. If the current market price is $900, the YTM is approximately 6.14%. This means that an investor who purchases the bond for $900 can expect to earn a 6.14% annual return if they hold the bond until maturity.
Understanding the connection between YTM and how to calculate the long coupon empowers investors to make informed investment decisions. By considering YTM alongside other factors such as the coupon rate and maturity date, investors can assess the potential return and risk of different bonds and strategize their bond portfolio accordingly.
Present Value
In the realm of how to calculate the long coupon, Present Value (PV) plays a pivotal role in determining the current worth of future cash flows associated with a bond. By discounting these future cash flows back to the present using an appropriate discount rate, investors can assess the bond’s intrinsic value and make informed investment decisions.
- Time Value of Money
PV underscores the principle that money available today is worth more than the same amount in the future due to its potential earning power.
- Discount Rate
The discount rate represents the rate at which future cash flows are discounted back to the present. It reflects the investor’s required rate of return and the perceived risk associated with the bond.
- Coupon Payments
PV considers the present value of all future coupon payments to be received over the bond’s life. This component captures the periodic interest payments that the bond will generate.
- Maturity Value
The maturity value, or face value, of the bond is also discounted back to the present. This represents the lump sum repayment of the principal at the end of the bond’s term.
Understanding the significance of these facets of PV empowers investors to accurately calculate the long coupon and gauge the overall value of a bond. By considering PV alongside other factors such as yield to maturity and credit risk, investors can optimize their bond portfolios for their specific investment goals and risk tolerance.
Bond Pricing
Bond pricing is a fundamental aspect of accurately calculating the long coupon and is influenced by various factors that determine the value of a bond. Understanding the key components of bond pricing enables investors to make informed decisions about bond investments.
- Face Value
The face value, or principal, of a bond is the amount that the issuer initially borrows and promises to repay at maturity. It serves as the basis for calculating the bond’s coupon payments and forms a key part of the long coupon calculation.
- Coupon Rate
The coupon rate represents the annual interest payment made to bondholders as a percentage of the face value. It directly impacts the value of the bond, with higher coupon rates typically leading to higher bond prices.
- Maturity Date
The maturity date indicates when the bond reaches its end and the issuer repays the face value to bondholders. It influences the long coupon calculation as the number of coupon payments is determined by the time until maturity.
- Yield to Maturity (YTM)
YTM is the annualized return an investor can expect to earn if they hold the bond until maturity. It’s a crucial factor in bond pricing as it reflects the market’s perception of the bond’s risk and return profile.
The interplay of these factors highlights the dynamic nature of bond pricing and its impact on the long coupon calculation. By understanding these components, investors can better assess the value and potential returns of bonds, enabling them to construct well-informed investment strategies.
Coupon Payment Calculation
Coupon payment calculation lies at the heart of understanding how to calculate the long coupon. It involves determining the periodic interest payments made to bondholders over the life of a bond. Accurate calculation of coupon payments is essential for assessing the overall value and return potential of a bond investment.
- Coupon Rate
The coupon rate specified in the bond’s terms determines the annual interest payment as a percentage of the face value. It directly influences the coupon payment calculation and, consequently, the long coupon.
- Face Value
The face value, or principal amount, of the bond serves as the base for calculating the coupon payment. The coupon rate is applied to the face value to determine the dollar amount of each interest payment.
- Payment Frequency
The frequency of coupon payments, whether annually, semi-annually, or quarterly, impacts the calculation of the long coupon. More frequent payments result in more frequent compounding and a higher long coupon.
- Maturity Date
The maturity date of the bond determines the duration over which coupon payments will be made. The number of coupon payments is calculated based on the time between the issue date and the maturity date.
Understanding these facets of coupon payment calculation empowers investors to accurately determine the long coupon for a given bond. It helps in assessing the cash flow stream generated by the bond, comparing different bond options, and making informed investment decisions based on individual risk tolerance and financial goals.
Long Coupon versus Short Coupon
Understanding the distinction between long coupons and short coupons is crucial in the context of “how to calculate the long coupon.” This distinction influences the bond’s overall value and return potential.
- Coupon Period
Long coupons represent bonds with longer periods between interest payments, typically semi-annually or annually. Short coupons, on the other hand, refer to bonds with shorter periods between interest payments, such as quarterly or monthly.
- Interest Payment Frequency
Long coupons result in less frequent interest payments compared to short coupons. This difference impacts the compounding effect and, consequently, the overall long coupon calculation.
- Bond Price Impact
Bonds with long coupons tend to have lower prices compared to bonds with short coupons due to the less frequent interest payments. This is because investors prefer more frequent interest payments, leading to higher demand for short coupon bonds.
- Yield Curve Relationship
The relationship between long and short coupons is influenced by the yield curve. When the yield curve is upward sloping, long coupon bonds may be more attractive as they offer higher yields compared to short coupon bonds. Conversely, when the yield curve is downward sloping, short coupon bonds may be more desirable due to their lower yields.
Comprehending the nuances of long coupons versus short coupons is essential for investors to make informed decisions when evaluating and selecting bonds. By considering the implications of coupon periods, interest payment frequency, bond prices, and the yield curve, investors can optimize their bond portfolios and align their investment strategies with their financial goals and risk tolerance.
Frequently Asked Questions about Calculating the Long Coupon
The following FAQs address common questions and misconceptions surrounding the calculation of the long coupon, providing clarity and practical insights.
Question 1: What factors influence the long coupon calculation?
The long coupon is determined by the face value of the bond, the coupon rate, the payment frequency, and the maturity date.
Question 2: How does the coupon period impact the long coupon?
Bonds with longer coupon periods, such as semi-annual or annual payments, result in a lower long coupon compared to bonds with shorter coupon periods, such as quarterly or monthly payments.
Question 3: What is the relationship between the yield curve and the long coupon?
When the yield curve is upward sloping, long coupon bonds may be more attractive due to higher yields. Conversely, when the yield curve is downward sloping, short coupon bonds may be more desirable due to lower yields.
Question 4: How can I calculate the accrued interest on a bond?
Accrued interest is calculated by multiplying the bond’s annual coupon rate by the face value and the number of days since the last coupon payment date, divided by the number of days in a year.
Question 5: What is the difference between a long coupon and a short coupon?
Long coupons represent bonds with longer periods between interest payments, typically semi-annually or annually, while short coupons represent bonds with shorter periods between interest payments, such as quarterly or monthly.
Question 6: How can I use the long coupon to assess the value of a bond?
The long coupon provides insights into the bond’s cash flow stream and overall return potential, enabling investors to compare different bond options and make informed investment decisions.
These FAQs offer a deeper understanding of how to calculate the long coupon, empowering investors to navigate the complexities of bond valuation and make well-informed investment choices.
In the next section, we will explore advanced strategies for utilizing the long coupon in bond portfolio management and risk assessment.
Tips for Calculating the Long Coupon
Accurately calculating the long coupon is crucial for informed bond investment decisions. Here are some practical tips to enhance your understanding and execution:
Tip 1: Understand the Bond’s Features
Determine the face value, coupon rate, payment frequency, and maturity date of the bond, as these factors directly impact the long coupon calculation.
Tip 2: Calculate the Annual Coupon Payment
Multiply the bond’s face value by its coupon rate to determine the annual interest payment.
Tip 3: Determine the Number of Coupon Payments
Divide the bond’s maturity date by the payment frequency to calculate the number of coupon payments to be received.
Tip 4: Multiply Annual Coupon Payment by the Number of Payments
To calculate the long coupon, multiply the annual coupon payment by the number of coupon payments.
Tip 5: Consider Accrued Interest
If you purchase a bond between coupon payment dates, calculate the accrued interest and add it to the long coupon.
Tip 6: Use a Bond Calculator
Utilize online bond calculators to simplify the long coupon calculation process and avoid errors.
Tip 7: Compare Bonds with Different Coupons
By calculating the long coupon for various bonds, you can compare their potential returns and make informed investment choices.
Tip 8: Monitor Changes in Interest Rates
Fluctuations in interest rates can impact the value of bonds, so monitor these changes to assess the potential impact on your bond investments.
By following these tips, you can effectively calculate the long coupon and enhance your understanding of bond valuation and investment strategies.
In the conclusion, we will summarize the key points and discuss the significance of the long coupon in optimizing bond portfolios and managing investment risks.
Conclusion
Understanding how to calculate the long coupon is a fundamental skill for investors seeking to make informed decisions in the bond market. This article has explored the intricacies of the calculation process, emphasizing the importance of factors such as the bond’s face value, coupon rate, payment frequency, and maturity date. By comprehending these elements, investors can accurately determine the total interest payments they will receive over the life of a bond.
The long coupon plays a crucial role in bond valuation and portfolio management. It provides a basis for comparing the returns of different bonds, assessing the impact of interest rate changes, and making strategic investment choices. Furthermore, understanding the long coupon is essential for evaluating a bond’s risk profile and determining its suitability for an investor’s financial goals and risk tolerance.