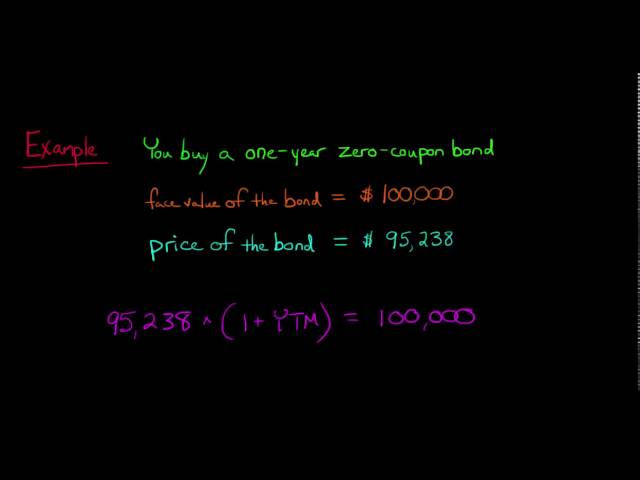Unveiling the Zero Coupon Bond: A Comprehensive Guide
A zero coupon bond, an intriguing financial instrument, offers investors a unique way to participate in fixed-income markets. Unlike traditional bonds that pay periodic interest payments, zero coupon bonds are issued at a deep discount to their face value and do not pay any interest during their life. This bond’s value appreciates steadily over time, with investors receiving the full face value upon maturity.
Zero coupon bonds provide advantages such as simplified cash flow streams, tax benefits, and diversification opportunities. Historically, the introduction of zero coupon bonds in the 1980s revolutionized the fixed-income landscape, opening up new investment possibilities for individuals and institutions alike.
This article delves into the intricacies of zero coupon bonds, exploring their mechanisms, valuation techniques, and strategic applications within investment portfolios.
How to Calculate Zero Coupon Bond
Understanding the nuances of zero coupon bond calculations is crucial for investors seeking to harness their unique characteristics. Key aspects to consider include:
- Face Value
- Maturity Date
- Yield to Maturity
- Discount Rate
- Present Value
- Accrued Interest
- Tax Implications
- Investment Horizon
These aspects are interconnected and influence the overall return and risk profile of zero coupon bonds. For instance, the yield to maturity determines the discount rate used to calculate the present value, which in turn affects the bond’s price. Understanding the interplay between these factors empowers investors to make informed decisions when incorporating zero coupon bonds into their portfolios.
Face Value
The face value of a zero coupon bond, also known as its par value or maturity value, represents the amount that the bondholder will receive upon its maturity date. It is a critical component in the calculation of the bond’s price, which is significantly discounted from the face value due to the lack of periodic interest payments.
The relationship between face value and the calculation of zero coupon bond price is inversely proportional. A higher face value results in a higher discounted price for the bond, as the present value of the future face value payment is spread over a longer period of time. Conversely, a lower face value leads to a lower discounted price.
For instance, consider two zero coupon bonds with the same maturity date and yield to maturity. One bond has a face value of $1,000, while the other has a face value of $5,000. The bond with the higher face value will have a higher present value and thus a higher purchase price than the bond with the lower face value. This is because the investor is essentially lending a larger sum of money to the bond issuer in exchange for the same future return.
Understanding the connection between face value and zero coupon bond calculation is crucial for investors to assess the potential return and risk associated with these bonds. By considering the face value in conjunction with other factors such as maturity date and yield to maturity, investors can make informed decisions about the suitability of zero coupon bonds within their portfolios.
Maturity Date
The maturity date of a zero coupon bond holds significant importance in its calculation. It represents the specific date on which the bond reaches its end and the holder receives the face value. This aspect directly influences the bond’s price and yield to maturity, which are crucial factors in determining its attractiveness to investors.
The maturity date serves as a critical component in calculating the present value of the bond’s future cash flow. Since zero coupon bonds do not pay periodic interest payments, the entire face value is discounted back to the present using the yield to maturity. The time period between the purchase date and the maturity date determines the length of this discounting period, thereby impacting the bond’s price.
For instance, consider two zero coupon bonds with identical face values but different maturity dates. The bond with a shorter maturity date will have a higher present value compared to the bond with a longer maturity date, assuming the same yield to maturity. This is because the shorter time period results in less discounting, leading to a higher present value for the bond.
Understanding the relationship between maturity date and zero coupon bond calculation empowers investors to make informed investment decisions. By considering the maturity date in conjunction with other factors such as face value and yield to maturity, investors can assess the potential return and risk associated with these bonds. This understanding aids in portfolio construction, allowing investors to align their investment horizon with the maturity dates of zero coupon bonds to meet their financial goals.
Yield to Maturity
Yield to Maturity (YTM) is a critical concept in the calculation of zero coupon bonds. It represents the annualized rate of return an investor expects to receive if they hold the bond until its maturity date. YTM is a crucial factor in determining the present value and attractiveness of zero coupon bonds, influencing investment decisions.
- Internal Rate of Return (IRR)
YTM is essentially the IRR of a zero coupon bond, taking into account the bond’s purchase price, face value, and maturity date. It represents the effective annual return on the investment.
- Discount Rate
YTM is used as the discount rate to calculate the present value of the bond’s future cash flow. Higher YTMs lead to lower present values, while lower YTMs result in higher present values.
- Market Conditions
YTM is influenced by market conditions, such as interest rates and inflation expectations. Changes in YTM can impact the price and attractiveness of zero coupon bonds.
- Tax Implications
YTM can affect the tax liability of investors, as accrued interest on zero coupon bonds is subject to taxation even though it is not paid out until maturity.
Understanding YTM is essential for investors seeking to evaluate and compare zero coupon bonds. By considering YTM in conjunction with other factors such as face value and maturity date, investors can make informed decisions that align with their financial goals and risk tolerance.
Discount Rate
Discount rate plays a critical role in the calculation of zero coupon bonds, as it determines the present value of the bond’s future cash flow. The discount rate is essentially the yield to maturity (YTM) of the bond, which represents the annualized rate of return an investor expects to receive if they hold the bond until its maturity date.
The discount rate is a crucial component of calculating zero coupon bond prices because it is used to discount the bond’s future face value back to its present value. This is important because zero coupon bonds do not pay periodic interest payments, so their entire return is realized at maturity. Therefore, the discount rate directly affects the bond’s price, with higher discount rates leading to lower bond prices and vice versa.
For example, consider a zero coupon bond with a face value of $1,000 and a maturity of 10 years. If the YTM is 5%, the present value of the bond would be $613.91. This is because the $1,000 face value is discounted back to its present value using the 5% discount rate over the 10-year period.
Understanding the relationship between discount rate and zero coupon bond calculation is essential for investors to make informed investment decisions. By considering the discount rate in conjunction with other factors such as face value and maturity date, investors can assess the potential return and risk associated with these bonds and determine their suitability within their portfolios.
Present Value
In the context of calculating zero coupon bonds, present value (PV) plays a pivotal role in determining their worth. It represents the current value of the bond’s future cash flow, discounted back to the present using a specific discount rate. This concept forms the foundation for understanding and evaluating zero coupon bonds.
- Face Value: The face value, or maturity value, is the amount the bondholder receives at maturity. It serves as the future cash flow that is discounted to calculate the bond’s present value.
- Maturity Date: The maturity date is the specific point in time when the bond matures, and the face value is paid out. This factor influences the duration over which the future cash flow is discounted, affecting the present value.
- Discount Rate: The discount rate, typically the bond’s yield to maturity (YTM), is the rate used to discount the future cash flow back to the present. A higher discount rate results in a lower present value, and vice versa.
- Time Value of Money: The time value of money principle underlies the calculation of present value. It acknowledges that money received in the future is worth less than money received today, as it has the potential to earn interest over time.
Understanding the interplay between these facets enables investors to accurately determine the present value of zero coupon bonds. This knowledge is crucial for assessing their potential return and making informed investment decisions. By considering the present value in conjunction with other factors, investors can optimize their investment strategies and align their portfolios with their financial goals.
Accrued Interest
In the context of zero coupon bonds, accrued interest plays a crucial role in their calculation. Unlike traditional coupon bonds that pay periodic interest payments, zero coupon bonds do not make any interest payments until maturity. However, they still accrue interest over time, which affects their present value and yield to maturity.
The accrued interest on a zero coupon bond represents the portion of the bond’s face value that has been earned but not yet paid out. It is calculated daily based on the bond’s yield to maturity and the number of days since the last interest payment date or the issuance date for newly issued bonds. As the bond approaches maturity, the accrued interest accumulates, increasing the bond’s present value.
Understanding the concept of accrued interest is critical for accurately calculating the present value and yield to maturity of zero coupon bonds. It allows investors to assess the bond’s true value and make informed investment decisions. For example, if an investor purchases a zero coupon bond with a face value of $1,000 and a yield to maturity of 5%, the accrued interest after one year would be $50. This accrued interest would be added to the bond’s purchase price to determine its current market value.
In summary, accrued interest is an integral component of calculating zero coupon bonds. By considering the accrued interest, investors can accurately assess the bond’s present value, yield to maturity, and potential return, enabling them to make informed investment decisions and optimize their portfolio strategies.
Tax Implications
Tax implications play a crucial role in the calculation of zero coupon bonds. Unlike traditional coupon bonds that pay periodic interest payments, zero coupon bonds do not make any interest payments until maturity. However, the Internal Revenue Service (IRS) considers the accrued interest on zero coupon bonds to be taxable income, even though it is not received until the bond matures. This unique tax treatment has significant implications for investors and affects the overall return and yield to maturity of zero coupon bonds.
The accrued interest on a zero coupon bond increases annually, and investors are required to pay taxes on this accrued interest even though they have not received any cash payments. This can lead to a situation where investors are paying taxes on income they have not yet received. For example, if an investor purchases a zero coupon bond with a face value of $1,000 and a yield to maturity of 5%, they will have to pay taxes on the $50 of accrued interest each year, even though they will not receive any cash payments until the bond matures.
Understanding the tax implications of zero coupon bonds is critical for investors to accurately assess their potential return and make informed investment decisions. Investors need to consider the impact of taxes on the overall yield to maturity and factor in the potential tax liability when evaluating zero coupon bonds. It is also important to note that the tax treatment of zero coupon bonds can vary depending on the investor’s tax bracket and other factors, so it is advisable to consult with a tax professional for personalized advice.
Investment Horizon
In the context of calculating zero coupon bonds, investment horizon holds great significance, influencing the overall approach and considerations involved. It refers to the period of time for which an investor intends to hold the bond, impacting factors such as yield to maturity and overall return.
- Bond Maturity:
The maturity date of the bond directly affects the investment horizon. Investors need to align their investment horizon with the bond’s maturity to maximize returns and minimize risks.
- Interest Rate Expectations:
Anticipated changes in interest rates can impact investment decisions. Investors with a shorter investment horizon may be less affected by interest rate fluctuations compared to those with a longer horizon.
- Financial Goals:
Investment horizon is closely tied to an investor’s financial goals. Zero coupon bonds can be tailored to meet specific goals, such as retirement planning or funding a child’s education.
- Risk Tolerance:
Investors with a lower risk tolerance may prefer shorter investment horizons to minimize potential losses, while those with a higher risk tolerance may be willing to commit to longer horizons for higher potential returns.
Understanding the concept of investment horizon is crucial for calculating zero coupon bonds accurately. By considering their investment horizon, investors can make informed decisions that align with their financial objectives and risk appetite.
FAQs on Zero Coupon Bond Calculations
This section addresses frequently asked questions and clarifies key aspects of calculating zero coupon bonds.
Question 1: What is the formula for calculating the present value of a zero coupon bond?
Answer: Present Value = Face Value / (1 + Yield to Maturity)^n, where n is the number of years to maturity.
Question 2: How does the yield to maturity affect the bond’s price?
Answer: A higher yield to maturity results in a lower bond price, and vice versa.
Question 3: What is the relationship between maturity date and present value?
Answer: The longer the maturity date, the lower the present value of the bond.
Question 4: How is accrued interest calculated for zero coupon bonds?
Answer: Accrued interest is calculated daily as a percentage of the bond’s face value based on the yield to maturity.
Question 5: What tax implications should investors be aware of?
Answer: Accrued interest on zero coupon bonds is taxable even though it is not received until maturity.
Question 6: How does investment horizon impact zero coupon bond calculations?
Answer: Investors’ investment horizon should align with the bond’s maturity to optimize returns and manage risks.
These FAQs provide a concise overview of key considerations in calculating zero coupon bonds. Understanding these concepts is essential for investors to make informed decisions and effectively incorporate these bonds into their portfolios.
In the next section, we will delve into advanced strategies for maximizing returns and managing risks associated with zero coupon bonds.
Tips for Maximizing Returns and Managing Risks with Zero Coupon Bonds
This section provides actionable tips to enhance your investment strategy involving zero coupon bonds:
Tip 1:Assess Investment Horizon: Align the bond’s maturity date with your investment goals and risk tolerance.
Tip 2:Consider Interest Rate Environment: Anticipate changes in interest rates and their potential impact on bond prices.
Tip 3:Diversify Your Portfolio: Incorporate zero coupon bonds alongside other investments to reduce overall risk.
Tip 4:Monitor Market Conditions: Stay informed about economic and financial events that may affect bond values.
Tip 5:Understand Tax Implications: Be aware of the unique tax treatment of accrued interest on zero coupon bonds.
Tip 6:Calculate Yield to Maturity Accurately: Use precise calculations to determine the bond’s true yield and make informed decisions.
Tip 7:Consider Inflation Protection: Explore TIPS (Treasury Inflation-Protected Securities) to hedge against inflation risk.
Tip 8:Seek Professional Advice: Consult a financial advisor for personalized guidance and portfolio optimization.
By implementing these tips, you can enhance your understanding of zero coupon bonds and make strategic decisions to maximize returns while managing potential risks.
In the concluding section, we will explore advanced strategies for incorporating zero coupon bonds into your portfolio and achieving your financial objectives.
Conclusion
In summary, understanding the nuances of zero coupon bond calculations empowers investors to harness their unique characteristics effectively. Key factors to consider include face value, maturity date, yield to maturity, present value, and tax implications. By grasping the interplay between these factors and employing advanced strategies, investors can maximize returns and manage risks.
Zero coupon bonds offer distinct advantages and can play a significant role in portfolio diversification. Their lack of periodic interest payments and unique tax treatment necessitate a thorough understanding of their calculation methods. This article has delved into the intricacies of zero coupon bond calculations, providing investors with the tools and insights to make informed decisions.