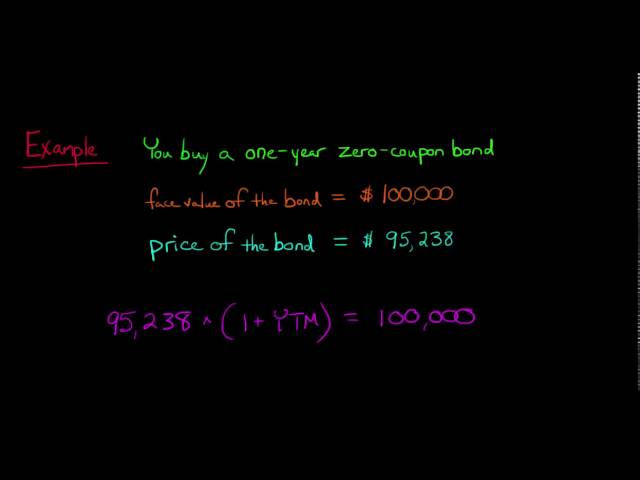Zero coupon bond yield is a calculation that helps investors evaluate the value of fixed-income securities that do not make periodic interest payments, also known as “zero-coupon bonds”. An example would be a 20-year bond with a face value of $1000 that is currently worth $300.
Calculating zero coupon bond yield is crucial because it provides investors with a clear understanding of the bond’s potential return. It allows investors to compare different bonds and make informed investment decisions. Historically, the development of zero-coupon bonds in the 1980s revolutionized the fixed-income market by providing investors with a new asset class offering unique risk and return characteristics.
This article explores the key considerations and steps involved in calculating zero coupon bond yield, providing readers with valuable insights into this essential financial concept.
How to Calculate Zero Coupon Bond Yield
Calculating zero coupon bond yield is essential for investors to evaluate the potential return and make informed investment decisions. Key aspects to consider include:
- Face value
- Maturity date
- Current price
- Yield to maturity
- Present value
- Time to maturity
- Interest rate
- Coupon rate
Understanding these aspects allows investors to accurately calculate zero coupon bond yield, compare different bonds, and assess their risk and return profiles. For example, the yield to maturity considers the time value of money and the present value of the bond’s future cash flows, providing a comprehensive measure of the bond’s return.
Face value
Within the context of calculating zero coupon bond yield, “Face value” plays a crucial role. It represents the principal amount borrowed by the bond issuer and repaid to the bondholder at maturity. Understanding its various aspects is essential for accurate yield calculations.
- Nominal value: The stated value of the bond, which is printed on the bond certificate. This value represents the amount that the bondholder will receive upon maturity.
- Par value: Synonymous with “Face value”, it signifies the redemption value of the bond at maturity. Zero-coupon bonds are typically issued at a discount to their Face value and mature at their Face value.
- Present value: The current worth of the bond’s future cash flows, calculated using the bond’s yield to maturity and time to maturity. The Face value is a key input in this calculation.
- Implied yield: The yield that equates the bond’s present value to its market price. This yield is important for investors to determine the potential return on their investment.
By considering these multifaceted aspects of Face value, investors can gain a comprehensive understanding of its significance in calculating zero coupon bond yield. These insights empower investors to make informed decisions and effectively evaluate the risk and return profiles of these fixed-income securities.
Maturity date
When calculating zero coupon bond yield, the “Maturity date” signifies the specific date on which the bond reaches its end and the principal amount becomes payable to the bondholder. Understanding the significance of Maturity date and its interrelation with yield calculation is pivotal for informed investment decisions.
The Maturity date serves as a critical component in determining the bond’s yield to maturity (YTM). YTM represents the annualized rate of return an investor can expect to earn if they hold the bond until its Maturity date. The time period between the bond’s issuance and Maturity date is known as its “term to maturity”. A bond’s YTM and price are inversely related: as the YTM increases, the bond’s price decreases, and vice versa.
For instance, consider a zero-coupon bond with a Face value of $1,000 and a Maturity date five years from now. If the current market price of the bond is $783.53, the YTM can be calculated using the following formula: Present Value = Face Value / (1 + YTM)^Term to Maturity Solving for YTM, we get: YTM = ( Face Value / Present Value)^(1/Term to Maturity) – 1 Plugging in the values, we find that the bond’s YTM is approximately 4.5%. This means that if an investor purchases the bond for $783.53 and holds it until its Maturity date, they will earn an annualized return of 4.5%.
By understanding the connection between Maturity date and zero coupon bond yield, investors can effectively evaluate the potential return and make informed investment choices. This knowledge empowers them to construct diversified portfolios that align with their risk tolerance and financial goals.
Current price
Within the realm of calculating zero coupon bond yield, “Current price” holds significant importance as a critical component that directly influences the yield calculation. The current price of a zero-coupon bond represents the market value at which it is currently being traded. It serves as the present value of the bond’s future cash flows, discounted at the prevailing market interest rate.
The relationship between current price and zero coupon bond yield is inverse. As the current price of the bond increases, the yield to maturity (YTM) decreases, and vice versa. This inverse relationship stems from the mathematical formula used to calculate yield to maturity: YTM = ( Face Value / Current Price)^(1/Term to Maturity) – 1 Where: – YTM is the yield to maturity – Face Value is the principal amount repaid at maturity – Current Price is the current market price of the bond – Term to Maturity is the time remaining until the bond matures
Practical applications of understanding this relationship are evident in fixed-income portfolio management. Investors can strategically buy or sell zero-coupon bonds to adjust the duration and yield profile of their portfolios. For instance, if an investor anticipates rising interest rates, they may sell zero-coupon bonds to lock in their current yield and avoid potential losses due to price declines.
In summary, the current price of a zero-coupon bond plays a crucial role in calculating its yield to maturity. By understanding this relationship, investors can make informed decisions when buying or selling zero-coupon bonds to optimize their investment portfolios.
Yield to maturity
Yield to maturity (YTM) is a crucial concept in fixed income investing and plays a significant role in calculating zero coupon bond yield. It represents the annualized rate of return an investor can expect to earn if they hold the bond until its maturity date. The calculation of YTM involves several key facets:
- Internal rate of return (IRR): YTM is essentially the IRR of an investment in a zero-coupon bond. It represents the discount rate that equates the present value of the bond’s future cash flows to its current market price.
- Time to maturity: The number of years until the bond reaches its maturity date. YTM is inversely related to time to maturity, meaning longer-term bonds generally have higher yields.
- Market interest rates: YTM is influenced by prevailing market interest rates. When interest rates rise, the YTM of existing bonds tends to fall, and vice versa.
- Default risk: The creditworthiness of the bond issuer can impact YTM. Bonds issued by higher-risk issuers typically have higher yields to compensate investors for the increased risk of default.
Understanding these facets is essential for accurately calculating zero coupon bond yield. YTM is a key metric that helps investors compare different bonds and make informed investment decisions. It provides insights into the potential return and risk associated with a particular bond and enables investors to construct diversified portfolios that align with their financial goals.
Present value
Present value plays a central role in the calculation of zero coupon bond yield. It represents the current worth of the bond’s future cash flows, discounted at the prevailing market interest rate. This concept is crucial for understanding how zero coupon bond yield is determined and its implications for investors.
The present value of a zero-coupon bond is calculated using the following formula: Present Value = Face Value / (1 + Yield to Maturity)^Term to Maturity Where: – Present Value is the current market price of the bond – Face Value is the principal amount repaid at maturity – Yield to Maturity is the annualized rate of return an investor can expect to earn if they hold the bond until its maturity date – Term to Maturity is the time remaining until the bond matures
By understanding the relationship between present value and yield to maturity, investors can make informed decisions when buying or selling zero-coupon bonds. For instance, if an investor anticipates rising interest rates, they may sell zero-coupon bonds to lock in their current yield and avoid potential losses due to price declines.
In summary, present value is a critical component of calculating zero coupon bond yield, providing insights into the bond’s current value and potential return. By understanding this relationship, investors can effectively manage their fixed-income portfolios and make informed investment decisions.
Time to maturity
In the realm of fixed-income securities, “Time to maturity” holds paramount importance in determining the yield of zero-coupon bonds. This concept represents the duration until a bond reaches its maturity date, significantly influencing its present value and overall return. Understanding the various facets of “Time to maturity” is essential for accurate yield calculations and informed investment decisions.
- Term to maturity: Calculated as the number of years or months remaining until the bond matures. It is a crucial input in yield-to-maturity calculations.
- Yield curve: Depicts the relationship between interest rates and time to maturity for bonds with similar credit risk. It serves as a benchmark for determining the appropriate yield to maturity for a given bond.
- Interest rate risk: Longer time to maturity generally implies higher interest rate risk. Interest rate fluctuations can impact bond prices, affecting the potential return for investors.
- Reinvestment risk: For zero-coupon bonds, reinvestment risk arises as investors must reinvest the proceeds from maturing bonds at prevailing market interest rates, which may be lower than the bond’s yield to maturity.
Comprehending these facets of “Time to maturity” empowers investors to make informed Entscheidungen when evaluating zero-coupon bonds. By considering the term to maturity, yield curve, interest rate risk, and reinvestment risk, investors can effectively calculate yield to maturity and make strategic investment decisions that align with their financial goals and risk tolerance.
Interest rate
Interest rate stands as a critical factor in calculating zero coupon bond yield, influencing the bond’s present value and overall return. Understanding its multifaceted nature is essential for accurate yield calculations and informed investment decisions.
- Discount rate: Represents the interest rate used to discount the bond’s future cash flows to determine its present value.
- Market interest rate: The prevailing interest rate in the market, which influences the bond’s yield to maturity.
- Yield curve: Depicts the relationship between interest rates and time to maturity for bonds with similar credit risk.
- Reinvestment rate: The interest rate at which investors can reinvest the proceeds from maturing bonds, impacting the overall return.
By considering these facets of interest rate, investors can effectively calculate zero coupon bond yield and make strategic investment decisions aligned with their financial goals and risk tolerance. For instance, a higher market interest rate generally leads to a lower yield to maturity for zero-coupon bonds, as investors demand higher returns for longer-term investments. Conversely, a lower market interest rate can result in a higher yield to maturity, making zero-coupon bonds more attractive to investors seeking higher returns.
Coupon rate
When calculating zero coupon bond yield, understanding “Coupon rate” is not applicable as zero coupon bonds do not pay periodic interest payments like traditional coupon bonds. Zero-coupon bonds are issued at a discount to their face value and mature at their face value, providing a return to investors through the difference between the purchase price and the maturity value.
In conclusion, while “Coupon rate” is a crucial factor in calculating the yield of traditional coupon bonds, it is not directly relevant to the calculation of zero coupon bond yield. Investors interested in zero-coupon bonds should focus on factors such as face value, maturity date, current price, yield to maturity, present value, and time to maturity to accurately assess the potential return and risk associated with these fixed-income securities.
FAQs on Calculating Zero Coupon Bond Yield
This FAQ section addresses common questions and provides clarity on essential aspects of calculating zero coupon bond yield.
Question 1: What is the formula for calculating zero coupon bond yield?
The formula is: Yield to Maturity = (Face Value / Current Price)^(1/Term to Maturity) – 1, where Face Value is the principal amount repaid at maturity, Current Price is the current market price of the bond, and Term to Maturity is the time remaining until the bond matures.
Question 2: How does the current price of a zero-coupon bond affect its yield?
Current price and yield are inversely related. As the current price increases, the yield decreases, and vice versa.
Question 3: What is the relationship between time to maturity and yield?
Generally, longer time to maturity leads to higher yield, as investors demand higher returns for locking in their funds for an extended period.
Question 4: How can I use zero coupon bond yield to compare different bonds?
Yield to Maturity allows investors to compare the potential returns of different zero-coupon bonds and make informed investment decisions.
Question 5: What are some factors that can influence zero coupon bond yield?
Factors such as market interest rates, creditworthiness of the issuer, and economic conditions can impact zero coupon bond yield.
Question 6: How does reinvestment risk affect zero-coupon bond yield?
Reinvestment risk arises when investors must reinvest the proceeds from maturing zero-coupon bonds at prevailing market interest rates, which may differ from the bond’s yield to maturity.
These FAQs provide a concise overview of key considerations and formulas related to calculating zero coupon bond yield. A comprehensive understanding of these concepts is crucial for investors navigating the fixed-income market and making informed investment decisions.
In the next section, we will delve deeper into the practical applications of zero coupon bond yield and explore strategies for incorporating these bonds into investment portfolios.
Tips for Maximizing Zero Coupon Bond Yield
This section provides actionable tips to help investors optimize their returns when investing in zero coupon bonds.
Tip 1: Calculate yield to maturity accurately: Utilisez the formula and consider all relevant factors to determine the true yield of a zero-coupon bond.
Tip 2: Assess interest rate risk: Understand how changes in interest rates can impact zero-coupon bond prices and yields.
Tip 3: Consider reinvestment risk: Factor in the potential risks associated with reinvesting proceeds from maturing zero-coupon bonds.
Tip 4: Evaluate credit risk: Assess the creditworthiness of the bond issuer to gauge the likelihood of timely payment of principal.
Tip 5: Diversify your portfolio: Spread investments across a range of zero-coupon bonds with varying maturities and issuers to mitigate risk.
By following these tips, investors can enhance their understanding of zero coupon bond yield and make informed decisions that align with their financial goals and risk tolerance.
In the concluding section, we will explore advanced strategies for incorporating zero coupon bonds into investment portfolios, further empowering investors to navigate the fixed-income market effectively.
Conclusion
This comprehensive guide has provided a thorough exploration of how to calculate zero coupon bond yield, empowering investors with the knowledge and tools to make informed investment decisions. Key takeaways include the understanding that zero coupon bond yield is crucial for evaluating the potential return of these fixed-income securities. The calculation involves considering factors such as face value, maturity date, current price, yield to maturity, present value, and time to maturity.
By accurately calculating zero coupon bond yield, investors can compare different bonds, assess their risk and return profiles, and make strategic choices that align with their financial goals. This knowledge is especially valuable in the current market environment, where fixed-income investments play a vital role in portfolio diversification and risk management.