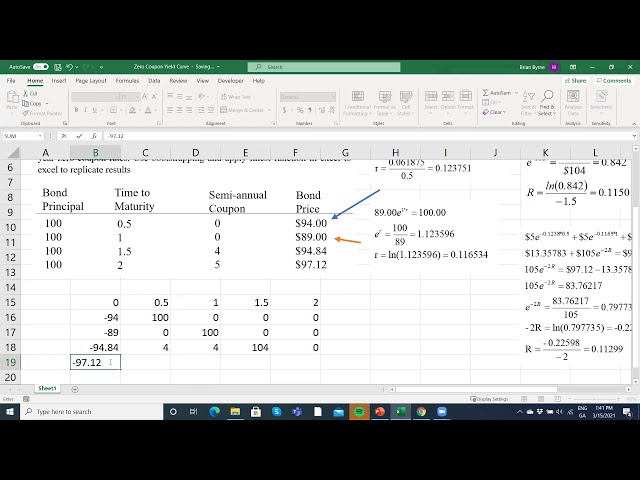
Calculating Zero Rate from Discount Factor: A Guide to Bond Valuation
Determining the zero rate, or risk-free rate, from a discount factor is essential for accurately valuing bonds in financial markets. Discount factors represent the present value of a future cash flow discounted at a specific rate, and understanding how to calculate the zero rate enables investors to assess bond yields and make informed investment decisions.
This concept holds great significance in fixed income analysis. By calculating the zero rate, investors can evaluate the true yield of a bond and compare it to other investment opportunities. Historically, the zero rate has played a crucial role in central bank policy decisions, affecting interest rate benchmarks and influencing economic growth.
How to Calculate Zero Rate from Discount Factor
Calculating the zero rate, or risk-free rate, from a discount factor is pivotal in fixed income analysis. Key aspects of this process include:
- Present value
- Future cash flows
- Discounting
- Bond yield
- Central bank policy
- Interest rate benchmarks
- Economic growth
- Investment decisions
Understanding these aspects enables investors to accurately value bonds, assess their yields, and make informed investment choices. The zero rate serves as a benchmark for bond pricing and plays a crucial role in central bank monetary policy decisions.
Present Value
Present value is a fundamental concept in finance, representing the value of a future sum of money today. In the context of calculating zero rate from a discount factor, present value plays a pivotal role in determining the risk-free rate used to discount future cash flows.
- Nominal Value: The face value or principal amount of the bond.
- Time Value of Money: The concept that money available today is worth more than the same amount of money in the future due to its earning potential.
- Compounding: The process of earning interest on interest, resulting in exponential growth over time.
- Discount Rate: The interest rate used to discount future cash flows back to their present value.
Understanding these facets of present value is essential for accurately calculating the zero rate and assessing bond yields. By considering the nominal value, time value of money, compounding, and discount rate, investors can effectively determine the risk-free rate and make informed investment decisions.
Future Cash Flows
In calculating the zero rate from a discount factor, future cash flows play a critical role. They represent the anticipated payments that an investor expects to receive from a bond investment over its lifetime. These cash flows include both periodic interest payments and the final principal repayment at maturity.
- Coupon Payments: Regular interest payments made by the bond issuer to the bondholder throughout the life of the bond.
- Maturity Value: The face value or principal amount of the bond, which is repaid to the bondholder at the end of the bond’s term.
- Timing and Frequency: Future cash flows are characterized by their timing and frequency. Bonds typically make semi-annual coupon payments and repay the principal at maturity.
- Default Risk: The possibility that the bond issuer may fail to make timely or full payments on the bond’s cash flows.
Understanding the nature and characteristics of future cash flows is essential for calculating the zero rate accurately. These cash flows, along with the discount factor, determine the present value of the bond and, consequently, its yield. By considering the timing, frequency, and default risk associated with future cash flows, investors can effectively assess the risk and return profile of a bond investment.
Discounting
Discounting is a fundamental concept in finance that involves reducing the value of future cash flows to their present value. In the context of calculating zero rate from a discount factor, discounting plays a crucial role in determining the risk-free rate used to assess bond yields and make informed investment decisions.
- Time Value of Money: The concept that money available today is worth more than the same amount in the future due to its potential earning power.
- Present Value: The value of a future sum of money today, calculated by discounting it back at a specific rate.
- Discount Factor: A multiplier used to discount future cash flows, representing the present value of $1 received at a specified future date.
- Zero Rate: The risk-free rate used to discount future cash flows, which is derived from the discount factor.
Understanding the principles of discounting is essential for accurately calculating the zero rate and assessing bond yields. By considering the time value of money, present value, and discount factor, investors can effectively evaluate the risk and return profile of a bond investment.
Bond Yield
Bond yield is a crucial concept in the financial markets and is intricately connected to the calculation of zero rate from discount factor. The bond yield, expressed as an annual percentage rate, represents the return an investor can expect to receive from a bond investment. It is calculated as the ratio of the bond’s annual coupon payments plus the change in its price to its market price. The discount factor, on the other hand, is a factor used to convert future cash flows to their present value. It is directly related to the bond yield, as it is calculated using the following formula:
Discount Factor = 1 / (1 + Bond Yield)^n
where n represents the number of years to maturity.
The bond yield is a critical component of calculating the zero rate from a discount factor. The zero rate is the risk-free rate, which is used to discount future cash flows back to their present value. By using the discount factor derived from the bond yield, investors can determine the present value of future cash flows and assess the bond’s overall value and attractiveness.
Central bank policy
Central bank policy plays a pivotal role in the calculation of zero rate from discount factor. The zero rate, also known as the risk-free rate, is the hypothetical interest rate at which risk-free loans can be made. It serves as the benchmark for pricing bonds and other fixed-income securities.
Central banks, such as the Federal Reserve in the United States, use various policy tools to influence the zero rate. By adjusting the federal funds rate, which is the interest rate at which banks lend to each other overnight, central banks can influence the overall level of interest rates in the economy. Changes in the federal funds rate directly affect the discount factor, which is used to calculate the zero rate.
For example, if the Federal Reserve increases the federal funds rate, it becomes more expensive for banks to borrow money. This, in turn, leads to higher interest rates on loans and bonds, resulting in a higher discount factor. Consequently, the zero rate, which is calculated using the discount factor, also increases.
Understanding the relationship between central bank policy and the calculation of zero rate from discount factor is essential for investors and financial analysts. By monitoring central bank announcements and policy decisions, investors can anticipate changes in the zero rate and make informed investment choices. This understanding also aids in assessing the overall health and direction of the economy, as central bank policy is often used to manage inflation, economic growth, and financial stability.
Interest rate benchmarks
Interest rate benchmarks are foundational in the calculation of zero rate from discount factor. The zero rate, also known as the risk-free rate, is the hypothetical interest rate at which risk-free loans can be made. It is calculated using the discount factor, which is derived from the prevailing interest rate benchmarks in the financial markets.
One of the most widely used interest rate benchmarks is the London Interbank Offered Rate (LIBOR). LIBOR represents the average interest rate at which banks lend to each other in the London interbank market. Other commonly used benchmarks include the Secured Overnight Financing Rate (SOFR) in the United States and the Euro Interbank Offered Rate (EURIBOR) in the eurozone.
Interest rate benchmarks play a critical role in the calculation of zero rate from discount factor, as they provide a reference point for determining the appropriate discount rate to use. Changes in interest rate benchmarks, such as those implemented by central banks to manage inflation or economic growth, directly affect the discount factor and, consequently, the zero rate. By understanding the relationship between interest rate benchmarks and the calculation of zero rate from discount factor, investors and financial analysts can make informed investment decisions and assess the impact of monetary policy on the financial markets.
Economic growth
Economic growth and the calculation of zero rate from discount factor are interconnected concepts within the realm of financial markets and economic analysis. The zero rate, also known as the risk-free rate, is a hypothetical interest rate used to discount future cash flows back to their present value. It serves as a benchmark for valuing bonds and other fixed-income securities.
Economic growth plays a crucial role in determining the zero rate. A growing economy typically leads to higher inflation expectations, which in turn lead to higher interest rates. This is because investors demand a higher return on their investments to compensate for the erosion of purchasing power due to inflation. As a result, the discount factor, which is derived from interest rates, also increases. Consequently, the zero rate, calculated using the discount factor, also rises.
Conversely, a slowing economy can lead to lower inflation expectations and a decrease in interest rates. This is because investors are less concerned about inflation eroding the value of their investments. As a result, the discount factor decreases, leading to a lower zero rate.
Understanding the relationship between economic growth and the calculation of zero rate from discount factor is essential for investors and financial analysts. By considering the impact of economic growth on interest rates and the discount factor, they can make informed investment decisions and assess the overall health and direction of the economy.
Investment decisions
Investment decisions are inextricably linked to the calculation of zero rate from discount factor. The zero rate, also known as the risk-free rate, plays a pivotal role in valuing bonds and other fixed-income securities. By understanding how to calculate the zero rate, investors can make informed investment decisions and assess the risk and return profile of different investment opportunities.
The zero rate serves as a benchmark for pricing bonds. A higher zero rate leads to a higher discount factor, which in turn results in a lower present value for future cash flows. This means that investors demand a higher return on their investments to compensate for the increased time value of money. Conversely, a lower zero rate leads to a lower discount factor and a higher present value for future cash flows, making bonds more attractive to investors.
Real-life examples of investment decisions that are influenced by the zero rate include:
Bond selection: Investors may choose to invest in bonds with longer maturities if they expect the zero rate to rise in the future, as this would lead to an increase in the present value of the bond’s future cash flows. Asset allocation: Investors may decide to allocate a greater portion of their portfolio to fixed-income securities if they anticipate a decrease in the zero rate, as this would make bonds more attractive relative to other asset classes. Interest rate hedging: Investors may use interest rate derivatives, such as futures or options, to hedge against the risk of interest rate fluctuations, which can affect the value of their bond investments.
Understanding the connection between investment decisions and the calculation of zero rate from discount factor is essential for investors seeking to optimize their returns and manage risk effectively. By considering the impact of the zero rate on bond prices and future cash flows, investors can make informed investment decisions that align with their financial goals and risk tolerance.
Frequently Asked Questions
This section addresses common questions and misconceptions surrounding the calculation of zero rate from discount factor, providing clarity and further insights into this important financial concept.
Question 1: What is the significance of calculating the zero rate from discount factor?
Answer: Calculating the zero rate helps determine the risk-free rate used to discount future cash flows, enabling accurate valuation of bonds and assessment of their yields.
Question 2: How does the discount factor relate to the zero rate?
Answer: The discount factor is derived from the zero rate and is used to convert future cash flows to their present value. A higher zero rate results in a higher discount factor, leading to a lower present value.
Question 3: What factors influence the zero rate?
Answer: The zero rate is primarily influenced by economic growth, inflation expectations, and central bank policy decisions.
Question 4: How can I apply the zero rate in investment decisions?
Answer: Understanding the zero rate allows investors to assess the risk and return profile of bond investments, make informed decisions on bond selection, and implement interest rate hedging strategies.
Question 5: Are there any limitations to using the zero rate?
Answer: While the zero rate provides a useful benchmark, it assumes a constant risk-free rate, which may not always hold true in real-world scenarios.
Question 6: What are some practical examples of how the zero rate is used?
Answer: The zero rate is used in various financial applications, such as bond pricing, yield curve construction, and risk management.
Summary of key takeaways or final thought
These frequently asked questions provide a deeper understanding of the calculation of zero rate from discount factor and its practical applications. By considering these factors, investors can make informed investment decisions and navigate the financial markets more effectively.
Transition to the next article section
In the next section, we will delve into advanced techniques for calculating the zero rate from discount factor and explore its implications for bond valuation and risk management.
Tips for Calculating Zero Rate from Discount Factor
This section provides practical tips to enhance your understanding and accuracy when calculating zero rate from discount factor, empowering you to make informed investment decisions.
Tip 1: Utilize reputable sources: Refer to reliable financial data providers and research platforms to obtain accurate discount factors and other relevant information.
Tip 2: Consider the time value of money: Recognize that the value of money changes over time due to inflation and interest rate fluctuations. Use appropriate discount factors that reflect the time period being considered.
Tip 3: Understand the relationship between discount rate and zero rate: The zero rate is derived from the discount rate used to discount future cash flows. A higher discount rate leads to a higher zero rate.
Tip 4: Monitor economic indicators: Keep track of economic data such as inflation, GDP growth, and central bank policy announcements, as these factors can influence the zero rate.
Tip 5: Use financial calculators or software: Leverage financial tools to simplify the calculation process and minimize errors. Many online calculators and software programs are available for this purpose.
Tip 6: Seek professional guidance: If needed, consult with financial professionals such as investment advisors or portfolio managers for assistance with complex calculations or investment decisions.
Following these tips can help you accurately calculate the zero rate from discount factor, enabling you to make well-informed investment decisions and navigate the financial markets with confidence.
In the next section, we will explore advanced techniques for calculating the zero rate from discount factor and discuss its implications for portfolio management and risk assessment.
Conclusion
In this article, we have explored the intricate topic of calculating zero rate from discount factor, providing a comprehensive guide to this fundamental financial concept. By understanding the principles and applications of zero rate calculation, investors can gain valuable insights into bond valuation, risk management, and informed investment decision-making.
Key takeaways from this exploration include the significance of the zero rate in determining the risk-free rate, the relationship between discount factor and zero rate, and the practical applications of this concept in investment decisions. Monitoring economic indicators, utilizing reputable sources, and leveraging financial tools can enhance the accuracy and effectiveness of zero rate calculations.