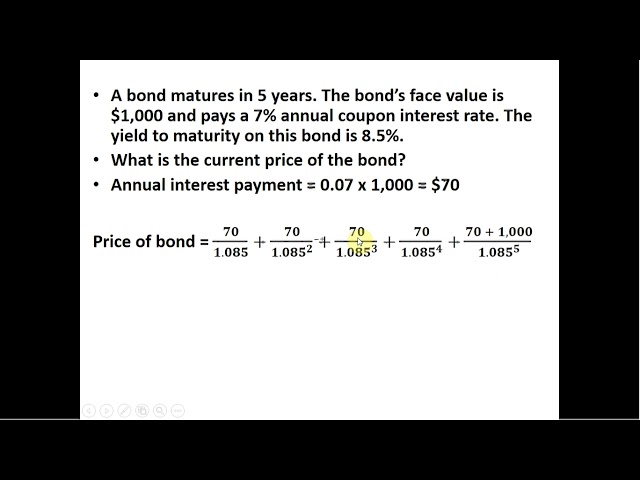
“How to calculate value of coupon payment” pertains to the process of determining the present value of periodic interest payments made on a bond or other fixed-income security. For instance, if a bond has a face value of $1,000 and pays an annual coupon of 5%, the coupon payment would be $50 per year.
Calculating the value of coupon payments is significant for investors as it allows them to assess the worth of their investments and make informed decisions. Understanding this concept empowers investors to evaluate the potential return on investment and compare different fixed-income securities.
Historically, the development of financial calculators and spreadsheet software has simplified the process of calculating coupon payments. This has made it more accessible for individuals to evaluate and manage their investments, leading to increased participation in fixed-income markets.
How to Calculate Value of Coupon Payment
Calculating the value of coupon payments is a crucial aspect of fixed-income investing. It enables investors to assess the worth of their investments and make informed decisions. Key aspects to consider include:
- Coupon Rate
- Face Value
- Time to Maturity
- Payment Frequency
- Yield to Maturity
- Present Value
- Accrued Interest
- Callable/Putable Bonds
A thorough understanding of these aspects provides valuable insights into the dynamics of coupon payments. For instance, a bond with a higher coupon rate generally offers a more attractive return in the short term, while a longer time to maturity implies greater interest rate risk. By considering these factors, investors can make informed decisions about their fixed-income investments.
Coupon Rate
The coupon rate is a crucial factor in determining the value of coupon payments. It represents the annual interest rate paid on a bond, expressed as a percentage of the bond’s face value. A higher coupon rate generally indicates a more attractive return in the short term, while a lower coupon rate may imply a higher potential for capital appreciation over time.
- Nominal Rate: The stated annual interest rate paid on the bond, as specified in the bond indenture.
- Effective Rate: The actual annual interest rate earned by the bondholder, taking into account the compounding frequency and the bond’s price.
- Yield to Maturity (YTM): The internal rate of return on a bond, assuming it is held until maturity. The YTM considers both the coupon rate and the bond’s price.
- Zero-Coupon Bond: A bond that does not pay periodic interest payments, but instead is sold at a discount to its face value. The return on a zero-coupon bond is realized when the bond matures and is redeemed at its face value.
Understanding the nuances of coupon rates is essential for investors to make informed decisions about fixed-income investments. The coupon rate, in conjunction with other factors such as time to maturity and credit risk, influences the overall value and return potential of a bond.
Face Value
Face value, also known as par value, is the nominal value of a bond. It represents the amount that the issuer of the bond promises to repay to the bondholder at maturity. The face value is a critical component of how to calculate value of coupon payment because it serves as the base amount on which the coupon payment is determined.
The coupon payment is a periodic interest payment made to bondholders. It is typically expressed as a percentage of the face value. For example, a bond with a face value of $1,000 and a 5% coupon rate would pay an annual coupon payment of $50. The coupon payment is calculated by multiplying the face value by the coupon rate.
Understanding the relationship between face value and coupon payment is essential for investors to accurately assess the value of bonds and make informed investment decisions. By considering the face value and coupon rate together, investors can determine the total amount of interest they will receive over the life of the bond. This information can be used to compare different bonds and select those that offer the most attractive combination of return and risk.
Time to Maturity
Time to maturity is the period until a bond reaches its maturity date, when the issuer repays the principal amount to the bondholder. It plays a critical role in calculating coupon payments because it determines the number of payments the bondholder will receive over the bond’s life. The relationship between time to maturity and coupon payments is directly proportional, meaning that longer-term bonds generally have higher coupon rates compared to shorter-term bonds with similar credit risk.
To illustrate this relationship, consider two bonds with a $1,000 face value and a 5% coupon rate. Bond A has a time to maturity of 5 years, while Bond B has a time to maturity of 10 years. Using the formula for calculating coupon payments (Coupon Payment = Face Value x Coupon Rate), we find that Bond A will pay $50 in coupon payments each year for 5 years, totaling $250 in coupon payments. Bond B, on the other hand, will pay $50 in coupon payments each year for 10 years, totaling $500 in coupon payments.
Understanding the connection between time to maturity and coupon payments is crucial for investors to make informed decisions. By considering the time to maturity in conjunction with other factors such as the coupon rate and credit risk, investors can assess the overall value and risk profile of a bond and determine whether it aligns with their investment goals and risk tolerance.
Payment Frequency
Payment frequency is a crucial consideration in calculating coupon payments. It refers to the number of times per year that interest payments are made to bondholders. The frequency of payments impacts the overall yield and value of a bond, and it can vary from annual to semi-annual, quarterly, or even monthly.
- Annual: Bonds that pay interest once a year offer a lower yield compared to those with more frequent payments, but they may be preferred by investors seeking simplicity and stability.
- Semi-Annual: Semi-annual payments provide a balance between yield and convenience, with two interest payments received each year.
- Quarterly: Bonds with quarterly payments offer a higher yield and more frequent cash flow, making them attractive to investors seeking regular income.
- Monthly: Monthly payments provide the highest yield and most frequent cash flow, but they may be subject to higher fees and administrative costs.
The payment frequency of a bond should be carefully considered in relation to the investor’s financial goals and risk tolerance. By understanding the impact of payment frequency on coupon payments and overall yield, investors can make informed decisions and select bonds that align with their specific investment objectives.
Yield to Maturity
Yield to Maturity (YTM) is a crucial concept in fixed-income investing and plays a significant role in calculating the value of coupon payments. It represents the internal rate of return (IRR) on a bond, assuming it is held until maturity. The YTM considers both the coupon payments and the bond’s price.
The relationship between YTM and the value of coupon payments is inverse. A higher YTM indicates a lower bond price, and vice versa. This is because investors are willing to pay a lower price for a bond with a higher YTM, as they will receive a higher return on their investment. Conversely, a lower YTM implies a higher bond price, as investors are willing to pay more for a bond that offers a lower return.
Understanding the connection between YTM and coupon payments is essential for investors to accurately calculate the value of bonds and make informed investment decisions. By considering the YTM in conjunction with other factors such as the coupon rate and time to maturity, investors can determine the overall value and return potential of a bond.
In practice, investors use financial calculators or spreadsheet software to calculate the YTM of a bond. This information can be used to compare different bonds and select those that offer the most attractive combination of return and risk.
Present Value
Present Value (PV) is a fundamental concept in finance that plays a critical role in calculating the value of coupon payments. It refers to the current worth of a future sum of money, discounted at a specified interest rate. In the context of fixed-income securities, PV is used to determine the fair value of a bond, considering the present value of all future coupon payments and the final repayment of the principal at maturity.
The relationship between PV and how to calculate the value of coupon payment is direct and essential. The value of coupon payments is calculated by multiplying the face value of the bond by the coupon rate and then discounting the result back to the present using the appropriate interest rate. This process is known as “present value of annuity” calculation. By discounting future coupon payments to their present value, investors can determine the current worth of the bond’s income stream.
Real-life examples of PV within how to calculate value of coupon payment are prevalent in the bond market. When an investor purchases a bond, they are essentially paying the present value of the bond’s future cash flows. This includes the present value of all future coupon payments and the present value of the final principal repayment. By understanding the concept of PV, investors can accurately assess the value of a bond and make informed investment decisions.
Accrued Interest
Accrued interest refers to the interest that has accumulated on a bond since the last coupon payment date but has not yet been paid to the bondholder. It is an important component of how to calculate the value of coupon payment, as it represents the portion of the coupon payment that has been earned but not yet received.
The relationship between accrued interest and the value of coupon payment is direct and essential. When an investor purchases a bond between coupon payment dates, they are entitled to receive the accrued interest in addition to the regular coupon payment on the next payment date. This is because accrued interest is considered part of the total return on the bond investment.
Real-life examples of accrued interest within how to calculate value of coupon payment are prevalent in the bond market. When an investor purchases a bond at a premium (i.e., above its face value), the accrued interest is included in the purchase price. Conversely, when a bond is purchased at a discount (i.e., below its face value), the accrued interest is deducted from the purchase price.
Understanding the concept of accrued interest is crucial for investors to accurately calculate the value of a bond and make informed investment decisions. By considering accrued interest in their calculations, investors can ensure that they are receiving the full value of their investment, including both the regular coupon payments and the accumulated interest since the last payment date.
Callable/Putable Bonds
Callable and putable bonds introduce additional dimensions to the calculation of coupon payments. Callable bonds grant the issuer the option to redeem the bond before its maturity date, typically at a predetermined price. Putable bonds, on the other hand, provide the bondholder with the option to sell the bond back to the issuer before maturity, again at a specified price.
- Call Option: The issuer’s right to redeem the bond before maturity, potentially affecting future coupon payments and the overall value of the bond.
- Put Option: The bondholder’s right to sell the bond back to the issuer before maturity, providing flexibility and potential risk management.
- Call/Put Price: The predetermined price at which the issuer or bondholder can exercise their respective options, influencing the calculation of the bond’s value and coupon payments.
- Impact on Coupon Payments: Callable bonds may lead to early termination of coupon payments, while putable bonds provide an alternative exit strategy, potentially impacting the investor’s projected income stream.
The presence of call or put options adds complexity to the calculation of coupon payments, as it introduces additional factors that can affect the timing and amount of future cash flows. Investors need to carefully consider the terms and conditions of callable/putable bonds, including the call/put prices and dates, to accurately assess their value and potential impact on their investment returns.
Frequently Asked Questions about Coupon Payment Calculation
This FAQ section aims to address common queries and clarify crucial aspects related to calculating the value of coupon payments, providing valuable insights for investors.
Question 1: What is the formula for calculating coupon payments?
Answer: Coupon Payment = Face Value Coupon Rate
Question 5: How does call or put options affect coupon payments?
Answer: Callable bonds may lead to early termination of coupon payments, while putable bonds provide an alternative exit strategy, potentially impacting the investor’s projected income stream.
Question 6: Why is it important to consider accrued interest in calculating coupon payments?
Answer: Accrued interest represents the earned but unpaid portion of the coupon payment since the last payment date. Including accrued interest ensures that investors receive the complete value of their investment, including both regular coupon payments and accumulated interest.
Summary: Understanding the various factors and nuances involved in calculating coupon payments is crucial for investors to make informed decisions and assess the value of fixed-income securities. Accurately calculating coupon payments helps investors determine the income they can expect and evaluate the overall return potential of their investments.
The next section will delve deeper into advanced concepts and strategies related to coupon payment calculation, providing investors with a comprehensive understanding to navigate the fixed-income market effectively.
Tips for Calculating Coupon Payments
Accurately calculating coupon payments is key to making informed investment decisions in fixed-income markets. Here are some valuable tips to help you master this process:
Tip 1: Understand the Key Variables: Begin by grasping the essential variables involved in calculating coupon payments, such as face value, coupon rate, time to maturity, and payment frequency.
Tip 2: Apply the Formula: Use the formula “Coupon Payment = Face Value x Coupon Rate” to determine the periodic interest payments you will receive.
Tip 3: Consider Payment Frequency: Factor in the payment frequency (annual, semi-annual, quarterly, etc.) to calculate the total number of payments over the bond’s lifetime.
Tip 4: Account for Accrued Interest: Remember to include accrued interest when purchasing a bond between coupon payment dates to ensure you receive the full value of your investment.
Tip 5: Understand Callable/Putable Bonds: Be aware of callable or putable bond features, as they may affect the timing and amount of coupon payments.
Tip 6: Use Financial Calculators or Spreadsheets: Leverage financial calculators or spreadsheet software to simplify and expedite the calculation process.
By following these tips, you can enhance your ability to accurately calculate coupon payments, empowering you to make well-informed decisions and maximize your returns in the fixed-income market.
The concluding section of this article will provide further insights into advanced strategies for evaluating and selecting fixed-income securities, building on the foundation established by these tips.
Conclusion
This comprehensive exploration of “how to calculate value of coupon payment” has illuminated key concepts and provided valuable insights for navigating the fixed-income market. Understanding the interplay between face value, coupon rate, time to maturity, payment frequency, accrued interest, and callable/putable bonds empowers investors to make informed decisions.
Accurately calculating coupon payments is not merely a technical exercise; it forms the foundation for evaluating and selecting fixed-income securities. By considering the factors outlined in this article and employing the tips provided, investors can determine the potential income stream and overall return on their investments. This knowledge is essential for portfolio diversification, risk management, and achieving long-term financial goals.