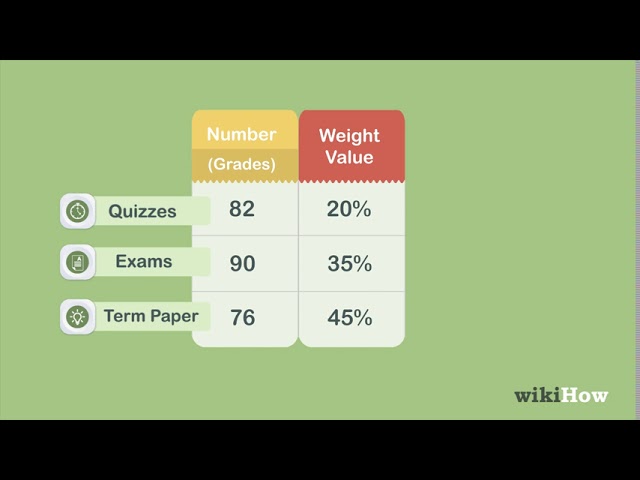
A weighted average coupon is a calculation that determines the average interest rate paid on a bond over its life. Multiply the coupon rate with its proportionate years to maturity; do this for each individual coupon, then divide the total by the total years to maturity. For instance, a bond with a $100 face value, a 5% coupon rate, and a 10-year maturity would have a weighted average coupon of 5%.
Understanding the weighted average coupon is crucial for bond investors as it provides valuable insights into the overall return and risk associated with a bond investment. Its significance lies in providing a more accurate representation of the actual return investors can expect over the bond’s lifetime, considering the time value of money.
The concept of the weighted average coupon emerged as a crucial development in bond valuation. It evolved from the simple practice of averaging coupon rates to a more sophisticated method that takes into account the timing of coupon payments and their impact on the bond’s value.
Weighted Average Coupon
The weighted average coupon is a crucial metric for bond investors, providing insights into the overall return and risk associated with a bond investment. Understanding its key aspects is essential for accurate bond valuation and informed investment decisions.
- Definition
- Importance
- Formula
- Time Value of Money
- Bond Maturity
- Coupon Frequency
- Bond Price
- Yield to Maturity
- Interest Rate Risk
- Historical Context
These aspects collectively provide a comprehensive understanding of the weighted average coupon and its significance in bond valuation. For instance, considering the time value of money helps investors recognize the impact of coupon payments received at different points in time on the overall return. Furthermore, understanding the relationship between the weighted average coupon and yield to maturity allows investors to assess the potential risk and return trade-offs associated with different bonds.
Definition
The definition of the weighted average coupon is the foundation for understanding its calculation and significance in bond valuation. It provides a clear understanding of the concept and its components.
- Concept
The weighted average coupon represents the average interest rate paid on a bond over its lifetime, considering the time value of money and the timing of coupon payments.
- Calculation
It is calculated by multiplying each coupon rate with its proportionate years to maturity and then dividing the sum by the total years to maturity.
- Components
The key components include coupon rate, time to maturity, and frequency of coupon payments.
- Significance
Understanding the weighted average coupon helps investors assess the overall return and risk associated with a bond investment.
In essence, the definition of the weighted average coupon provides the groundwork for comprehending its role in bond valuation. It encompasses the concept, calculation, key components, and significance, enabling investors to make informed investment decisions.
Importance
Understanding the importance of the weighted average coupon is crucial for bond investors. It provides valuable insights into the overall return and risk associated with a bond investment, enabling informed decision-making.
- Accurate Return Assessment
The weighted average coupon considers the time value of money and the timing of coupon payments, providing a more accurate representation of the actual return investors can expect over the bond’s lifetime.
- Risk Evaluation
It helps investors assess the interest rate risk associated with a bond. Bonds with a higher weighted average coupon are generally less sensitive to interest rate changes, making them more stable investments.
- Bond Comparison
The weighted average coupon allows investors to compare different bonds on an equal footing, even if they have different coupon rates and maturity dates.
- Portfolio Management
It assists investors in constructing a bond portfolio that aligns with their risk tolerance and return objectives.
In summary, the importance of the weighted average coupon lies in its ability to provide investors with a comprehensive understanding of a bond’s return and risk characteristics, enabling them to make informed investment decisions and optimize their bond portfolio.
Formula
The formula for calculating the weighted average coupon is a crucial aspect of understanding how to calculate the weighted average coupon. It provides a step-by-step approach to determine the average interest rate paid on a bond over its lifetime, considering the time value of money and the timing of coupon payments.
- Components
The formula involves multiplying each coupon rate with its proportionate years to maturity and then dividing the sum by the total years to maturity. The components include coupon rate, years to maturity, and number of coupon payments per year.
- Example
For a bond with a $100 face value, a 5% coupon rate, and a 10-year maturity, the weighted average coupon would be calculated as follows: WAC = (5% x 10 years) / 10 years = 5%.
- Time Value of Money
The formula considers the time value of money by taking into account the present value of future coupon payments. This ensures that the weighted average coupon reflects the actual return investors can expect to receive over the bond’s lifetime.
- Implications
The weighted average coupon has implications for bond pricing and yield calculation. A higher weighted average coupon generally leads to a higher bond price and a lower yield to maturity.
In summary, the formula for calculating the weighted average coupon provides a systematic approach to determining the average interest rate paid on a bond over its lifetime, considering the time value of money and the timing of coupon payments. This formula is essential for accurate bond valuation and informed investment decisions.
Time Value of Money
The time value of money (TVM) is a fundamental concept in finance that recognizes the different values of money at different points in time. It is a crucial consideration in calculating the weighted average coupon (WAC) of a bond, as it takes into account the present value of future coupon payments.
- Present Value
The present value of a future sum of money is the current value of that future amount, discounted at a given interest rate. In calculating the WAC, the present value of each future coupon payment is determined and used in the calculation.
- Discount Rate
The discount rate used in the TVM calculation is typically the yield to maturity (YTM) of the bond. The YTM represents the internal rate of return (IRR) of the bond, and it is used to discount future coupon payments back to their present value.
- Compounding
Compounding refers to the process of earning interest on interest. In the context of the WAC, compounding considers the effect of reinvesting coupon payments at the prevailing interest rate.
- Annuity
An annuity is a series of equal payments made at regular intervals. The WAC calculation treats the stream of future coupon payments as an annuity, and it uses the annuity formula to determine the present value of these payments.
By incorporating the time value of money into the WAC calculation, investors can accurately assess the present value of future coupon payments and determine the overall return and risk characteristics of a bond investment.
Bond Maturity
Bond maturity is a crucial aspect of calculating the weighted average coupon (WAC) of a bond, as it determines the time period over which coupon payments will be received. Understanding the different facets of bond maturity is essential for accurate WAC calculation and informed investment decisions.
- Maturity Date
The maturity date is the date on which the bond’s principal amount becomes due and payable to the bondholder. It represents the end of the bond’s life, and all remaining coupon payments are made before this date.
- Term to Maturity
The term to maturity refers to the number of years from the present date until the maturity date. It is a key factor in determining the present value of future coupon payments and the overall return on a bond investment.
- Callable Bonds
Callable bonds give the issuer the option to redeem the bonds before the maturity date. This feature affects the WAC calculation, as the issuer may call the bonds if interest rates decline, potentially reducing the number of future coupon payments.
- Putable Bonds
Putable bonds grant the bondholder the right to sell the bond back to the issuer before the maturity date. This optionality can impact the WAC calculation, especially if interest rates rise, as bondholders may exercise their put option.
By considering these facets of bond maturity, investors can accurately calculate the WAC of a bond, which is essential for assessing the overall return and risk characteristics of a bond investment. The maturity date, term to maturity, and the presence of call or put options all influence the timing and amount of future coupon payments, which ultimately affect the WAC and the attractiveness of the bond to potential investors.
Coupon Frequency
Coupon frequency plays a crucial role in calculating the weighted average coupon (WAC) of a bond. It refers to the number of times per year that a bond pays interest to its holders. Understanding the different aspects of coupon frequency is essential for accurate WAC calculation and informed investment decisions.
- Frequency Options
Bonds typically have coupon frequencies of annual, semi-annual, quarterly, or monthly payments. The more frequent the coupon payments, the more frequently investors receive interest income.
- Impact on WAC
Coupon frequency affects the WAC by influencing the timing of coupon payments. Bonds with more frequent coupon payments have a higher WAC because the present value of future coupon payments is higher.
- Investor Preferences
Some investors prefer bonds with frequent coupon payments for regular income, while others may prefer less frequent payments for potential capital appreciation.
- Tax Implications
In some cases, the frequency of coupon payments can affect the tax treatment of bond income. Investors should consider tax implications when choosing bonds with different coupon frequencies.
By factoring in coupon frequency, investors can accurately calculate the WAC of a bond and make informed decisions about which bonds to include in their portfolios. Coupon frequency influences the timing and amount of interest payments, which ultimately affects the overall return and risk profile of a bond investment.
Bond Price
The bond price and weighted average coupon (WAC) are intricately connected in the world of fixed-income investments. The bond price is the current market value of a bond, and it is influenced by several factors, including the WAC. Understanding this relationship is crucial for investors seeking to make informed decisions in bond markets.
WAC plays a direct role in determining the bond price. A higher WAC generally leads to a higher bond price, as investors are willing to pay more for a bond that pays a higher average interest rate over its lifetime. Conversely, a lower WAC typically results in a lower bond price. This relationship stems from the present value concept, where future cash flows (in this case, coupon payments) are discounted back to their present value to determine the bond’s price.
In real-life scenarios, the bond price and WAC are constantly evolving in response to changes in the market environment. For instance, when interest rates rise, the prices of existing bonds with lower WACs may decline as investors seek higher-yielding bonds. Conversely, when interest rates fall, the prices of bonds with higher WACs may increase as they become more attractive to investors seeking stable income.
Understanding the relationship between bond price and WAC is essential for investors to make informed investment decisions. By considering the WAC alongside other factors such as credit risk and maturity, investors can assess the overall value and potential return of a bond investment.
Yield to Maturity
Yield to maturity (YTM) is a crucial concept in bond valuation, and it shares a fundamental relationship with the weighted average coupon (WAC) in the context of fixed-income investments. YTM represents the annualized rate of return an investor can expect to receive if they hold a bond until its maturity date. WAC, on the other hand, is the average interest rate a bond is expected to pay over its lifetime, taking into account the time value of money.
YTM is a critical component of WAC calculation. When calculating WAC, the present value of each future coupon payment is discounted back to the present using the YTM as the discount rate. This process ensures that the WAC accurately reflects the investor’s expected return over the bond’s lifetime.
In real-life scenarios, YTM and WAC are interdependent. When YTM rises, the WAC of existing bonds generally falls, and vice versa. This relationship is driven by the present value concept: as YTM increases, the present value of future coupon payments decreases, leading to a lower WAC. Conversely, when YTM decreases, the present value of future coupon payments increases, resulting in a higher WAC.
Understanding the connection between YTM and WAC is essential for bond investors to make informed investment decisions. By considering both metrics together, investors can assess the overall value and potential return of a bond investment. This understanding helps investors construct well-diversified bond portfolios that align with their risk tolerance and return objectives.
Interest Rate Risk
Interest rate risk is a critical consideration in the calculation of the weighted average coupon (WAC) of a bond. WAC represents the average interest rate a bond is expected to pay over its lifetime, and it is used to determine the bond’s price and yield. Interest rate risk refers to the potential impact of changes in interest rates on the value of a bond.
When interest rates rise, the prices of existing bonds with lower WACs tend to decline. This is because investors can now purchase new bonds with higher coupons at a lower price, making the older bonds less attractive. Conversely, when interest rates fall, the prices of bonds with higher WACs tend to increase as they offer a more attractive return compared to new bonds with lower coupons.
A real-life example of interest rate risk in the calculation of WAC occurred during the period of rising interest rates in the early 1980s. Many bonds issued in the 1970s had low WACs due to the prevailing low interest rates at that time. As interest rates rose sharply in the early 1980s, the prices of these older bonds plummeted, causing significant losses for investors.
Understanding the relationship between interest rate risk and WAC is crucial for investors to make informed investment decisions. By considering both WAC and interest rate risk, investors can assess the potential impact of changing interest rates on the value of their bond investments. This understanding helps investors manage their portfolios effectively and mitigate the risks associated with interest rate fluctuations.
Historical Context
The historical context surrounding the weighted average coupon (WAC) provides valuable insights into its evolution and significance in bond valuation. Understanding this context enables investors to appreciate the dynamic nature of WAC and its role in shaping bond market practices over time.
- Origins
WAC emerged as a crucial concept in bond valuation during the early 20th century. Prior to this, bond yields were typically calculated using simple averaging methods, which did not consider the time value of money or the varying payment schedules of bonds.
- Development of Yield Curve
The development of the yield curve theory in the 1950s and 1960s further highlighted the importance of WAC. The yield curve depicted the relationship between interest rates and bond maturities, providing a benchmark against which WAC could be compared.
- Technological Advancements
The advent of computers and calculators in the 1970s and 1980s revolutionized the calculation of WAC. These advancements enabled bond professionals to perform complex calculations quickly and accurately, facilitating the widespread adoption of WAC in bond valuation.
- Regulatory Changes
Regulatory changes, such as the introduction of mark-to-market accounting in the 1990s, increased the need for accurate bond valuation. WAC became an essential tool for financial institutions to assess the fair value of their bond portfolios.
In conclusion, the historical context of WAC encompasses its origins, the development of the yield curve, technological advancements, and regulatory changes. Understanding this context provides investors with a deeper appreciation of WAC’s significance and its role in shaping bond market practices over time.
Weighted Average Coupon FAQs
This section addresses frequently asked questions to clarify the concept of weighted average coupon and guide its calculation.
Question 1: What is the significance of weighted average coupon in bond valuation?
Weighted average coupon is crucial as it provides a comprehensive view of the average interest rate an investor can expect over a bond’s lifetime, considering the time value of money and the timing of coupon payments.
Question 2: How is weighted average coupon calculated?
Weighted average coupon is calculated by multiplying each coupon rate with its proportionate years to maturity and then dividing the sum by the total years to maturity.
Question 3: Does a higher weighted average coupon always indicate a better investment?
Not necessarily. While a higher weighted average coupon generally signifies higher interest payments, investors should also consider factors like credit risk, liquidity, and market conditions before making investment decisions.
Question 4: How does interest rate risk affect weighted average coupon?
Interest rate risk refers to the potential impact of interest rate changes on the value of a bond. When interest rates rise, bonds with lower weighted average coupons may experience a decline in value, while bonds with higher weighted average coupons may become more valuable.
Question 5: Why is it important to consider the frequency of coupon payments when calculating weighted average coupon?
Coupon frequency influences the timing of interest payments, which affects the present value of future coupon payments. Bonds with more frequent coupon payments tend to have a higher weighted average coupon due to the higher present value of their cash flows.
Question 6: How is the yield to maturity related to weighted average coupon?
Yield to maturity is a crucial factor in calculating weighted average coupon. It serves as the discount rate used to determine the present value of future coupon payments. Changes in yield to maturity can impact the weighted average coupon of a bond.
These FAQs offer valuable insights into weighted average coupon and its calculation, helping investors make informed decisions in the bond market.
In the next section, we will delve further into the practical applications of weighted average coupon in bond portfolio management and risk assessment.
Weighted Average Coupon
This section provides practical tips to effectively utilize weighted average coupon (WAC) in bond portfolio management and risk assessment.
Tip 1: Consider WAC in Bond Selection: Evaluate WAC alongside other factors when selecting bonds to identify those that offer a favorable combination of yield and risk.
Tip 2: Monitor WAC for Interest Rate Risk: Track WAC to assess the potential impact of interest rate changes on your bond portfolio. Bonds with lower WACs are more sensitive to interest rate increases.
Tip 3: Utilize WAC for Yield Curve Analysis: Compare WACs of bonds with different maturities to identify opportunities arising from the shape of the yield curve.
Tip 4: Calculate WAC for Portfolio Optimization: Determine the overall WAC of your bond portfolio to ensure it aligns with your investment objectives and risk tolerance.
Tip 5: Leverage WAC for Duration Assessment: WAC can provide insights into the duration of your bond portfolio, helping you manage interest rate risk and volatility.
Tip 6: Compare WAC Across Bond Types: Analyze the WACs of different bond types, such as corporate bonds, government bonds, and high-yield bonds, to identify potential diversification opportunities.
Tip 7: Utilize WAC for Bond Ladder Strategy: Implement a bond laddering strategy by investing in bonds with varying WACs and maturities to manage interest rate risk and generate regular income.
Tip 8: Integrate WAC into Risk Management: Incorporate WAC into your risk management framework to identify and mitigate potential risks associated with bond investments, including credit risk and liquidity risk.
By following these tips, investors can harness the power of WAC to make informed decisions, optimize their bond portfolios, and effectively manage risk in the fixed-income market.
In the final section of this article, we will delve into advanced applications of WAC, exploring its role in sophisticated bond investment strategies and portfolio construction.
Conclusion
Our exploration of “Weighted Average Coupon: How to Calculate” has shed light on its significance in bond valuation and risk assessment. The weighted average coupon provides a comprehensive view of a bond’s average interest rate over its lifetime, considering the time value of money and the timing of coupon payments. Understanding its calculation and implications empowers investors to make informed investment decisions.
Key points to remember:
- Weighted average coupon reflects the average interest rate investors can expect to receive from a bond, considering the time value of money and the timing of coupon payments.
- It is a crucial factor in bond valuation, influencing a bond’s price and yield.
- Weighted average coupon helps investors assess interest rate risk and make informed portfolio decisions.
In conclusion, the weighted average coupon is an indispensable tool for bond investors, enabling them to accurately assess the return and risk characteristics of potential investments. By incorporating this concept into their investment strategies, investors can navigate the bond market with greater confidence and make informed decisions that align with their financial goals.